Lecture Notes for 120 - UCLA Department of Mathematics

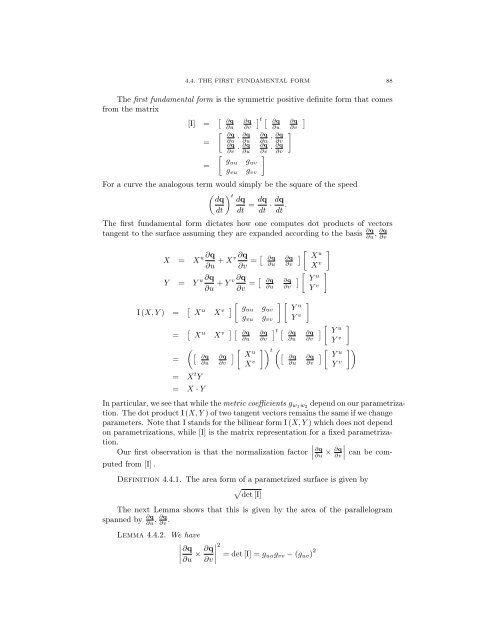
4.4. THE FIRST FUNDAMENTAL FORM 88
The first fundamental form is the symmetric positive definite form that comes
from the matrix
[I] = ⇥ ⇤
@q t ⇥ @q
⇤
=
=
@q
@u @v @u
apple @q
@u · @q @q
@u @u · @q
@v
@q
@v · @q @q
@u @v · @q
@v
apple
guu g uv
g vu g vv
For a curve the analogous term would simply be the square of the speed
✓ ◆ t dq dq
dt dt = dq
dt · dq
dt .
The first fundamental form dictates how one computes dot products of vectors
tangent to the surface assuming they are expanded according to the basis @q
@u , @q
@v
@q
@v
X = X u @q @q
+ Xv
@u @v = ⇥ @q
@u
Y = Y u @q
@u + Y v @q
@v = ⇥ @q
@u
@q
@v
@q
@v
⇤ apple X u
X v
⇤ apple Y u
Y v
I(X, Y ) = ⇥ X u X ⇤ apple apple v g uu g uv Y
u
g vu g vv Y v
= ⇥ X u X v ⇤⇥ @q
@u
=
✓ ⇥ @q
@u
= X t Y
= X · Y
@q
@v
⇤ apple X u
X v
@q
@v
⇤ t ⇥ @q
@u
◆ t ✓ ⇥ @q
@u
@q
@v
@q
@v
⇤ apple Y u
Y v
⇤ apple Y u
In particular, we see that while the metric coefficients g w1w 2
depend on our parametrization.
The dot product I(X, Y ) of two tangent vectors remains the same if we change
parameters. Note that I stands for the bilinear form I(X, Y ) which does not depend
on parametrizations, while [I] is the matrix representation for a fixed parametrization.
Our first observation is that the normalization factor
can be computed
from [I] .
Y v
@q
@u ⇥ @q
@v
Definition 4.4.1. The area form of a parametrized surface is given by
p
det [I]
◆
The next Lemma shows that this is given by the area of the parallelogram
spanned by @q
@u , @q
@v .
Lemma 4.4.2. We have
@q
@u ⇥ @q
@v
2
=det[I]=g uu g vv (g uv ) 2