Lecture Notes for 120 - UCLA Department of Mathematics

B.5. GEODESIC COORDINATES 187
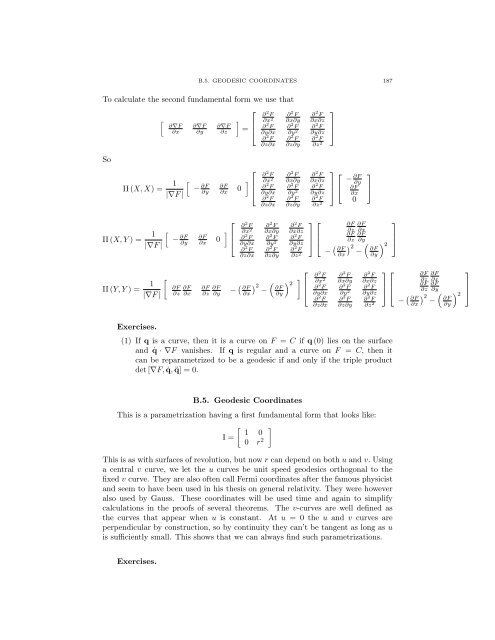
To calculate the second fundamental form we use that
2
@ 2 F
i @x
6
2
@
= 4
2 F
h
@rF
@x
@rF
@y
@rF
@z
@y@x
@ 2 F
@z@x
@ 2 F
@x@y
@ 2 F
@y 2
@ 2 F
@z@y
3
@ 2 F
@x@z
@ 2 F 7
@y@z 5
@ 2 F
@z 2
So
II (X, X) = 1 h
|rF |
@F
@y
@F
@x
0
2
i
6
4
@ 2 F
@x 2
@ 2 F
@y@x
@ 2 F
@z@x
@ 2 F
@x@y
@ 2 F
@y 2
@ 2 F
@z@y
3
@ 2 F
@x@z
@ 2 F 7
@y@z 5
@ 2 F
@z 2
2
4
@y
@F
@x
0
5
@F
3
II (X, Y )= 1 h
|rF |
@F
@y
@F
@x
0
2
i
6
4
@ 2 F
@x 2
@ 2 F
@y@x
@ 2 F
@z@x
@ 2 F
@x@y
@ 2 F
@y 2
@ 2 F
@z@y
3
@ 2 F
@x@z
@ 2 F 7
@y@z 5
@ 2 F
@z 2
2
6
4
@F @F
@z @x
@F @F
@z @y
@F 2
@x
⇣
@F
@y
3
7
⌘ 2
5
II (Y,Y )= 1 apple
|rF |
@F @F
@z @x
@F @F
@z @y
@F
@x
2
⇣
@F
@y
⌘ 2
2
6
4
@ 2 F
@x 2
@ 2 F
@y@x
@ 2 F
@z@x
@ 2 F
@x@y
@ 2 F
@y 2
@ 2 F
@z@y
3
@ 2 F
@x@z
@ 2 F 7
@y@z 5
@ 2 F
@z 2
2
6
4
@F @F
@z @x
@F @F
@z @y
@F 2
@x
⇣
@F
@y
3
7
⌘ 2
5
Exercises.
(1) If q is a curve, then it is a curve on F = C if q (0) lies on the surface
and ˙q ·rF vanishes. If q is regular and a curve on F = C, then it
can be reparametrized to be a geodesic if and only if the triple product
det [rF, ˙q, ¨q] =0.
B.5. Geodesic Coordinates
This is a parametrization having a first fundamental form that looks like:
apple 1 0
I=
0 r 2
This is as with surfaces of revolution, but now r can depend on both u and v. Using
acentralv curve, we let the u curves be unit speed geodesics orthogonal to the
fixed v curve. They are also often call Fermi coordinates after the famous physicist
and seem to have been used in his thesis on general relativity. They were however
also used by Gauss. These coordinates will be used time and again to simplify
calculations in the proofs of several theorems. The v-curves are well defined as
the curves that appear when u is constant. At u = 0 the u and v curves are
perpendicular by construction, so by continuity they can’t be tangent as long as u
is sufficiently small. This shows that we can always find such parametrizations.
Exercises.