enumeration of the number of spanning trees in some ... - Toubkal

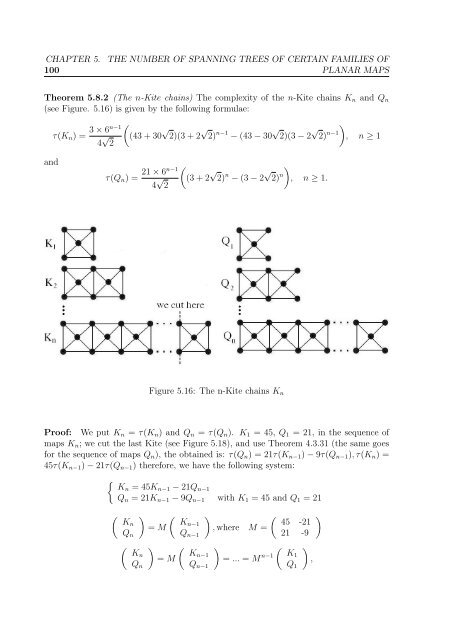
CHAPTER 5.100THE NUMBER OF SPANNING TREES OF CERTAIN FAMILIES OFPLANAR MAPSTheorem 5.8.2 (The n-Kite chains) The complexity of the n-Kite chains K n and Q n(see Figure. 5.16) is given by the following formulae:τ(K n ) = 3 × ((43 6n−14 √ + 30 √ 2)(3 + 2 √ 2) n−1 − (43 − 30 √ 2)(3 − 2 √ )2) n−1 , n ≥ 12andτ(Q n ) =21 × 6n−14 √ 2((3 + 2 √ 2) n − (3 − 2 √ 2) n ), n ≥ 1.Figure 5.16: The n-Kite chains K nProof: We put K n = τ(K n ) and Q n = τ(Q n ). K 1 = 45, Q 1 = 21, in the sequence ofmaps K n ; we cut the last Kite (see Figure 5.18), and use Theorem 4.3.31 (the same goesfor the sequence of maps Q n ), the obtained is: τ(Q n ) = 21τ(K n−1 ) − 9τ(Q n−1 ), τ(K n ) =45τ(K n−1 ) − 21τ(Q n−1 ) therefore, we have the following system:{Kn = 45K n−1 − 21Q n−1Q n = 21K n−1 − 9Q n−1 with K 1 = 45 and Q 1 = 21(Kn)= MQ n(Kn)= MQ n(Kn−1Q n−1), where M =( 45 -2121 -9(Kn−1Q n−1)= ... = M n−1 (K1Q 1),)