enumeration of the number of spanning trees in some ... - Toubkal

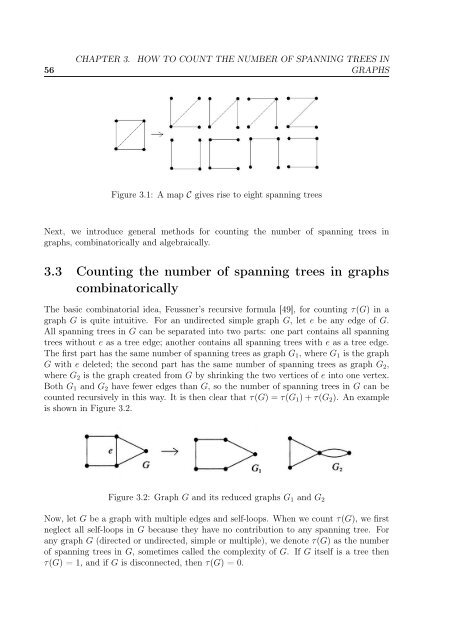
56CHAPTER 3.HOW TO COUNT THE NUMBER OF SPANNING TREES INGRAPHSFigure 3.1: A map C gives rise to eight spanning treesNext, we introduce general methods for counting the number of spanning trees ingraphs, combinatorically and algebraically.3.3 Counting the number of spanning trees in graphscombinatoricallyThe basic combinatorial idea, Feussner’s recursive formula [49], for counting τ(G) in agraph G is quite intuitive. For an undirected simple graph G, let e be any edge of G.All spanning trees in G can be separated into two parts: one part contains all spanningtrees without e as a tree edge; another contains all spanning trees with e as a tree edge.The first part has the same number of spanning trees as graph G 1 , where G 1 is the graphG with e deleted; the second part has the same number of spanning trees as graph G 2 ,where G 2 is the graph created from G by shrinking the two vertices of e into one vertex.Both G 1 and G 2 have fewer edges than G, so the number of spanning trees in G can becounted recursively in this way. It is then clear that τ(G) = τ(G 1 ) + τ(G 2 ). An exampleis shown in Figure 3.2.Figure 3.2: Graph G and its reduced graphs G 1 and G 2Now, let G be a graph with multiple edges and self-loops. When we count τ(G), we firstneglect all self-loops in G because they have no contribution to any spanning tree. Forany graph G (directed or undirected, simple or multiple), we denote τ(G) as the numberof spanning trees in G, sometimes called the complexity of G. If G itself is a tree thenτ(G) = 1, and if G is disconnected, then τ(G) = 0.