enumeration of the number of spanning trees in some ... - Toubkal

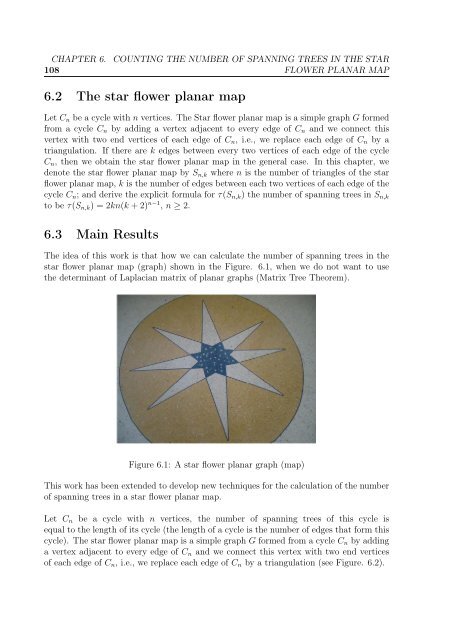
CHAPTER 6.108COUNTING THE NUMBER OF SPANNING TREES IN THE STARFLOWER PLANAR MAP6.2 The star flower planar mapLet C n be a cycle with n vertices. The Star flower planar map is a simple graph G formedfrom a cycle C n by adding a vertex adjacent to every edge of C n and we connect thisvertex with two end vertices of each edge of C n , i.e., we replace each edge of C n by atriangulation. If there are k edges between every two vertices of each edge of the cycleC n , then we obtain the star flower planar map in the general case. In this chapter, wedenote the star flower planar map by S n,k where n is the number of triangles of the starflower planar map, k is the number of edges between each two vertices of each edge of thecycle C n ; and derive the explicit formula for τ(S n,k ) the number of spanning trees in S n,kto be τ(S n,k ) = 2kn(k + 2) n−1 , n ≥ 2.6.3 Main ResultsThe idea of this work is that how we can calculate the number of spanning trees in thestar flower planar map (graph) shown in the Figure. 6.1, when we do not want to usethe determinant of Laplacian matrix of planar graphs (Matrix Tree Theorem).Figure 6.1: A star flower planar graph (map)This work has been extended to develop new techniques for the calculation of the numberof spanning trees in a star flower planar map.Let C n be a cycle with n vertices, the number of spanning trees of this cycle isequal to the length of its cycle (the length of a cycle is the number of edges that form thiscycle). The star flower planar map is a simple graph G formed from a cycle C n by addinga vertex adjacent to every edge of C n and we connect this vertex with two end verticesof each edge of C n , i.e., we replace each edge of C n by a triangulation (see Figure. 6.2).