enumeration of the number of spanning trees in some ... - Toubkal

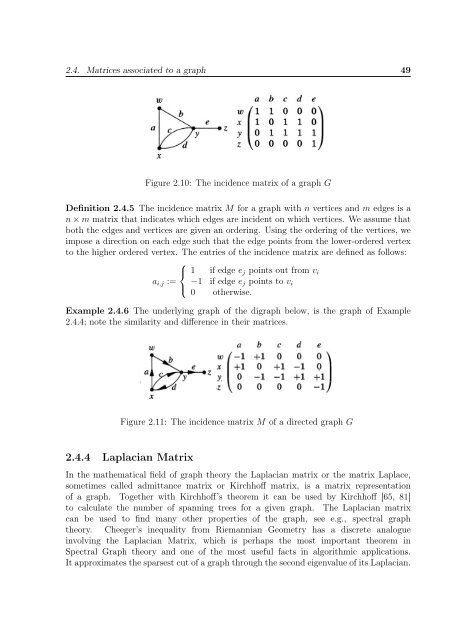
2.4. Matrices associated to a graph 49Figure 2.10: The incidence matrix of a graph GDefinition 2.4.5 The incidence matrix M for a graph with n vertices and m edges is an × m matrix that indicates which edges are incident on which vertices. We assume thatboth the edges and vertices are given an ordering. Using the ordering of the vertices, weimpose a direction on each edge such that the edge points from the lower-ordered vertexto the higher ordered vertex. The entries of the incidence matrix are defined as follows:⎧⎨ 1 if edge e j points out from v ia i,j := −1 if edge e j points to v i⎩0 otherwise.Example 2.4.6 The underlying graph of the digraph below, is the graph of Example2.4.4; note the similarity and difference in their matrices.Figure 2.11: The incidence matrix M of a directed graph G2.4.4 Laplacian MatrixIn the mathematical field of graph theory the Laplacian matrix or the matrix Laplace,sometimes called admittance matrix or Kirchhoff matrix, is a matrix representationof a graph. Together with Kirchhoff’s theorem it can be used by Kirchhoff [65, 81]to calculate the number of spanning trees for a given graph. The Laplacian matrixcan be used to find many other properties of the graph, see e.g., spectral graphtheory. Cheeger’s inequality from Riemannian Geometry has a discrete analogueinvolving the Laplacian Matrix, which is perhaps the most important theorem inSpectral Graph theory and one of the most useful facts in algorithmic applications.It approximates the sparsest cut of a graph through the second eigenvalue of its Laplacian.