enumeration of the number of spanning trees in some ... - Toubkal
enumeration of the number of spanning trees in some ... - Toubkal
enumeration of the number of spanning trees in some ... - Toubkal
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
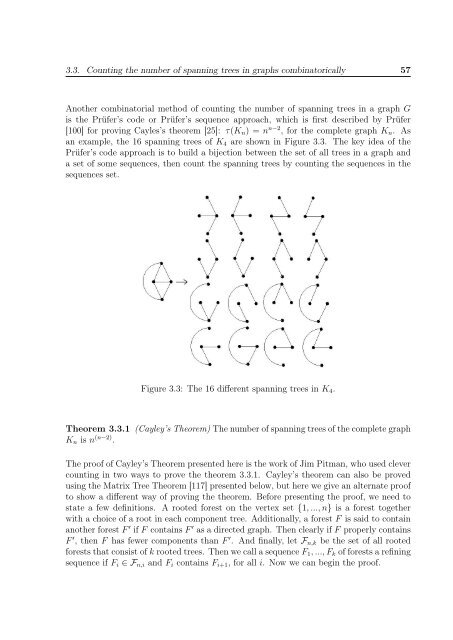
3.3. Count<strong>in</strong>g <strong>the</strong> <strong>number</strong> <strong>of</strong> <strong>spann<strong>in</strong>g</strong> <strong>trees</strong> <strong>in</strong> graphs comb<strong>in</strong>atorically 57Ano<strong>the</strong>r comb<strong>in</strong>atorial method <strong>of</strong> count<strong>in</strong>g <strong>the</strong> <strong>number</strong> <strong>of</strong> <strong>spann<strong>in</strong>g</strong> <strong>trees</strong> <strong>in</strong> a graph Gis <strong>the</strong> Prüfer’s code or Prüfer’s sequence approach, which is first described by Prüfer[100] for prov<strong>in</strong>g Cayles’s <strong>the</strong>orem [25]: τ(K n ) = n n−2 , for <strong>the</strong> complete graph K n . Asan example, <strong>the</strong> 16 <strong>spann<strong>in</strong>g</strong> <strong>trees</strong> <strong>of</strong> K 4 are shown <strong>in</strong> Figure 3.3. The key idea <strong>of</strong> <strong>the</strong>Prüfer’s code approach is to build a bijection between <strong>the</strong> set <strong>of</strong> all <strong>trees</strong> <strong>in</strong> a graph anda set <strong>of</strong> <strong>some</strong> sequences, <strong>the</strong>n count <strong>the</strong> <strong>spann<strong>in</strong>g</strong> <strong>trees</strong> by count<strong>in</strong>g <strong>the</strong> sequences <strong>in</strong> <strong>the</strong>sequences set.Figure 3.3: The 16 different <strong>spann<strong>in</strong>g</strong> <strong>trees</strong> <strong>in</strong> K 4 .Theorem 3.3.1 (Cayley’s Theorem) The <strong>number</strong> <strong>of</strong> <strong>spann<strong>in</strong>g</strong> <strong>trees</strong> <strong>of</strong> <strong>the</strong> complete graphK n is n (n−2) .The pro<strong>of</strong> <strong>of</strong> Cayley’s Theorem presented here is <strong>the</strong> work <strong>of</strong> Jim Pitman, who used clevercount<strong>in</strong>g <strong>in</strong> two ways to prove <strong>the</strong> <strong>the</strong>orem 3.3.1. Cayley’s <strong>the</strong>orem can also be provedus<strong>in</strong>g <strong>the</strong> Matrix Tree Theorem [117] presented below, but here we give an alternate pro<strong>of</strong>to show a different way <strong>of</strong> prov<strong>in</strong>g <strong>the</strong> <strong>the</strong>orem. Before present<strong>in</strong>g <strong>the</strong> pro<strong>of</strong>, we need tostate a few def<strong>in</strong>itions. A rooted forest on <strong>the</strong> vertex set {1, ..., n} is a forest toge<strong>the</strong>rwith a choice <strong>of</strong> a root <strong>in</strong> each component tree. Additionally, a forest F is said to conta<strong>in</strong>ano<strong>the</strong>r forest F ′ if F conta<strong>in</strong>s F ′ as a directed graph. Then clearly if F properly conta<strong>in</strong>sF ′ , <strong>the</strong>n F has fewer components than F ′ . And f<strong>in</strong>ally, let F n,k be <strong>the</strong> set <strong>of</strong> all rootedforests that consist <strong>of</strong> k rooted <strong>trees</strong>. Then we call a sequence F 1 , ..., F k <strong>of</strong> forests a ref<strong>in</strong><strong>in</strong>gsequence if F i ∈ F n,i and F i conta<strong>in</strong>s F i+1 , for all i. Now we can beg<strong>in</strong> <strong>the</strong> pro<strong>of</strong>.