Flujo de fluidos estratificados - PreMAT

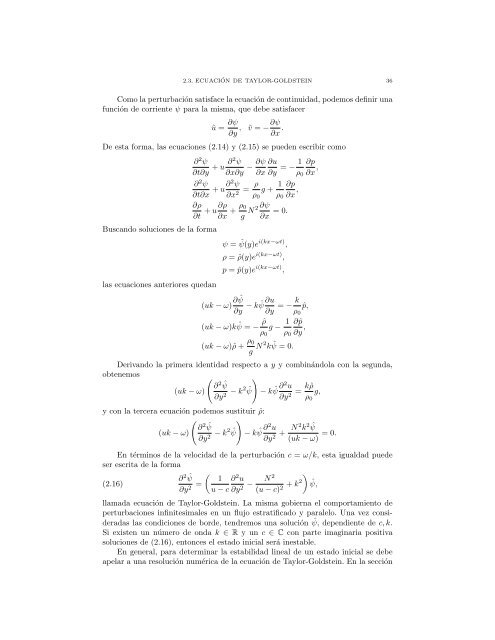
2.3. ECUACIÓN DE TAYLOR-GOLDSTEIN 36
Como la perturbación satisface la ecuación de continuidad, podemos definir una
función de corriente ψ para la misma, que debe satisfacer
ũ = ∂ψ
, ˜v = −∂ψ
∂y ∂x .
De esta forma, las ecuaciones (2.14) y (2.15) se pueden escribir como
∂2ψ ∂t∂y + u ∂2ψ ∂ψ ∂u 1 ∂p
− = −
∂x∂y ∂x ∂y ρ0 ∂x ,
∂2ψ ∂t∂x + u∂2 ψ ρ
= g +
∂x2 1 ∂p
∂x ,
∂ρ ∂ρ
+ u
∂t ∂x
Buscando soluciones de la forma
las ecuaciones anteriores quedan
ρ0
ρ0
ρ0 2 ∂ψ
+ N = 0.
g ∂x
ψ = ˆ ψ(y)e i(kx−ωt) ,
ρ = ˆρ(y)e i(kx−ωt) ,
p = ˆp(y)e i(kx−ωt) ,
(uk − ω) ∂ ˆ ψ
∂y − k ˆ ψ ∂u k
= − ˆp,
∂y ρ0
(uk − ω)k ˆ ψ = − ˆρ
g − 1 ∂ ˆp
∂y ,
ρ0
ρ0
(uk − ω)ˆρ + ρ0
g N 2 k ˆ ψ = 0.
Derivando la primera identidad respecto a y y combinándola con la segunda,
obtenemos
∂
(uk − ω)
2 ˆ ψ
∂y2 − k2
ψˆ
− k ˆ ψ ∂2u kˆρ
= g,
∂y2 y con la tercera ecuación podemos sustituir ˆρ:
∂
(uk − ω)
2 ˆ ψ
∂y2 − k2
ψˆ
− k ˆ ψ ∂2u ∂y2 + N 2k2 ˆ ψ
= 0.
(uk − ω)
En términos de la velocidad de la perturbación c = ω/k, esta igualdad puede
ser escrita de la forma
∂
(2.16)
2 ˆ
ψ 1 ∂
=
∂y2 u − c
2
2 u N
− + k2 ˆψ,
∂y2 (u − c) 2
llamada ecuación de Taylor-Goldstein. La misma gobierna el comportamiento de
perturbaciones infinitesimales en un flujo estratificado y paralelo. Una vez consideradas
las condiciones de borde, tendremos una solución ˆ ψ, dependiente de c, k.
Si existen un número de onda k ∈ R y un c ∈ C con parte imaginaria positiva
soluciones de (2.16), entonces el estado inicial será inestable.
En general, para determinar la estabilidad lineal de un estado inicial se debe
apelar a una resolución numérica de la ecuación de Taylor-Goldstein. En la sección
ρ0