Conference, Proceedings

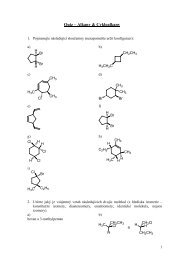
1a. Three‐dimensional temperature field inside a block
The border conditions mentioned above are
( 1 = 2, 2, 3, ) = ( 1 =− 2, 2, 3, ) = ( 2 = 2 2, 1, 3,
) =
( ) ( ) ( )
T x l x x t T x l x x t T x l x x t
T x2 =− l2 2, x1, x3, t = T x3 = l3 2, x1, x2, t = T x3 =− l3 2, x1, x2, t = Ts,
t > 0 (1.1)
and the initial condition is
( )
T x1, x2, x3, t = 0 = T0 , -li 2 < xi < li
2
,
( 1, 2, 3,
)
Solution
T x x x t of 3D heat conduction equation
∂T 2 2
−a ∇ T =
i = 1, 2, 3
, t > 0 (1.2)
0
∂ t
(1.3)
by the method of variable separation assuming it obeys boundary (1.1), and initial (1.2)
conditions is given as [1]
( )
T x1, x2, x3, t −Ts
=
T −T
0
s
(
n1+ n2+ n3+
)
( 2 −1)( 2 −1)( 2 −1)
( n ) ( n ) ( n )
3
3 2 2 2
∞
⎛ 4 ⎞
−1 ⎡ ⎛
2 2 2 1−1 2 2 −1 2 3−1
⎞ ⎤
= ⎜ ⎟ ∑
exp ⎢− π a ⎜ + + ⎟t⎥×
2 2 2
⎝π⎠ n1, n2, n3=
1 n1 n2 n3 ⎢ ⎜ l1 l2 l ⎟
⎝ 3
⎣ ⎠ ⎥
⎦
x x
cos 2 1 cos 2 1 cos 2 1
1 2
3
( n − ) π ( n − ) π ( n − ) π
1 2 3
l1 l2 l3
Threefold infinite sum contains terms (in the second line behind the sum) which with increase
n
of trinity natural numbers 1, n2, n3as well as with increase of the time t decrease. Space
distribution of temperature inside the block at the instant of time t is determined by the product
x, 1,2,3
of three cosine functions. This product represents even function of space variables i i =
.
We see that each term in infinite sum exponentially decreases with characteristic time equal to
τ
i
i = ⎨ ⎬
aπ( 2ni−1) 2
⎧⎪ l ⎫⎪
1
⎛ li
⎞
τ =
⎪⎩ ⎪⎭
2n1 and amplitude decrease is proportional to i −
⎜ ⎟
. The time ⎝2a⎠ is called the relaxation time. Here 2
a = λ cρ
is the diffusivity, λ ‐thermal conductivity and cρ is
the heat capacity density of a body.
Because, the centre of the sample coincides with coordinate origin the time development of
cos( γ i )
temperature at that point is given by the same formula (1.4) in which last three
functions are equal to one.
l
In a case of a cube 1 = l2 = l3 = l
at the center of a cube triple infinite sum can by reduce to one
sum. The time development temperature at that place then is given by the relation
x
2
(1.4)
27