Emmanuel Amiot Modèles algébriques et algorithmes pour la ...

18 autosimilar melodies
at most q ‘alternate melodies’ (under iteration of f). Though in theory one might stumble on the case
f q = id (for instance if the original melody has n distinct notes!), in practice one frequently finds some non
trivial attractor. In this sense, autosimilar melodies are exactly the attractors of any affine map operating
on any initial (periodic) melody, thus reaching the elated status of universal object.
7 Perspectives
7.1 More symmetries
Other generalizations are of course possible. Uncharacteristically, Tom Johnson put forward in [9] a false
conjecture, refuted by Feldman ( [7]), that hints at transformations of the pitch- or time-space more general
than inversion or retrogradation. The conjecture was:
A related melody [= an augmentation of the original melody] produced by playing a melodic loop [= a periodic
melody] at some ratio other than 1:1, can never be the inversion of the original loop, unless it is also a retrograde
of the original loop.
The melodies satisfying this conjecture can be formalized and generalized in the following way:
Definition 7.1 Let G be some finite order transformation of the pitch (classes) domain, and f ∈ Affn.
We define a melody M0, M1, Mn = M0 with period n autosimilar under f, with respect to G, by the
condition ∀k ∈ Zn M f(k) = G(Mk).
We state without proof that this occurs whenever (Mk) is built from the iteration of g on the orbits of
f:
∀x ∈ Zn, ∀k ∈ Z, M f k (x) = G k (Mx).
Also, for this to happen, the order of G must divide all the orbit lengths.
David Feldman, who cast the first shrewd mathematical look on these melodies (he used some himself as a
composer) yet unfortunately only published one page in [7] about them, explains why Johnson’s conjecture
will be true in most cases, and provides a counterexample with period 15. Inversion is G(m) = −m, but
other operators are possible: a reviewer suggested G(m) = m + 3 (mod 12) [on length 4 orbits]. Feldman’s
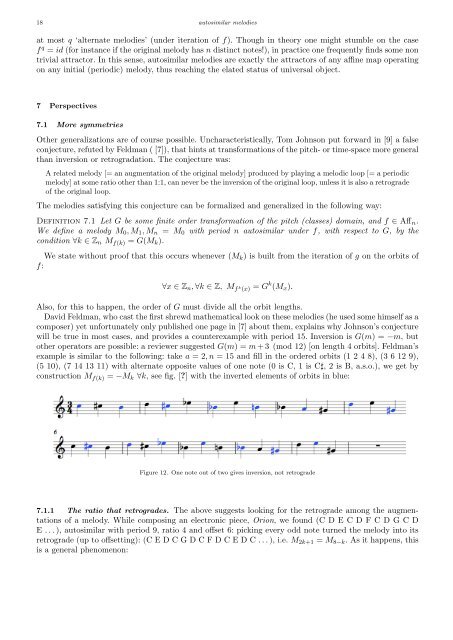
example is similar to the following: take a = 2, n = 15 and fill in the ordered orbits (1 2 4 8), (3 6 12 9),
(5 10), (7 14 13 11) with alternate opposite values of one note (0 is C, 1 is C♯, 2 is B, a.s.o.), we get by
construction M f(k) = −Mk ∀k, see fig. [?] with the inverted elements of orbits in blue:
Figure 12. One note out of two gives inversion, not retrograde
7.1.1 The ratio that retrogrades. The above suggests looking for the retrograde among the augmentations
of a melody. While composing an electronic piece, Orion, we found (C D E C D F C D G C D
E . . . ), autosimilar with period 9, ratio 4 and offset 6: picking every odd note turned the melody into its
retrograde (up to offsetting): (C E D C G D C F D C E D C . . . ), i.e. M2k+1 = M8−k. As it happens, this
is a general phenomenon: