John Stillwell - Naive Lie Theory.pdf - Index of

178 8 Topology
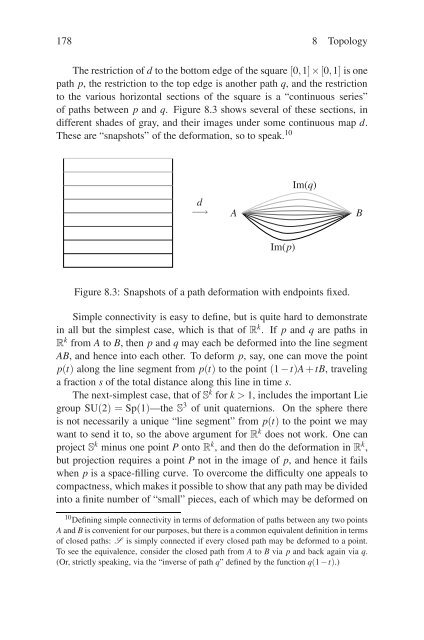
The restriction of d to the bottom edge of the square [0,1]×[0,1] is one
path p, the restriction to the top edge is another path q, and the restriction
to the various horizontal sections of the square is a “continuous series”
of paths between p and q. Figure 8.3 shows several of these sections, in
different shades of gray, and their images under some continuous map d.
These are “snapshots” of the deformation, so to speak. 10
−→ d
A
Im(q)
B
Im(p)
Figure 8.3: Snapshots of a path deformation with endpoints fixed.
Simple connectivity is easy to define, but is quite hard to demonstrate
in all but the simplest case, which is that of R k . If p and q are paths in
R k from A to B, thenp and q may each be deformed into the line segment
AB, and hence into each other. To deform p, say, one can move the point
p(t) along the line segment from p(t) to the point (1 −t)A +tB, traveling
a fraction s of the total distance along this line in time s.
The next-simplest case, that of S k for k > 1, includes the important Lie
group SU(2) =Sp(1)—the S 3 of unit quaternions. On the sphere there
is not necessarily a unique “line segment” from p(t) to the point we may
want to send it to, so the above argument for R k does not work. One can
project S k minus one point P onto R k , and then do the deformation in R k ,
but projection requires a point P not in the image of p, and hence it fails
when p is a space-filling curve. To overcome the difficulty one appeals to
compactness, which makes it possible to show that any path may be divided
into a finite number of “small” pieces, each of which may be deformed on
10 Defining simple connectivity in terms of deformation of paths between any two points
A and B is convenient for our purposes, but there is a common equivalent definition in terms
of closed paths: S is simply connected if every closed path may be deformed to a point.
To see the equivalence, consider the closed path from A to B via p and back again via q.
(Or, strictly speaking, via the “inverse of path q” defined by the function q(1 −t).)