- Page 2 and 3: Undergraduate Texts in Mathematics
- Page 4 and 5: John Stillwell Naive Lie Theory 123
- Page 6 and 7: To Paul Halmos In Memoriam
- Page 8 and 9: viii Preface Where my book diverges
- Page 10 and 11: Contents 1 Geometry of complex numb
- Page 12 and 13: Contents xiii 8 Topology 160 8.1 Op
- Page 14 and 15: 2 1 The geometry of complex numbers
- Page 16 and 17: 4 1 The geometry of complex numbers
- Page 18 and 19: 6 1 The geometry of complex numbers
- Page 20 and 21: 8 1 The geometry of complex numbers
- Page 22 and 23: 10 1 The geometry of complex number
- Page 24 and 25: 12 1 The geometry of complex number
- Page 26 and 27: 14 1 The geometry of complex number
- Page 28 and 29: 16 1 The geometry of complex number
- Page 30 and 31: 18 1 The geometry of complex number
- Page 32 and 33: 20 1 The geometry of complex number
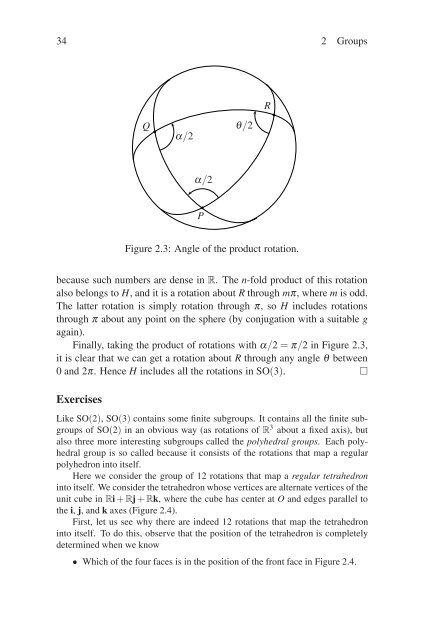
- Page 34 and 35: 22 1 The geometry of complex number
- Page 36 and 37: 24 2 Groups 2.1 Crash course on gro
- Page 38 and 39: 26 2 Groups This algebraic argument
- Page 40 and 41: 28 2 Groups is the right coset of H
- Page 42 and 43: 30 2 Groups and h ∈ ker ϕ ⇒ ϕ
- Page 44 and 45: 32 2 Groups show that the real proj
- Page 48 and 49: 36 2 Groups 1/2 turn 1/3 turn Figur
- Page 50 and 51: 38 2 Groups 2.4.4 Show that reflect
- Page 52 and 53: 40 2 Groups 2.5.1 Check that q ↦
- Page 54 and 55: 42 2 Groups Exercises If we let x 1
- Page 56 and 57: 44 2 Groups SO(4) is not simple. Th
- Page 58 and 59: 46 2 Groups include “infinitesima
- Page 60 and 61: 3 Generalized rotation groups PREVI
- Page 62 and 63: 50 3 Generalized rotation groups Th
- Page 64 and 65: 52 3 Generalized rotation groups An
- Page 66 and 67: 54 3 Generalized rotation groups th
- Page 68 and 69: 56 3 Generalized rotation groups Pa
- Page 70 and 71: 58 3 Generalized rotation groups Ho
- Page 72 and 73: 60 3 Generalized rotation groups On
- Page 74 and 75: 62 3 Generalized rotation groups Ex
- Page 76 and 77: 64 3 Generalized rotation groups In
- Page 78 and 79: 66 3 Generalized rotation groups Th
- Page 80 and 81: 68 3 Generalized rotation groups Ca
- Page 82 and 83: 70 3 Generalized rotation groups Pr
- Page 84 and 85: 72 3 Generalized rotation groups Ma
- Page 86 and 87: 4 The exponential map PREVIEW The g
- Page 88 and 89: 76 4 The exponential map course, th
- Page 90 and 91: 78 4 The exponential map imaginary
- Page 92 and 93: 80 4 The exponential map This const
- Page 94 and 95: 82 4 The exponential map 4.4 The Li
- Page 96 and 97:
84 4 The exponential map 4.5 The ex
- Page 98 and 99:
86 4 The exponential map Definition
- Page 100 and 101:
88 4 The exponential map obtained b
- Page 102 and 103:
90 4 The exponential map Then subst
- Page 104 and 105:
92 4 The exponential map It was dis
- Page 106 and 107:
94 5 The tangent space 5.1 Tangent
- Page 108 and 109:
96 5 The tangent space The matrices
- Page 110 and 111:
98 5 The tangent space as in ordina
- Page 112 and 113:
100 5 The tangent space Conversely,
- Page 114 and 115:
102 5 The tangent space Exercises A
- Page 116 and 117:
104 5 The tangent space To see why
- Page 118 and 119:
106 5 The tangent space 5.5 Dimensi
- Page 120 and 121:
108 5 The tangent space but not nec
- Page 122 and 123:
110 5 The tangent space Conversely,
- Page 124 and 125:
112 5 The tangent space However, th
- Page 126 and 127:
114 5 The tangent space the set of
- Page 128 and 129:
6 Structure of Lie algebras PREVIEW
- Page 130 and 131:
118 6 Structure of Lie algebras isa
- Page 132 and 133:
120 6 Structure of Lie algebras An
- Page 134 and 135:
122 6 Structure of Lie algebras 6.3
- Page 136 and 137:
124 6 Structure of Lie algebras We
- Page 138 and 139:
126 6 Structure of Lie algebras whi
- Page 140 and 141:
128 6 Structure of Lie algebras Our
- Page 142 and 143:
130 6 Structure of Lie algebras [X,
- Page 144 and 145:
132 6 Structure of Lie algebras l
- Page 146 and 147:
134 6 Structure of Lie algebras and
- Page 148 and 149:
136 6 Structure of Lie algebras If
- Page 150 and 151:
138 6 Structure of Lie algebras of
- Page 152 and 153:
140 7 The matrix logarithm 7.1 Loga
- Page 154 and 155:
142 7 The matrix logarithm 7.1.1 Su
- Page 156 and 157:
144 7 The matrix logarithm Taking e
- Page 158 and 159:
146 7 The matrix logarithm If A(t)
- Page 160 and 161:
148 7 The matrix logarithm The log
- Page 162 and 163:
150 7 The matrix logarithm 7.4.1 Sh
- Page 164 and 165:
152 7 The matrix logarithm By the t
- Page 166 and 167:
154 7 The matrix logarithm The idea
- Page 168 and 169:
156 7 The matrix logarithm Next, re
- Page 170 and 171:
158 7 The matrix logarithm Exercise
- Page 172 and 173:
8 Topology PREVIEW One of the essen
- Page 174 and 175:
162 8 Topology The set N ε (P) is
- Page 176 and 177:
164 8 Topology 8.2 Closed matrix gr
- Page 178 and 179:
166 8 Topology Matrix Lie groups Wi
- Page 180 and 181:
168 8 Topology also a continuous fu
- Page 182 and 183:
170 8 Topology Pick, say, the leftm
- Page 184 and 185:
172 8 Topology true that f −1 (
- Page 186 and 187:
174 8 Topology describing specific
- Page 188 and 189:
176 8 Topology These roots represen
- Page 190 and 191:
178 8 Topology The restriction of d
- Page 192 and 193:
180 8 Topology can divide [0,1] int
- Page 194 and 195:
182 8 Topology 8.8 Discussion Close
- Page 196 and 197:
184 8 Topology topology book will s
- Page 198 and 199:
9 Simply connected Lie groups PREVI
- Page 200 and 201:
188 9 Simply connected Lie groups T
- Page 202 and 203:
190 9 Simply connected Lie groups I
- Page 204 and 205:
192 9 Simply connected Lie groups f
- Page 206 and 207:
194 9 Simply connected Lie groups 9
- Page 208 and 209:
196 9 Simply connected Lie groups T
- Page 210 and 211:
198 9 Simply connected Lie groups W
- Page 212 and 213:
200 9 Simply connected Lie groups L
- Page 214 and 215:
202 9 Simply connected Lie groups L
- Page 216 and 217:
Bibliography J. Frank Adams. Lectur
- Page 218 and 219:
206 Bibliography Otto Schreier. Abs
- Page 220 and 221:
208 Index and continuity, 171 and u
- Page 222 and 223:
210 Index Lorentz, 113 matrix, vii,
- Page 224 and 225:
212 Index knew SO(4) anomaly, 47 Tr
- Page 226 and 227:
214 Index projective space, 185 rea
- Page 228 and 229:
216 Index is semisimple, 47 so(4) i
- Page 230:
Undergraduate Texts in Mathematics