John Stillwell - Naive Lie Theory.pdf - Index of

8.7 Simple connectedness 179
the sphere to a “line segment” (a great circle arc). This clears space on the
sphere that enables the projection method to work. For more details see the
exercises below.
Compactness is also important in proving that certain groups are not
simply connected. The most important case is the circle S 1 = SO(2),which
we now study in detail, because the idea of “lifting,” introduced here, will
be important in Chapter 9.
The circle and the line
The function f (θ) =(cos θ,sin θ) maps R onto the unit circle S 1 . It is
called a covering of S 1 by R and the points θ +2nπ ∈ R are said to lie over
the point (cos θ,sin θ) ∈ S 1 . This map is far from being 1-to-1, because
infinitely many points of R lie over each point of S 1 . For example, the
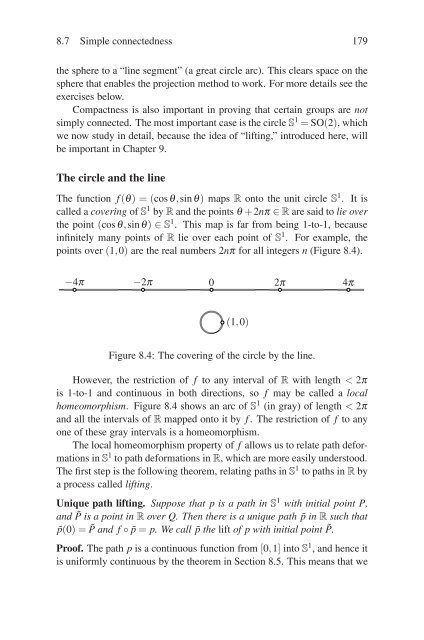
points over (1,0) are the real numbers 2nπ for all integers n (Figure 8.4).
−4π
−2π
0 2π 4π
(1,0)
Figure 8.4: The covering of the circle by the line.
However, the restriction of f to any interval of R with length < 2π
is 1-to-1 and continuous in both directions, so f may be called a local
homeomorphism. Figure 8.4 shows an arc of S 1 (in gray) of length < 2π
and all the intervals of R mapped onto it by f . The restriction of f to any
one of these gray intervals is a homeomorphism.
The local homeomorphism property of f allows us to relate path deformations
in S 1 to path deformations in R, which are more easily understood.
The first step is the following theorem, relating paths in S 1 to paths in R by
a process called lifting.
Unique path lifting. Suppose that p is a path in S 1 with initial point P,
and ˜P is a point in R over Q. Then there is a unique path ˜p inR such that
˜p(0)= ˜P and f ◦ ˜p = p. We call ˜pthelift of p with initial point ˜P.
Proof. The path p is a continuous function from [0,1] into S 1 , and hence it
is uniformly continuous by the theorem in Section 8.5. This means that we