Chapter 7 Rational Functions - College of the Redwoods

More documents

Recommendations

Info

$Math 50A February 18, 2011 Quiz #10 Name: David Arnold ...$

$College of the Redwoods Math Lab What is the Math Lab course? The$

$Math Department Meeting Minutes April 19, 2013 in PS 117 3-â€5pm ...$

714 Chapter 7 Rational Functions Make one side zero. x 2 ( 2 x 2 ) = ( 1 − 2 ) x 2 x 2 = x 2 − 2x 0 = x 2 − 2x − 2 The right-hand side is a quadratic trinomial with ac = (1)(−2) = −2. There are no integer pairs with product −2 that sum to −2, so this quadratic trinomial does not factor. Fortunately, the equation is quadratic (second degree), so we can use the quadratic formula with a = 1, b = −2, and c = −2. x = −b ± √ b 2 − 4ac 2a = −(−2) ± √ (−2) 2 − 4(1)(−2) 2(1) = 2 ± √ 12 2 This gives us two solutions, x = (2 − √ 12)/2 and x = (2 + √ 12)/2. Let’s check the solution x = (2 − √ 12)/2. First, enter this result in your calculator, press the STO◮ button, press X, then press the ENTER key to execute the command and store the solution in the variable X. This command is shown in Figure 2(a). Enter the left-hand side of the original equation (6) as 2/xˆ2 and press the ENTER key to execute this command. This is shown in Figure 2(b). Enter the right-hand side of the original equation (6) as 1-2/X and press the ENTER key to execute this command. This is shown in Figure 2(c). Note that the left- and right-hand sides of equation (6) are both shown to equal 3.732050808 at x = (2 − √ 12)/2 (at X = -0.7320508076), as shown in Figure 2(c). This shows that x = (2 − √ 12)/2 is a solution of equation (6). We leave it to our readers to check the second solution, x = (2 + √ 12)/2. (a) (b) (c) Figure 2. Using the graphing calculator to check the solutions of equation (6). Let’s look at another example, this one involving function notation. ◮ Example 7. Consider the function defined by f(x) = 1 x + 1 x − 4 . (8) Version: Fall 2007
Section 7.7 Solving Rational Equations 715 Solve the equation f(x) = 2 for x using both graphical and analytical techniques, then compare solutions. Perform each of the following tasks. a. Sketch the graph of f on graph paper. Label the zeros of f with their coordinates and the asymptotes of f with their equations. b. Add the graph of y = 2 to your plot and estimate the coordinates of where the graph of f intersects the graph of y = 2. c. Use the intersect utility on your calculator to find better approximations of the points where the graphs of f and y = 2 intersect. d. Solve the equation f(x) = 2 algebraically and compare your solutions to those found in part (c). For the graph in part (a), we need to find the zeros of f and the equations of any vertical or horizontal asymptotes. To find the zero of the function f, we find a common denominator and add the two rational expressions in equation (8). f(x) = 1 x + 1 x − 4 = x − 4 x(x − 4) + x x(x − 4) = 2x − 4 x(x − 4) Note that the numerator of this result equal zero (but not the denominator) when x = 2. This is the zero of f. Thus, the graph of f has x-intercept at (2, 0), as shown in Figure 4. Note that the rational function in equation (9) is reduced to lowest terms. The denominators of x and x + 4 in equation (9) are zero when x = 0 and x = 4. These are our vertical asymptotes, as shown in Figure 4. 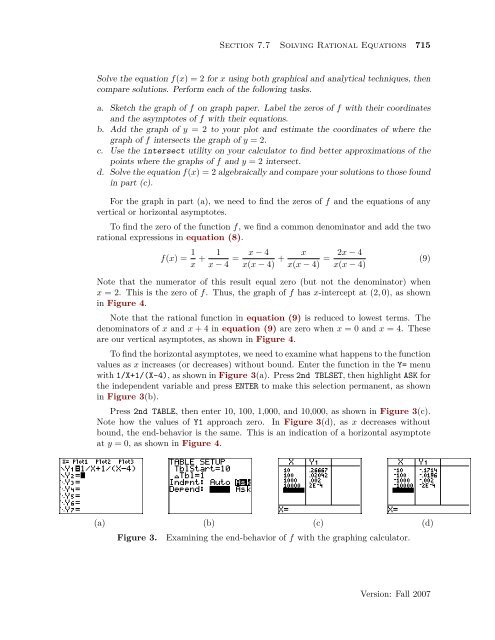To find the horizontal asymptotes, we need to examine what happens to the function values as x increases (or decreases) without bound. Enter the function in the Y= menu with 1/X+1/(X-4), as shown in Figure 3(a). Press 2nd TBLSET, then highlight ASK for the independent variable and press ENTER to make this selection permanent, as shown in Figure 3(b). Press 2nd TABLE, then enter 10, 100, 1,000, and 10,000, as shown in Figure 3(c). Note how the values of Y1 approach zero. In Figure 3(d), as x decreases without bound, the end-behavior is the same. This is an indication of a horizontal asymptote at y = 0, as shown in Figure 4. (9) (a) (b) (c) (d) Figure 3. Examining the end-behavior of f with the graphing calculator. Version: Fall 2007
Page 1 and 2:
7 Rational Functions In this chapte
Page 3 and 4:
Section 7.1 Introducing Rational Fu
Page 5 and 6:
Page 7 and 8:
Page 9 and 10:
Page 11 and 12:
Page 13 and 14:
Page 15 and 16:
Page 17 and 18:
Page 19 and 20:
Section 7.2 Reducing Rational Funct
Page 21 and 22:
Page 23 and 24:
Page 25 and 26:
Page 27 and 28:
Page 29 and 30:
Page 31 and 32:
Page 33 and 34:
Page 35 and 36:
Page 37 and 38:
Page 39 and 40:
Section 7.3 Graphing Rational Funct
Page 41 and 42:
Page 43 and 44:
Page 45 and 46:
Page 47 and 48:
Page 49 and 50:
Page 51 and 52:
Page 53 and 54:
Page 55 and 56:
Page 57 and 58:
Page 59 and 60:
Page 61 and 62:
Section 7.4 Products and Quotients
Page 63 and 64: Section 7.4 Products and Quotients
Page 79 and 80: Section 7.5 Sums and Differences of
Page 95 and 96: Section 7.6 Complex Fractions 695 7
Page 97 and 98: Section 7.6 Complex Fractions 697 (
Page 99 and 100: Section 7.6 Complex Fractions 699 (
Page 101 and 102: Section 7.6 Complex Fractions 701 W
Page 111 and 112: Section 7.7 Solving Rational Equati
Page 113: Section 7.7 Solving Rational Equati
Page 131 and 132: Section 7.8 Applications of Rationa
Page 147 and 148: Section 7.9 Index 747 7.9 Index a a
show all

Chapter 7 Rational Functions - College of the Redwoods

Create successful ePaper yourself

Delete template?

Save as template?