Chapter 7 Rational Functions - College of the Redwoods
Chapter 7 Rational Functions - College of the Redwoods
Chapter 7 Rational Functions - College of the Redwoods
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
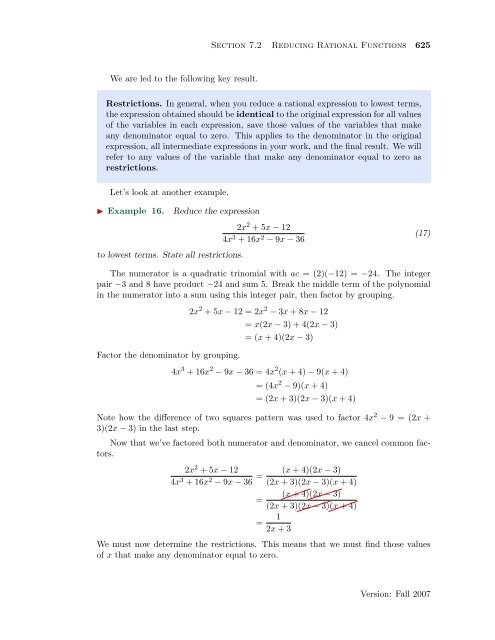
Section 7.2 Reducing <strong>Rational</strong> <strong>Functions</strong> 625<br />
We are led to <strong>the</strong> following key result.<br />
Restrictions. In general, when you reduce a rational expression to lowest terms,<br />
<strong>the</strong> expression obtained should be identical to <strong>the</strong> original expression for all values<br />
<strong>of</strong> <strong>the</strong> variables in each expression, save those values <strong>of</strong> <strong>the</strong> variables that make<br />
any denominator equal to zero. This applies to <strong>the</strong> denominator in <strong>the</strong> original<br />
expression, all intermediate expressions in your work, and <strong>the</strong> final result. We will<br />
refer to any values <strong>of</strong> <strong>the</strong> variable that make any denominator equal to zero as<br />
restrictions.<br />
Let’s look at ano<strong>the</strong>r example.<br />
◮ Example 16.<br />
Reduce <strong>the</strong> expression<br />
to lowest terms. State all restrictions.<br />
2x 2 + 5x − 12<br />
4x 3 + 16x 2 − 9x − 36<br />
(17)<br />
The numerator is a quadratic trinomial with ac = (2)(−12) = −24. The integer<br />
pair −3 and 8 have product −24 and sum 5. Break <strong>the</strong> middle term <strong>of</strong> <strong>the</strong> polynomial<br />
in <strong>the</strong> numerator into a sum using this integer pair, <strong>the</strong>n factor by grouping.<br />
Factor <strong>the</strong> denominator by grouping.<br />
2x 2 + 5x − 12 = 2x 2 − 3x + 8x − 12<br />
= x(2x − 3) + 4(2x − 3)<br />
= (x + 4)(2x − 3)<br />
4x 3 + 16x 2 − 9x − 36 = 4x 2 (x + 4) − 9(x + 4)<br />
= (4x 2 − 9)(x + 4)<br />
= (2x + 3)(2x − 3)(x + 4)<br />
Note how <strong>the</strong> difference <strong>of</strong> two squares pattern was used to factor 4x 2 − 9 = (2x +<br />
3)(2x − 3) in <strong>the</strong> last step.<br />
Now that we’ve factored both numerator and denominator, we cancel common factors.<br />
2x 2 + 5x − 12<br />
4x 3 + 16x 2 − 9x − 36 = (x + 4)(2x − 3)<br />
(2x + 3)(2x − 3)(x + 4)<br />
(x + 4)(2x − 3)<br />
=<br />
(2x + 3)(2x − 3)(x + 4)<br />
= 1<br />
2x + 3<br />
We must now determine <strong>the</strong> restrictions. This means that we must find those values<br />
<strong>of</strong> x that make any denominator equal to zero.<br />
Version: Fall 2007