FOUNDATIONS OF QUANTUM MECHANICS

A. 3. FORMULATION OF THE PROBLEM ON THE SURFACE OF A SPHERE 191
above while taking ϕ from 0 to 2π and changing over just often enough to descend the required
distance, but we will show it can also be done taking a path along two great circles only, again
starting in their most northern points.
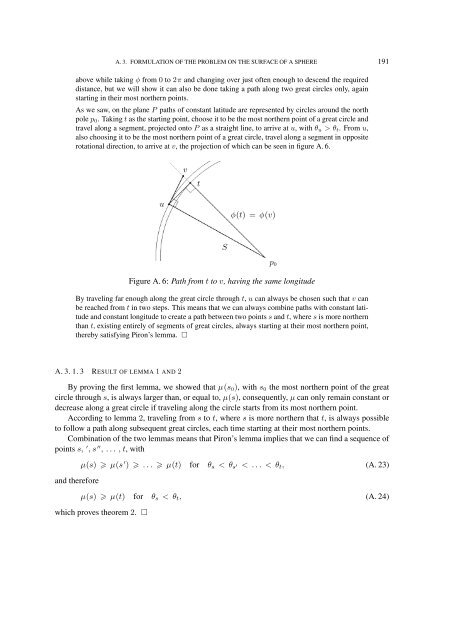
As we saw, on the plane P paths of constant latitude are represented by circles around the north
pole p 0 . Taking t as the starting point, choose it to be the most northern point of a great circle and
travel along a segment, projected onto P as a straight line, to arrive at u, with θ u > θ t . From u,
also choosing it to be the most northern point of a great circle, travel along a segment in opposite
rotational direction, to arrive at v, the projection of which can be seen in figure A. 6.
v
t
u
ϕ(t) = ϕ(v)
S
Figure A. 6: Path from t to v, having the same longitude
By traveling far enough along the great circle through t, u can always be chosen such that v can
be reached from t in two steps. This means that we can always combine paths with constant latitude
and constant longitude to create a path between two points s and t, where s is more northern
than t, existing entirely of segments of great circles, always starting at their most northern point,
thereby satisfying Piron’s lemma. □
p 0
A. 3. 1. 3 RESULT OF LEMMA 1 AND 2
By proving the first lemma, we showed that µ(s 0 ), with s 0 the most northern point of the great
circle through s, is always larger than, or equal to, µ(s), consequently, µ can only remain constant or
decrease along a great circle if traveling along the circle starts from its most northern point.
According to lemma 2, traveling from s to t, where s is more northern that t, is always possible
to follow a path along subsequent great circles, each time starting at their most northern points.
Combination of the two lemmas means that Piron’s lemma implies that we can find a sequence of
points s, ′ , s ′′ , . . . , t, with
and therefore
µ(s) µ(s ′ ) . . . µ(t) for θ s < θ s ′ < . . . < θ t , (A. 23)
µ(s) µ(t) for θ s < θ t , (A. 24)
which proves theorem 2. □