FOUNDATIONS OF QUANTUM MECHANICS

194 APPENDIX A. GLEASON’S THEOREM
Therefore, if s moves southwards, passing through p, and simultaneously s ⊥ moves northwards,
passing through q, the value of µ(s ⊥ ) also has to jump discontinuously. If µ(s) jumps with −ε,
then µ(s ⊥ ) jumps with ε.
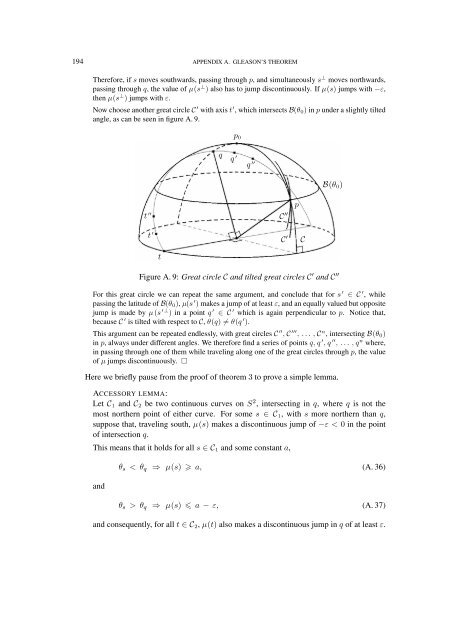
Now choose another great circle C ′ with axis t ′ , which intersects B(θ 0 ) in p under a slightly tilted
angle, as can be seen in figure A. 9.
p 0
q
q ′ q ′′ B(θ 0 )
t ′′
C ′′
p
t ′
C ′
C
t
Figure A. 9: Great circle C and tilted great circles C ′ and C ′′
For this great circle we can repeat the same argument, and conclude that for s ′ ∈ C ′ , while
passing the latitude of B(θ 0 ), µ(s ′ ) makes a jump of at least ε, and an equally valued but opposite
jump is made by µ (s ′⊥ ) in a point q ′ ∈ C ′ which is again perpendicular to p. Notice that,
because C ′ is tilted with respect to C, θ(q) ≠ θ(q ′ ).
This argument can be repeated endlessly, with great circles C ′′ , C ′′′ , . . . , C n , intersecting B(θ 0 )
in p, always under different angles. We therefore find a series of points q, q ′ , q ′′ , . . . , q n where,
in passing through one of them while traveling along one of the great circles through p, the value
of µ jumps discontinuously. □
Here we briefly pause from the proof of theorem 3 to prove a simple lemma.
ACCESSORY LEMMA:
Let C 1 and C 2 be two continuous curves on S 2 , intersecting in q, where q is not the
most northern point of either curve. For some s ∈ C 1 , with s more northern than q,
suppose that, traveling south, µ(s) makes a discontinuous jump of −ε < 0 in the point
of intersection q.
This means that it holds for all s ∈ C 1 and some constant a,
and
θ s < θ q ⇒ µ(s) a, (A. 36)
θ s > θ q ⇒ µ(s) a − ε, (A. 37)
and consequently, for all t ∈ C 2 , µ(t) also makes a discontinuous jump in q of at least ε.