Woo Young Lee Lecture Notes on Operator Theory

CHAPTER 5.
TOEPLITZ THEORY
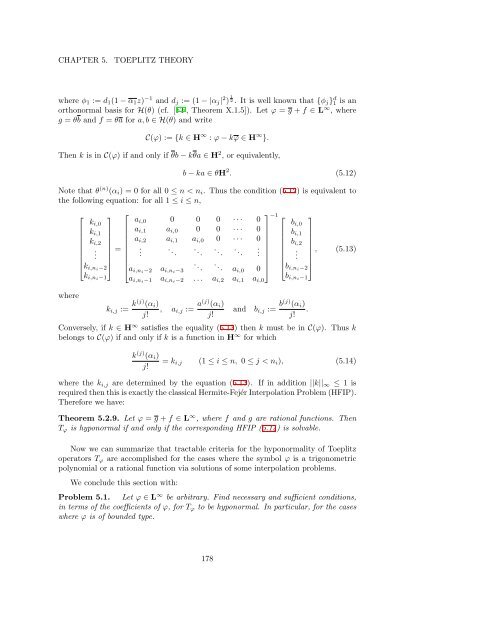
where ϕ 1 := d 1 (1 − α 1 z) −1 and d j := (1 − |α j | 2 ) 1 2 . It is well known that {ϕ j } d 1 is an
orthonormal basis for H(θ) (cf. [FF, Theorem X.1.5]). Let φ = g + f ∈ L ∞ , where
g = θb and f = θa for a, b ∈ H(θ) and write
C(φ) := {k ∈ H ∞ : φ − kφ ∈ H ∞ }.
Then k is in C(φ) if and only if θb − kθa ∈ H 2 , or equivalently,
b − ka ∈ θH 2 . (5.12)
Note that θ (n) (α i ) = 0 for all 0 ≤ n < n i . Thus the condition (5.12) is equivalent to
the following equation: for all 1 ≤ i ≤ n,
where
⎡ ⎤
k i,0
k i,1
k i,2
⎢ .
⎥
⎣k i,ni
⎦
−2
k i,ni−1
⎡
⎤
a i,0 0 0 0 · · · 0
a i,1 a i,0 0 0 · · · 0
a i,2 a i,1 a i,0 0 · · · 0
=
. . .. . .. . .. . .. . ⎢
⎣
.
a i,ni−2 a .. . ⎥
.. i,ni−3
ai,0 0 ⎦
a i,ni −1 a i,ni −2 . . . a i,2 a i,1 a i,0
k i,j := k(j) (α i )
, a i,j := a(j) (α i )
j!
j!
−1 ⎡
⎢
⎣
b i,0
b i,1
b i,2
. .
b i,ni −2
b i,ni−1
and b i,j := b(j) (α i )
.
j!
⎤
, (5.13)
⎥
⎦
Conversely, if k ∈ H ∞ satisfies the equality (5.13) then k must be in C(φ). Thus k
belongs to C(φ) if and only if k is a function in H ∞ for which
k (j) (α i )
j!
= k i,j (1 ≤ i ≤ n, 0 ≤ j < n i ), (5.14)
where the k i,j are determined by the equation (5.13). If in addition ||k|| ∞ ≤ 1 is
required then this is exactly the classical Hermite-Fejér Interpolation Problem (HFIP).
Therefore we have:
Theorem 5.2.9. Let φ = g + f ∈ L ∞ , where f and g are rational functions. Then
T φ is hyponormal if and only if the corresponding HFIP (5.14) is solvable.
Now we can summarize that tractable criteria for the hyponormality of Toeplitz
operators T φ are accomplished for the cases where the symbol φ is a trigonometric
polynomial or a rational function via solutions of some interpolation problems.
We conclude this section with:
Problem 5.1. Let φ ∈ L ∞ be arbitrary. Find necessary and sufficient conditions,
in terms of the coefficients of φ, for T φ to be hyponormal. In particular, for the cases
where φ is of bounded type.
178