The Geometry of Ships
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
THE GEOMETRY OF SHIPS 19<br />
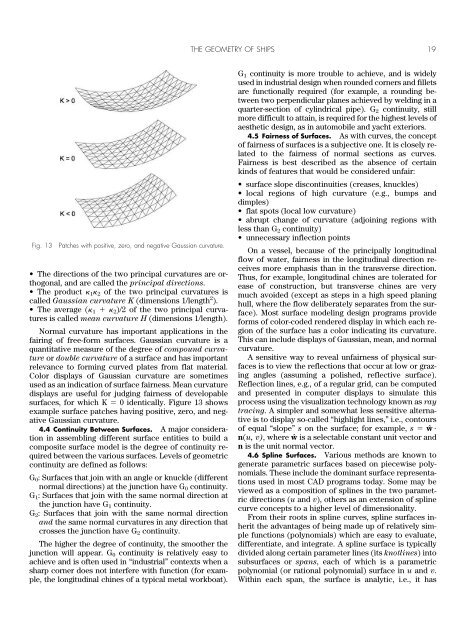
Fig. 13<br />
Patches with positive, zero, and negative Gaussian curvature.<br />
• <strong>The</strong> directions <strong>of</strong> the two principal curvatures are orthogonal,<br />
and are called the principal directions.<br />
• <strong>The</strong> product 1 2 <strong>of</strong> the two principal curvatures is<br />
called Gaussian curvature K (dimensions 1/length 2 ).<br />
• <strong>The</strong> average ( 1 2 )/2 <strong>of</strong> the two principal curvatures<br />
is called mean curvature H (dimensions 1/length).<br />
Normal curvature has important applications in the<br />
fairing <strong>of</strong> free-form surfaces. Gaussian curvature is a<br />
quantitative measure <strong>of</strong> the degree <strong>of</strong> compound curvature<br />
or double curvature <strong>of</strong> a surface and has important<br />
relevance to forming curved plates from flat material.<br />
Color displays <strong>of</strong> Gaussian curvature are sometimes<br />
used as an indication <strong>of</strong> surface fairness. Mean curvature<br />
displays are useful for judging fairness <strong>of</strong> developable<br />
surfaces, for which K 0 identically. Figure 13 shows<br />
example surface patches having positive, zero, and negative<br />
Gaussian curvature.<br />
4.4 Continuity Between Surfaces. A major consideration<br />
in assembling different surface entities to build a<br />
composite surface model is the degree <strong>of</strong> continuity required<br />
between the various surfaces. Levels <strong>of</strong> geometric<br />
continuity are defined as follows:<br />
G 0 : Surfaces that join with an angle or knuckle (different<br />
normal directions) at the junction have G 0 continuity.<br />
G 1 : Surfaces that join with the same normal direction at<br />
the junction have G 1 continuity.<br />
G 2 : Surfaces that join with the same normal direction<br />
and the same normal curvatures in any direction that<br />
crosses the junction have G 2 continuity.<br />
<strong>The</strong> higher the degree <strong>of</strong> continuity, the smoother the<br />
junction will appear. G 0 continuity is relatively easy to<br />
achieve and is <strong>of</strong>ten used in “industrial” contexts when a<br />
sharp corner does not interfere with function (for example,<br />
the longitudinal chines <strong>of</strong> a typical metal workboat).<br />
G 1 continuity is more trouble to achieve, and is widely<br />
used in industrial design when rounded corners and fillets<br />
are functionally required (for example, a rounding between<br />
two perpendicular planes achieved by welding in a<br />
quarter-section <strong>of</strong> cylindrical pipe). G 2 continuity, still<br />
more difficult to attain, is required for the highest levels <strong>of</strong><br />
aesthetic design, as in automobile and yacht exteriors.<br />
4.5 Fairness <strong>of</strong> Surfaces. As with curves, the concept<br />
<strong>of</strong> fairness <strong>of</strong> surfaces is a subjective one. It is closely related<br />
to the fairness <strong>of</strong> normal sections as curves.<br />
Fairness is best described as the absence <strong>of</strong> certain<br />
kinds <strong>of</strong> features that would be considered unfair:<br />
• surface slope discontinuities (creases, knuckles)<br />
• local regions <strong>of</strong> high curvature (e.g., bumps and<br />
dimples)<br />
• flat spots (local low curvature)<br />
• abrupt change <strong>of</strong> curvature (adjoining regions with<br />
less than G 2 continuity)<br />
• unnecessary inflection points<br />
On a vessel, because <strong>of</strong> the principally longitudinal<br />
flow <strong>of</strong> water, fairness in the longitudinal direction receives<br />
more emphasis than in the transverse direction.<br />
Thus, for example, longitudinal chines are tolerated for<br />
ease <strong>of</strong> construction, but transverse chines are very<br />
much avoided (except as steps in a high speed planing<br />
hull, where the flow deliberately separates from the surface).<br />
Most surface modeling design programs provide<br />
forms <strong>of</strong> color-coded rendered display in which each region<br />
<strong>of</strong> the surface has a color indicating its curvature.<br />
This can include displays <strong>of</strong> Gaussian, mean, and normal<br />
curvature.<br />
A sensitive way to reveal unfairness <strong>of</strong> physical surfaces<br />
is to view the reflections that occur at low or grazing<br />
angles (assuming a polished, reflective surface).<br />
Reflection lines, e.g., <strong>of</strong> a regular grid, can be computed<br />
and presented in computer displays to simulate this<br />
process using the visualization technology known as ray<br />
tracing. A simpler and somewhat less sensitive alternative<br />
is to display so-called “highlight lines,” i.e., contours<br />
<strong>of</strong> equal “slope” s on the surface; for example, s ŵ <br />
n(u, v), where ŵ is a selectable constant unit vector and<br />
n is the unit normal vector.<br />
4.6 Spline Surfaces. Various methods are known to<br />
generate parametric surfaces based on piecewise polynomials.<br />
<strong>The</strong>se include the dominant surface representations<br />
used in most CAD programs today. Some may be<br />
viewed as a composition <strong>of</strong> splines in the two parametric<br />
directions (u and v), others as an extension <strong>of</strong> spline<br />
curve concepts to a higher level <strong>of</strong> dimensionality.<br />
From their roots in spline curves, spline surfaces inherit<br />
the advantages <strong>of</strong> being made up <strong>of</strong> relatively simple<br />
functions (polynomials) which are easy to evaluate,<br />
differentiate, and integrate. A spline surface is typically<br />
divided along certain parameter lines (its knotlines) into<br />
subsurfaces or spans, each <strong>of</strong> which is a parametric<br />
polynomial (or rational polynomial) surface in u and v.<br />
Within each span, the surface is analytic, i.e., it has