The Geometry of Ships

THE GEOMETRY OF SHIPS 43
The general principles of weight prediction are wellknown.
Weight is the product of mass times acceleration
due to gravity, g. The total mass will be the sum of
all component masses, and the center of mass (or center
of gravity) can be figured by accumulating x, y, z
moments:
(91)
{M x
,M y
,M z
} {x i
,y i
,z i
}m i (92)
i
where m i is a component mass and {x i , y i , z i } is the location
of its center of mass. The resultant center of mass
(center of gravity) has coordinates
{x G , y G , z G } {M x /m, M y /m, M z /m}. (93)
In SI units, the mass units in naval architecture are
typically kg for small craft, or metric tons for ships, and
the term “weight estimate,” although widely used, is
something of a misnomer. Weights, i.e., the forces exerted
by gravity on these masses, are used in such applications
as static equilibrium and stability analysis.
In some situations, primarily in regard to dynamic
analysis of maneuvering and motions in waves, the mass
moments of inertia are also of importance. The total moments
of inertia with respect to the global coordinates x,
y, z are defined as follows:
I
I
I
I
I
m
I xx
[m i
(y 2 i z2)(i ) ]
i xx i
I
I yy
[m i
(z 2 i x2 i )(i yy ) i ]
I zz
[m i
(x 2 i y2 i )(i zz ) i ]
I xy
I yx
[m i
x i
y i
(i xy
) i
]
I yz
I zy
[m i
y i
z i
(i yz
) i
]
I zx
I xz
[m i
z i
x i
(i zx
) i
]
(94)
(95)
(96)
(97)
(98)
(99)
where m i is the mass of the ith item, and (i xx ) i , (i xy ) i ,
etc., are its mass moments of inertia with respect to its
own center of mass.
The mass moments of inertia of the complete ship
about its center of mass are obtained from the parallelaxis
theorem. Let x, y, z be the centroidal coordinate
frame parallel to the global coordinates, with origin at
the center of mass, i.e., x x x G , etc. Then the components
of mass moments of inertia with respect to the
centroidal frame are:
I xx I xx M (yG 2 zG) 2 (100)
I yy I yy M (zG 2 xG) 2 (101)
I zz I zz M (xG 2 yG) 2 (102)
i
m i
I xy I xy M x G y G (103)
I yz I yz M y G z G (104)
I zx I zx M z G x G (105)
The weight schedule is a table of weights, centroids,
and moments arranged to facilitate the above calculations.
Today it is most commonly maintained as a
spreadsheet, with the tremendous advantage that its totals
can be updated continuously as component
weights are added and revised. Often it is useful to categorize
weight components into groups, e.g., hull,
propulsion, tanks, and cargo. Some 3-D modelers allow
unit weights to be assigned to geometric elements, and
will maintain a weight schedule that dynamically updates
to reflect changes in geometry, as well as unit
weights.
Some component weights can be treated as points,
e.g., an engine or an item of hardware. Some weights are
distributed over curves and surfaces; their mass calculation
has been outlined in Sections 3 and 4. Weights that
are complex-shaped volumes or solids are generally the
most difficult to evaluate; for example, ballast castings
and tank contents. Here the general techniques of volume
and centroid computation developed for hydrostatics
can be brought to bear.
Of course, the vessel can vary from the design during
construction. The architect, builder, and owner/operator
all have an interest in monitoring weights and center of
gravity throughout construction and outfitting so the
flotation, stability, capacity, and performance requirements
and objectives are met when the vessel is placed
in service. Weight analysis and flotation calculations are
an ongoing concern during operation of the vessel, too,
as cargo and stores are loaded and unloaded. Often this
is performed by on-board computer programs which
contain a geometric description of the ship and its partitioning
into cargo spaces and tanks.
9.6 Hydrostatic Stability. Hydrostatic stability is the
principal topic of Moore (2009) and Tagg (2009). Here
we provide a brief introduction relating the subject to
vessel geometry, and focusing primarily on an upright
equilibrium attitude.
Archimedes’ principle provides necessary and sufficient
conditions for a floating object to be in equilibrium.
However, further analysis is required to determine
whether such an equilibrium is stable. The
general topic of stability of equilibrium examines
whether, following a small disturbance that moves a
given system away from equilibrium, the system tends
to restore itself to equilibrium, or to move farther away
from it.
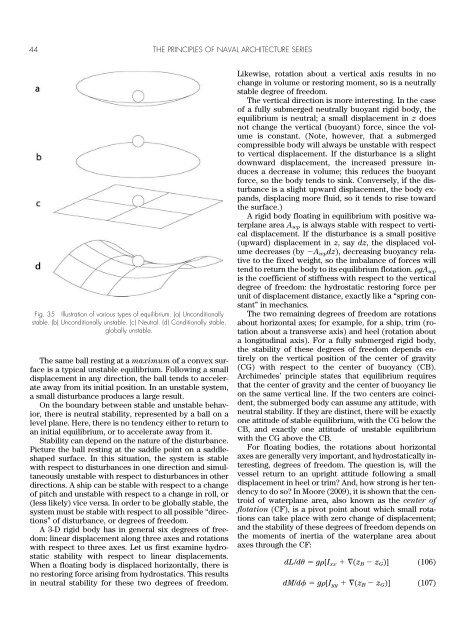
A ball resting at the low point of a concave surface is
a prototype of stable equilibrium (Fig. 35). If the ball is
pushed a little away from center, it tends to roll back. Its
characteristic motion under this restoring force is an oscillation
about the equilibrium position. Another way to
characterize stable behavior is that a small disturbance
produces small results.