The Geometry of Ships

THE GEOMETRY OF SHIPS 41
Alternatively, we could choose P 0 and Q x; then
(63)
Often the boundary C can be approximated as a
closed polygon (polyline) P of N segments C i , i 0,...N
1, connecting points x i , i 0,...N 1, where each x i
is a two-component vector {x i , y i }. (The last segment
connects point x N1 back to point x 0 .) The line integral
equation (63) becomes a sum over the N straight
segments:
(64)
Segment i runs from x i1 to x i ; it can be parameterized
as x i1 (1 t) x i t, with 0 t 1; i.e.,
x x i1 (1 t) x i t, (65)
y y i1 (1 t) y i t (66)
so dy (y i y i1 )dt. Thus the contribution from C i is
A i
1
0
A
N
i1
R
(x i1
x i
)(y i
y i1
)/2
(67)
and this allows the enclosed area A to be easily computed
as the sum of N such terms.
Note that the polygonal region R cannot have any interior
holes because its boundary is defined to be a single
closed polygon. However, polygonal holes are easily
allowed for by applying the same formula [equation (67)]
to each hole and subtracting their areas from the areas
of the outer boundary.
This same general scheme can be applied to compute
integrals of other polynomial quantities over arbitrary
polygonal regions. The first moments of area of R with
respect to x and y are defined as
{m x
, m y
}
(68)
These have dimensions of length cubed. m x can be
put in the form of Green’s theorem by choosing Q 0
and P xy; then
m x
(69)
Similarly, m y can be put in the form of Greens’s theorem
by choosing Q 0 and P y 2 /2; then
m y
dx dy
A A i
where A i
[x i1
(1t)x i
t](y i
y i1
)dt
R
x dy
R
{x, y} dx dy
xy dx
R
y 2 /2dx
R
C i
xdy
(70)
Replacing the boundary with a polygon as before, the
x- and y-moments of area are:
m x
(71)
(72)
There is a need for some purposes to compute moments
of inertia of plane regions. Moments of inertia
with respect to the origin are defined as follows:
(73)
These have units of length to the fourth power.
Application of Green’s theorem and calculations similar
to the above for an arbitrary closed polygon result in:
I xx
I xy
I yy
N
i1
N
i1
N
i1
N
i1
N
i1
(x i
x i1
)(2y i1
x i1
{I xx
, I xy
, I yy
}
(x i
x i1
)(y 3 i1
(x i
x i1
)(3x i1
y 2 i1
x x i1
y 2 i
y 2 i1 2x i1 y i1 y i
i
(x i
x i1
)(3x 2 i1 y i1
x2 i1 y i 2x i1 x i y i1 x2 i y i1
(74)
(75)
(76)
The centroid (center of area) is the point with coordinates
m x /A, m y /A. Note that the centroid is undefined
for a polygon with zero enclosed area, whereas the moments
are always well defined. This suggests postponing
the division, de-emphasizing section centroids, and performing
calculations with moments as far as possible,
especially in automated calculations where attempting a
division by zero will either halt the program or produce
erroneous results.
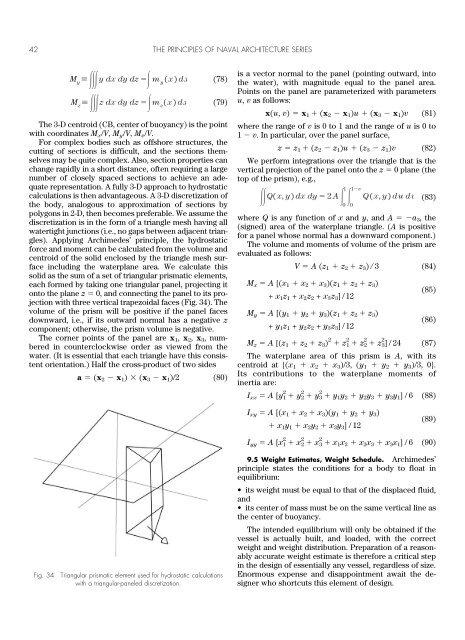
For example, there is generally no need to calculate
the centroid of a section. The 3-D moments of displaced
volume for a ship are
M x
2x i1
x i
y i
3x 2 i y i )/12
y i1
x i
y i
x i1
2y i
x i
)/6
m y
(x i
x i1
)(y 2 i1 y i y i1 y2 i )/6
{y 2 ,xy,x 2 } dx dy
R
y 2 i1 y i y i1 y2 i y3 i )/12
2x i
y i1
y i
3x i
y 2 i )/24
xdxdydz xS(x) dx
(77)