PDF (1016 kB)

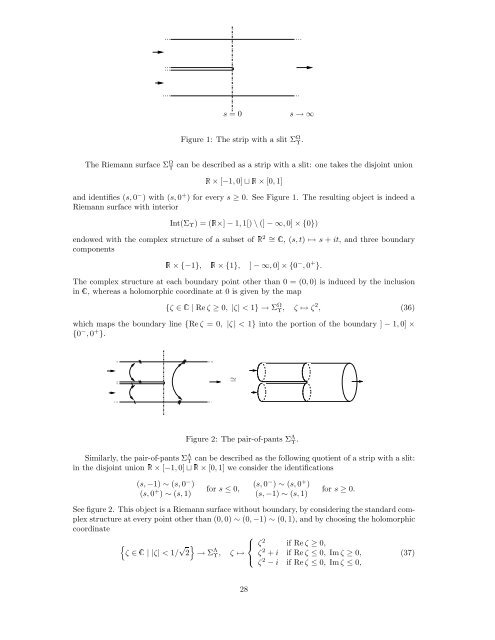
The Riemann surface Σ Ω Υ
s = 0 s → ∞
Figure 1: The strip with a slit Σ Ω Υ .
can be described as a strip with a slit: one takes the disjoint union
Ê×[−1, 0] ⊔Ê×[0, 1]
and identifies (s, 0 − ) with (s, 0 + ) for every s ≥ 0. See Figure 1. The resulting object is indeed a
Riemann surface with interior
Int(ΣΥ) = (Ê×] − 1, 1[) \ (] − ∞, 0] × {0})
endowed with the complex structure of a subset ofÊ2 ∼ =�, (s, t) ↦→ s + it, and three boundary
components Ê×{−1},Ê×{1}, ] − ∞, 0] × {0 − , 0 + }.
The complex structure at each boundary point other than 0 = (0, 0) is induced by the inclusion
in�, whereas a holomorphic coordinate at 0 is given by the map
{ζ ∈�|Re ζ ≥ 0, |ζ| < 1} → Σ Ω Υ , ζ ↦→ ζ2 , (36)
which maps the boundary line {Reζ = 0, |ζ| < 1} into the portion of the boundary ] − 1, 0] ×
{0 − , 0 + }.
Similarly, the pair-of-pants Σ Λ Υ
≃
Figure 2: The pair-of-pants Σ Λ Υ .
can be described as the following quotient of a strip with a slit:
in the disjoint unionÊ×[−1, 0] ⊔Ê×[0, 1] we consider the identifications
(s, −1) ∼ (s, 0 − )
(s, 0 + ) ∼ (s, 1)
for s ≤ 0,
(s, 0 − ) ∼ (s, 0 + )
(s, −1) ∼ (s, 1)
for s ≥ 0.
See figure 2. This object is a Riemann surface without boundary, by considering the standard complex
structure at every point other than (0, 0) ∼ (0, −1) ∼ (0, 1), and by choosing the holomorphic
coordinate
�
ζ ∈�||ζ| < 1/ √ �
2 → Σ Λ ⎧
⎨
Υ, ζ ↦→
⎩
28
ζ 2 if Re ζ ≥ 0,
ζ 2 + i if Re ζ ≤ 0, Im ζ ≥ 0,
ζ 2 − i if Re ζ ≤ 0, Im ζ ≤ 0,
(37)