PDF (1016 kB)

M into Λ(M) as the space of constant loops. More information about the loop product and about
its relationship with the Pontrjagin and the intersection product are recalled in section 1. Note
that, we also obtain immediately in an anologous way a coproduct of degree −n by composing
e∗ ◦Γ! which corresponds to the pair-of-pants coproduct on Floer homology. It is easy to see, that
this coproduct is almost entirely trivial except for homology class of dimension n, see [AS08]
Actually, the analogy between the pair-of-pants product and the loop product is even deeper.
Indeed, we may look at the solutions (x1, x2) : [0, 1] → T ∗ M × T ∗ M of the following pair of
Hamiltonian systems
coupled by the non-local boundary condition
x ′ 1(t) = XH1(t, x1(t)), x ′ 2(t) = XH2(t, x1(2)), (5)
q1(0) = q1(1) = q2(0) = q2(1),
p1(1) − p1(0) = p2(0) − p2(1).
Here we are using the notation xj(t) = (qj(t), pj(t)), with qj(t) ∈ M and pj(t) ∈ T ∗ qj(t) M, for j =
1, 2. By studying the corresponding Lagrangian boundary value Cauchy-Riemann type problem
on the stripÊ×[0, 1], we obtain a chain complex, the Floer complex for figure-8 loops (F Θ (H), ∂),
on the graded free Abelian group generated by solutions of (5)-(6). Then we can show that:
(i) The homology of the chain complex (F Θ (H), ∂) is isomorphic to the singular homology of
Θ(M).
(ii) The pair of pants product factors through the homology of this chain complex.
(iii) The first homomorphism in this factorization corresponds to the homomorphism e!◦×, while
the second one corresponds to homomorphism Γ∗.
We also show that similar results hold for the space of based loops. The Hamiltonian problem
in this case is the equation (1) for x = (q, p) : [0, 1] → T ∗ M with boundary conditions
q(0) = q(1) = q0,
for a fixed q0 ∈ M. The corresponding Floer homology HF Ω ∗ (T ∗ M) is isomorphic to the singular
homology of the based loop space Ω(M), and there is a product on such a Floer homology, the
triangle product, which corresponds to the classical Pontrjagin product # on H∗(Ω(M)). Actually,
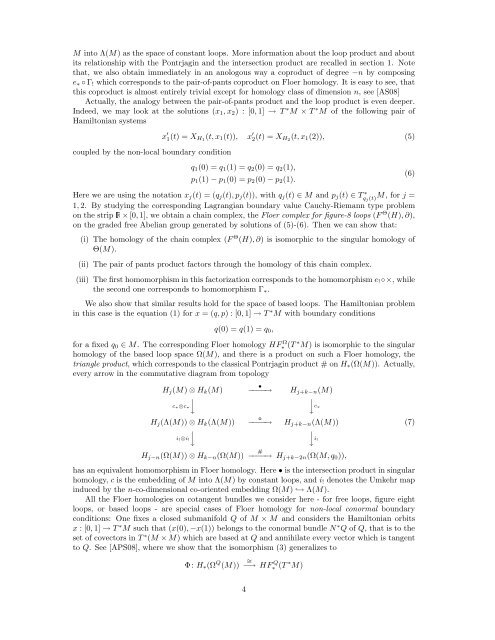
every arrow in the commutative diagram from topology
Hj(M) ⊗ Hk(M)
⏐
c∗⊗c∗�
Hj(Λ(M)) ⊗ Hk(Λ(M))
⏐
i!⊗i! �
Hj−n(Ω(M)) ⊗ Hk−n(Ω(M))
•
−−−−→ Hj+k−n(M)
o
⏐
� c∗
−−−−→ Hj+k−n(Λ(M))
⏐
�i!
#
−−−−→ Hj+k−2n(Ω(M, q0)),
has an equivalent homomorphism in Floer homology. Here • is the intersection product in singular
homology, c is the embedding of M into Λ(M) by constant loops, and i! denotes the Umkehr map
induced by the n-co-dimensional co-oriented embedding Ω(M) ֒→ Λ(M).
All the Floer homologies on cotangent bundles we consider here - for free loops, figure eight
loops, or based loops - are special cases of Floer homology for non-local conormal boundary
conditions: One fixes a closed submanifold Q of M × M and considers the Hamiltonian orbits
x : [0, 1] → T ∗ M such that (x(0), −x(1)) belongs to the conormal bundle N ∗ Q of Q, that is to the
set of covectors in T ∗ (M × M) which are based at Q and annihilate every vector which is tangent
to Q. See [APS08], where we show that the isomorphism (3) generalizes to
Φ: H∗(Ω Q (M)) ∼ =
−→ HF Q ∗ (T ∗ M)
4
(6)
(7)