Boundary Lyer Theory

80
I\'. Crnrtnl proprrtirs of the Nnvier Stnlzcs rqr~ntions
f. Mntlwn~nticnl illctntrntion of tltc process of goirlg to the limit R 4 oo t
Let IU rotinitlrr t.lie tlnmpr(l vihrntious of n point-mass tlmrrihctl hy t,hc tlifirrnlinl cr(~~nt,ion
Herc irr donolrn the vihrnling mnsn, c (.lie spring c:or~ntnnt., k I.l)c. tlnniping f:wto~.. r t.I~t: Irng(.ll
roordinnt.~ nlcrlmcrccl from t,lw jiosit,ion of ril~~ilibrin~n. nnrl I t.lw ti~nr. '1'11r initial ron(lilions arc
ILRRIIII~~C~ t,o be
r-O at 1-0. (4.14)
In ruinlogy with (.tie Nnvier-Stnkon eqr~ntions for t.lie cnse \rhcn thr lrinrnintic visronity, I*, is very
sninll, we condcr hcrr tlw limitsing rnsc of vrry smnll mnss nr, hrrn~~nr this loo rnrlnrs 1.11~ lerm
of thc Iiigllest ordrr in cqn. (4.13) to brromt: very small.
l'lir complrtr solrition of cqn. (4.13) s~~hjrrt tn the initinl rondit.ion (4.14) hns the form
x = A {exp ( I )
-- cxp (-k 11iri)): irr -t 0, (4.15)
where A in n frre constnnt \vl~osc v11111e rnn hr (Irtrrn~inr(l with rrfcrct~cr to 11 srron(l initinl contlit,ion.
If we put, in - 0 in eqn. (4.13), we nrc lrtl to t.lw simplified rqr~t~t.ion
wiiirli is of first orckr, nnrl whose solrclion is
d x
k- f e:r =0,
tlt
TO(/) = A rxp ( - ctlk). (4.17)
This solrrtion is idcnt,irnl wit,h the first term of the aomplctc solr~tion dur to the feliritous choice
of t.lw ndjuntnhle co~~utnnt,. However. this solution rnnnot he ~nntlc t.o satisfy t,lie init,iol coridit.ion
(4.14); it thus reprc~entR a eolut,ion for 1n.rge values of thr time, t ( Lco~~l~r" so111t.ion). 'l'hr8oIntion
for smnll vnlrtcs of time ("inner" solt~tion) snlisfies n.noLhrr diflerentinl equation \rliirlt can also
be dnrivwl from eqn. (4.13). 111 order to nchicw this, t.hr in~lopcntlrnt vnrinl~lr: t is "stret.cllcd"
in t,hnt a now "inner" vnrinhle
iv int,roduccd. 111 this manner, cqn. (4.13) is Ira~~sformetl t,o
wliicl~ p)vrrnn ll~r "innrr" ~ol~~t~ion. WII: soI111io11 now is
I
1.1 (I*) = A, rxp ( - kt*) 1 A,.
t* = t/m (4.18)
t 1 nln i~~dcl~f.cvl 1.0 Profrssor Klnns Grrnten for (I1c rosisrtl vrrnion of t,liin section.
* 1,. I'r~l.wlt.l, Annrhnr~liche 1t11t1 ~~wtzlirhc hlnt,henintik. I,rrt,ures drlivrrrd nt. (;oet,t.ingrn Univrrnil.y
ill t.hr \Yint.rr-Srmcnt,rr of 1!):11/:12.
f. Mnt,lremnticnl illust,ration of the procens of going t.o the limit R -t m 8 1
In sl>il.c of thn sirnl~lificatiorl, Il~e diflixentinl rqrrnt,ion (4.20) is onr of noco~irl ~lcgrrr: it c.nn 1)r
mntlc? t.o e:rt.idy LIIO initial rondit,iot~ (4.14) hy t.1~ clmirr
P 7
Il~c vnll~c of const.nnt. Az folloaw froin t.110 tnnl.ol~i~ip; to t11c "o~~lrr" nnl~~t,io~~, rt111. (4.17). 111 1111
ovrrlnpping rnngr, tht. is for ~noclcr:i(c vnlt~rn of tiinr, t.hc nol~~lionn in nqnn. (4.17) i ~nd (4.21)
nit~nl. ngrr:r. 'l'lit~s \VO tnr~sl, II~v~~
or, in wortln: 'l'h "or~t,nr" limit of t.lic "innrr" solntion IR~,
"outer" solnlion. Condition (4.23) Icntls nt, oncc to
he C~IIRI lo the "innt:r" lin~il 01 thr
nntl no to the innrr solrltion
.rr(t*) = A (1 - cxp (-- kt*)}. (4.25)
'I'l~c snme form rnn be obt.ninrtl from Lllr ron~plclr soI11t icm fro111 rqn. (4.15) by r*x~~:~n(ling (It(.
tirut t,rrni for small vnlws of I nnd rctni~~i~lg the GrnL tern1 only, Ihnt is by p~t,ling
7'11~ t\vo iic!ution~, tlic onter so111tion from eqn. (4.17) nrid Ll~c inner ~olution from rqn (4.26).
togct,l~er form the m!!iplcte solution on condition tlint cnrh is 118rtl ill its projwr I.III~~C of vnlidity.
ht finite 1, cqu. (4.15) tends (c the outer solut~ion for nt + 0. whcrens at constant t* eqn. (4.15)
tol~tl~ t,o the inner nolution. 'l'lle pnrtinl solut,io~is give I llc cornplrtc, cotnposit.~ nol~~t.ion which is
vnlitl in the cnl,ire rnnge of vni~cs of t i)y ridding thrnl togrtl~rr, rcmemhrring that Ihr ronlmon
tcr111 from eqn (4.23) ~nnst. he included only once, tlint, in sul~t,r:rclrrl from the R I nr.rorrling to
tho prcsrription
x(1) = ~"(1) + rt(t*) - Iim x: (l*) = TO (t) I rt(t*) -- lim xn(1). (4.27)
I* -. m 1 -. tJ
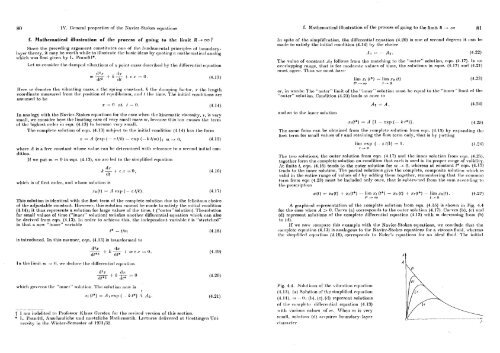
A graphical roprencntation of the complete .soIr~t,ion from eqn. (4.15) i~ nhown in Fig. 4.4
for the cnse when A > 0. Curve (a) corresponds to t,l~e outer solution (4.17). Cnrvcs (I)), (r) nntl
(d) represent solutions of the cotnplct,~ tlifirrntinl equation (4.13) \vitlt vr clrrrmsir~g from (h)
to ((I).
If wc now cor~ipnrc this rxamplo with thr Navier-St,okcs cq~mt,ions, we COIICIU~O
l.liat. t.Iie
r.o~nplctc cqrt:~t ion (4.13) is nn:~Iogonn 1.0 thr Nnvicr-Stokes cq11a1.ions for n vi~oonn Iluicl. wlwrms
Ilw sirnpIiliv(1 tqwtt,io~~ (4.1(;), t!orrcs~~on~ls In lh~lcr's rquntiom for nu i(lral ll~tid. WIP i11iti:11
Fig. 4.4. SOIIII~OIIS of thr viOr:itio~~ rq~~:iti(~n
( t . I:!). (a) Sol111io11 of tl~e sin~plifitd rqn:~th~
(s!. 14). 111 -- 0: (11). (c), ((I) rcprrsent so111tions
of 111r vo111111rt(: tlil'li~rcnt in1 cquntion (4.13)
\I it11 vnriol~s V:IIIIIY of i11. JVhcn irl is wry
s~nnll. soI111io11 ((I) arq~~irrn I~nun(l:tr,y- layer
141:1rnrtrr