The construction of the wonderful canon of logarithms
The construction of the wonderful canon of logarithms
The construction of the wonderful canon of logarithms
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
;<br />
Construction <strong>of</strong> <strong>the</strong> Canon, 41<br />
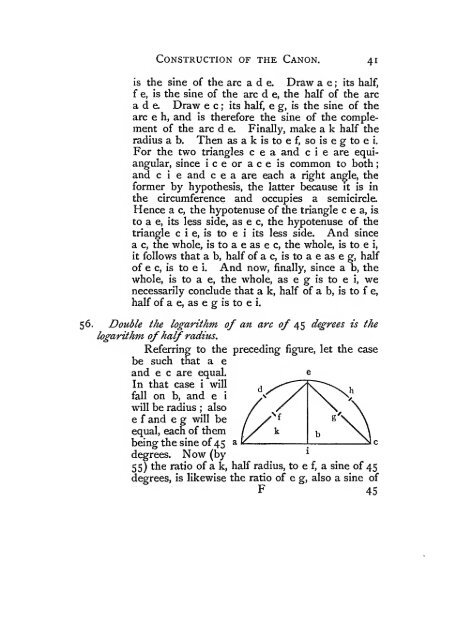
is <strong>the</strong> sine <strong>of</strong> <strong>the</strong> arc a d e. Draw a e ; its half,<br />
f e, is <strong>the</strong> sine <strong>of</strong> <strong>the</strong> arc d e, <strong>the</strong> half <strong>of</strong> <strong>the</strong> arc<br />
a d e. Draw e c ; its half, e g, is <strong>the</strong> sine <strong>of</strong> <strong>the</strong><br />
arc e h, and is <strong>the</strong>refore <strong>the</strong> sine <strong>of</strong> <strong>the</strong> complement<br />
<strong>of</strong> <strong>the</strong> arc d e. Finally, make a k half <strong>the</strong><br />
radius a b. <strong>The</strong>n as a k is to e f, so is e g to e i.<br />
For <strong>the</strong> two triangles c e a and c i e are equiangular,<br />
since i c e or a c e is common to both<br />
and c i e and c e a are each a right angle, <strong>the</strong><br />
former by hypo<strong>the</strong>sis, <strong>the</strong> latter because it is in<br />
<strong>the</strong> circumference and occupies a semicircle.<br />
Hence a c, <strong>the</strong> hypotenuse <strong>of</strong> <strong>the</strong> triangle c e a, is<br />
to a e, its less side, as e c, <strong>the</strong> hypotenuse <strong>of</strong> <strong>the</strong><br />
triangle c i e, is to e i its less side. And since<br />
a c, <strong>the</strong> whole, is to a e as e c, <strong>the</strong> whole, is to e i,<br />
it follows that a b, half <strong>of</strong> a c, is to a e as e g, half<br />
<strong>of</strong> e c, is to e i. And now, finally, since a b, <strong>the</strong><br />
whole, is to a e, <strong>the</strong> whole, as e g is to e i, we<br />
necessarily conclude that a k, half <strong>of</strong> a b, is to f e,<br />
half <strong>of</strong> a e, as e g is to e i.<br />
56. Double <strong>the</strong> logarithm <strong>of</strong> an arc <strong>of</strong> /^^ degrees is <strong>the</strong><br />
logarithm <strong>of</strong> half radius.<br />
Referring to <strong>the</strong> preceding figure, let <strong>the</strong> case<br />
be such that a e<br />
and e c are equal.<br />
e<br />
In that case i will<br />
fall on b, and e i<br />
will be radius ; also<br />
e f and e g will be<br />
equal, each <strong>of</strong> <strong>the</strong>m<br />
being <strong>the</strong> sine <strong>of</strong> 45<br />
*<br />
degrees. Now (by<br />
55) <strong>the</strong> ratio <strong>of</strong> a k, half radius, to e f, a sine <strong>of</strong> 45<br />
degrees, is likewise <strong>the</strong> ratio <strong>of</strong> e g, also a sine <strong>of</strong><br />
F 45











!['eries enti\`eres (+ [D78 Th d'Abel angulaire])](https://img.yumpu.com/14067031/1/184x260/eries-entieres-d78-th-dabel-angulaire.jpg?quality=85)

