Itinerant Spin Dynamics in Structures of ... - Jacobs University

Chapter 3: WL/WAL Crossover and Spin Relaxation in Confined Systems 41
Comparing Eqs. (3.49) and Eq.(3.66), we see that diagonalization leads to Eqs. (3.50)-
(3.52).
Influence of Dresselhaus SOC
It can be seen from Eqs. (3.58) and (3.59) that in the case of a homogeneous
Rashba field, the spin-density has a finite decay rate as we pointed out in Sec.2.3.4. However,
if we go beyond the pureRashba system and include a linear Dresselhaus coupling, the
first term in Eq.(3.33), we can find spin states which do not relax and are thus persistent.
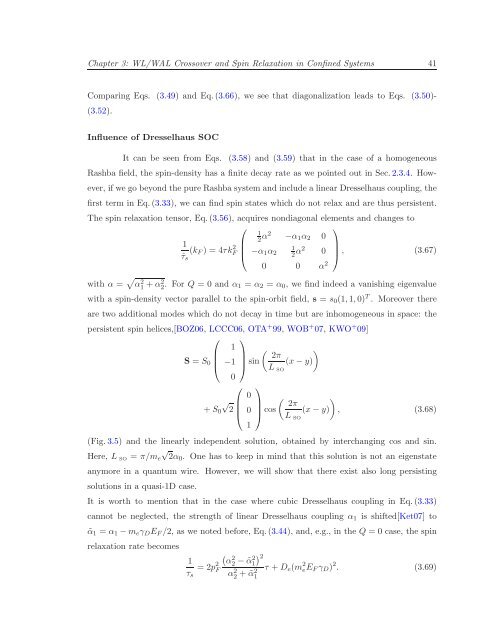
The spin relaxation tensor, Eq.(3.56), acquires nondiagonal elements and changes to
⎛ ⎞
1
2
1
(k F ) = 4τkF
2 α2 −α 1 α 2 0
⎜ 1
ˆτ s
⎝ −α 1 α 2 2 α2 0 ⎟
⎠ , (3.67)
0 0 α 2
with α = √ α 2 1 +α2 2 . For Q = 0 and α 1 = α 2 = α 0 , we find indeed a vanishing eigenvalue
with a spin-density vector parallel to the spin-orbit field, s = s 0 (1,1,0) T . Moreover there
are two additional modes which do not decay in time but are inhomogeneous in space: the
persistent spin helices,[BOZ06, LCCC06, OTA + 99, WOB + 07, KWO + 09]
⎛ ⎞
1 ( )
S = S 0
⎜
⎝ −1 ⎟ 2π
⎠ sin (x−y)
L SO
0
⎛ ⎞
0 ( )
√ +S 0 2 ⎜
⎝ 0 ⎟ 2π
⎠ cos (x−y) , (3.68)
L SO
1
(Fig.3.5) and the linearly independent solution, obtained by interchanging cos and sin.
Here, L SO = π/m e
√
2α0 . One has to keep in mind that this solution is not an eigenstate
anymore in a quantum wire. However, we will show that there exist also long persisting
solutions in a quasi-1D case.
It is worth to mention that in the case where cubic Dresselhaus coupling in Eq.(3.33)
cannot be neglected, the strength of linear Dresselhaus coupling α 1 is shifted[Ket07] to
˜α 1 = α 1 −m e γ D E F /2, as we noted before, Eq.(3.44), and, e.g., in the Q = 0 case, the spin
relaxation rate becomes
(
1 α
= 2p 2 2
2 − ˜α 2 2
1)
F
τ s α 2 2 + τ +D e (m 2 eE F γ D ) 2 . (3.69)
˜α2 1