103 Trigonometry Problems

̸
172 103 Trigonometry Problems
(|AI|+|DI|) 2 + (|BI|+|CI|) 2 = (|AB|+|CD|) 2 .
Prove that ABCD is an isosceles trapezoid.
Note: We introduce two trigonometric solutions and a synthetic-geometric
solution. The first solution, by Oleg Golberg, is very technical. The second solution,
by Tiankai Liu and Tony Zhang, reveals more geometrical background
in their computations. This is by far the most challenging problem in the US-
AMO 2004. There were only four complete solutions. The fourth student is
Jacob Tsimerman, from Canada. Evidently, these four students placed top four
in the contest. There were nine IMO gold medals won by these four students,
with each of Oleg and Tiankai winning three, Jacob two, and Tony one. Oleg
won his first two representing Russia, and the third representing the United
States. Jacob is one of only four students who achieved a perfect score at the
IMO 2004 in Athens, Greece.
The key is to recognize that the given identity is a combination of equality
cases of certain inequalities. By equal tangents, we have |AB| +|CD| =
|AD|+|BC| if and only if ABCD has an incenter. We will prove that for a
convex quadrilateral ABCD with incenter I, then
(|AI|+|DI|) 2 +(|BI|+|CI|) 2 ≤ (|AB|+|CD|) 2 = (|AD|+|BC|) 2 .(∗)
Equality holds if and only if AD ‖ BC and |AB| =|CD|. Without loss of
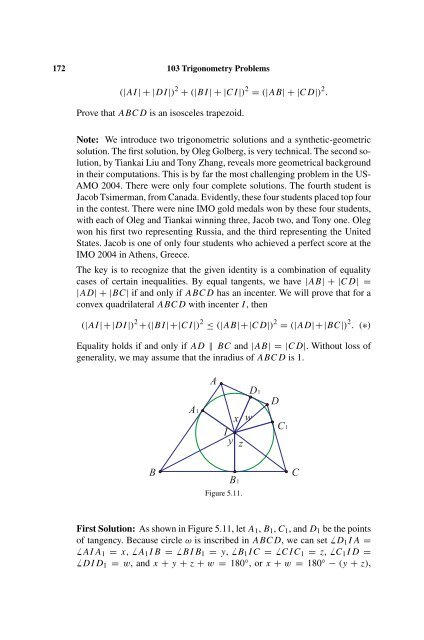
generality, we may assume that the inradius of ABCD is 1.
A1
A
x w
I
y z
D1
D
C1
B
B1
Figure 5.11.
C
First Solution: As shown in Figure 5.11, let A 1 , B 1 , C 1 , and D 1 be the points
of tangency. Because circle ω is inscribed in ABCD, we can set ̸ D 1 IA =
̸ AIA 1 = x, ̸ A 1 IB = ̸ BIB 1 = y, ̸ B 1 IC = ̸ CIC 1 = z, ̸ C 1 ID =
DID 1 = w, and x + y + z + w = 180 ◦ ,orx + w = 180 ◦ − (y + z),