103 Trigonometry Problems

1. Trigonometric Fundamentals 11
For a point A in the plane, we can also describe the position of A (relative to the
origin) by the distance r =|OA| and standard angle θ formed by the line OA and
the x axis. These coordinates are called polar coordinates, and they are written in
the form A = (r, θ). (Note that the polar coordinates of a point are not unique.)
In general, for any angle θ, we define the values of sin θ and cos θ as the coordinates
of points on the unit circle. Indeed, for any θ, there is a unique point A = (x 0 ,y 0 )
(in rectangular coordinates) on the unit circle ω such that A = (1,θ) (in polar
coordinates). We define cos θ = x 0 and sin θ = y 0 ; that is, A = (cos θ,sin θ) if and
only if A = (1,θ)in polar coordinates.
From the definition of the sine and cosine functions, it is clear that for all integers
k, sin(θ + k · 360 ◦ ) = sin θ and cos(θ + k · 360 ◦ ) = cos θ; that is, they are periodic
functions with period 360 ◦ .Forθ ̸= (2k + 1) · 90 ◦ , we define tan θ =
cos sin θ
θ ; and
for θ ̸= k · 180 ◦ , we define cot θ = cos sin θ θ . It is not difficult to see that tan θ is equal
to the slope of a line that forms a standard angle of θ with the x axis.
A
O
y
x
D
C2
O
y
x
B
A
C1
E
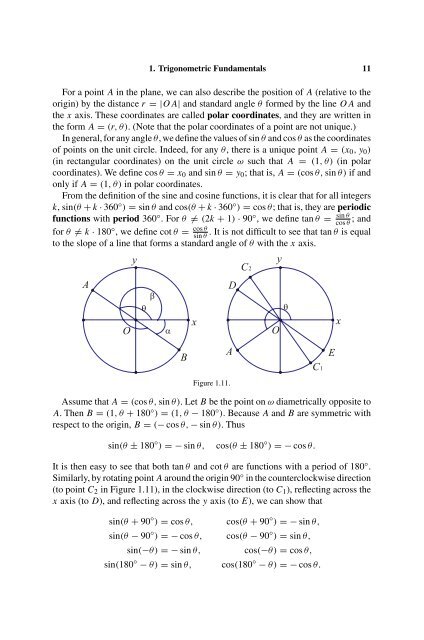
Figure 1.11.
Assume that A = (cos θ,sin θ). Let B be the point on ω diametrically opposite to
A. Then B = (1,θ + 180 ◦ ) = (1,θ − 180 ◦ ). Because A and B are symmetric with
respect to the origin, B = (− cos θ,− sin θ). Thus
sin(θ ± 180 ◦ ) =−sin θ, cos(θ ± 180 ◦ ) =−cos θ.
It is then easy to see that both tan θ and cot θ are functions with a period of 180 ◦ .
Similarly, by rotating point A around the origin 90 ◦ in the counterclockwise direction
(to point C 2 in Figure 1.11), in the clockwise direction (to C 1 ), reflecting across the
x axis (to D), and reflecting across the y axis (to E), we can show that
sin(θ + 90 ◦ ) = cos θ, cos(θ + 90 ◦ ) =−sin θ,
sin(θ − 90 ◦ ) =−cos θ, cos(θ − 90 ◦ ) = sin θ,
sin(−θ) =−sin θ, cos(−θ) = cos θ,
sin(180 ◦ − θ) = sin θ, cos(180 ◦ − θ) =−cos θ.