103 Trigonometry Problems
103 Trigonometry Problems
103 Trigonometry Problems
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
30 <strong>103</strong> <strong>Trigonometry</strong> <strong>Problems</strong><br />
A<br />
F<br />
P<br />
E<br />
B<br />
D1<br />
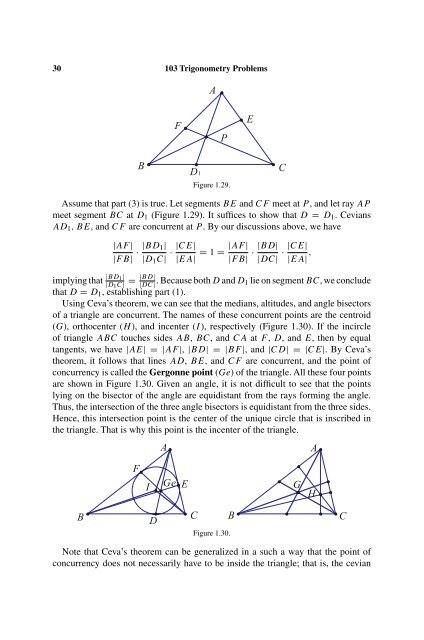
Figure 1.29.<br />
C<br />
Assume that part (3) is true. Let segments BE and CF meet at P , and let ray AP<br />
meet segment BC at D 1 (Figure 1.29). It suffices to show that D = D 1 . Cevians<br />
AD 1 ,BE, and CF are concurrent at P . By our discussions above, we have<br />
|AF |<br />
|FB| · |BD 1|<br />
|D 1 C| · |CE|<br />
|EA| = 1 = |AF |<br />
|FB| · |BD|<br />
|DC| · |CE|<br />
|EA| ,<br />
implying that |BD 1|<br />
|D 1 C| = |BD|<br />
|DC| . Because both D and D 1 lie on segment BC, we conclude<br />
that D = D 1 , establishing part (1).<br />
Using Ceva’s theorem, we can see that the medians, altitudes, and angle bisectors<br />
of a triangle are concurrent. The names of these concurrent points are the centroid<br />
(G), orthocenter (H ), and incenter (I), respectively (Figure 1.30). If the incircle<br />
of triangle ABC touches sides AB, BC, and CA at F , D, and E, then by equal<br />
tangents, we have |AE| =|AF |, |BD| =|BF|, and |CD| =|CE|. ByCeva’s<br />
theorem, it follows that lines AD, BE, and CF are concurrent, and the point of<br />
concurrency is called the Gergonne point (Ge) of the triangle. All these four points<br />
are shown in Figure 1.30. Given an angle, it is not difficult to see that the points<br />
lying on the bisector of the angle are equidistant from the rays forming the angle.<br />
Thus, the intersection of the three angle bisectors is equidistant from the three sides.<br />
Hence, this intersection point is the center of the unique circle that is inscribed in<br />
the triangle. That is why this point is the incenter of the triangle.<br />
A<br />
A<br />
F<br />
I<br />
Ge<br />
E<br />
G<br />
H<br />
B<br />
D<br />
C<br />
Figure 1.30.<br />
B<br />
C<br />
Note that Ceva’s theorem can be generalized in a such a way that the point of<br />
concurrency does not necessarily have to be inside the triangle; that is, the cevian