103 Trigonometry Problems
103 Trigonometry Problems
103 Trigonometry Problems
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
176 <strong>103</strong> <strong>Trigonometry</strong> <strong>Problems</strong><br />
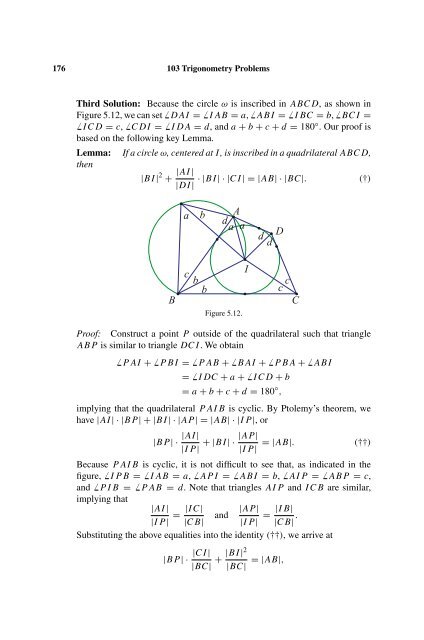
Third Solution: Because the circle ω is inscribed in ABCD, as shown in<br />
Figure 5.12, we can set ̸ DAI = ̸ IAB = a, ̸ ABI = ̸ IBC = b, ̸ BCI =<br />
̸ ICD = c, ̸ CDI = ̸ IDA = d, and a + b + c + d = 180 ◦ . Our proof is<br />
based on the following key Lemma.<br />
Lemma: If a circle ω, centered at I, is inscribed in a quadrilateral ABCD,<br />
then<br />
|BI| 2 + |AI| ·|BI|·|CI|=|AB|·|BC|. (†)<br />
|DI|<br />
a<br />
b<br />
A<br />
d<br />
a a<br />
d<br />
D<br />
d<br />
B<br />
c<br />
I<br />
b<br />
b<br />
Figure 5.12.<br />
c<br />
c<br />
C<br />
Proof: Construct a point P outside of the quadrilateral such that triangle<br />
ABP is similar to triangle DCI. We obtain<br />
̸ PAI + ̸ PBI = ̸ PAB + ̸ BAI + ̸ PBA+ ̸ ABI<br />
= ̸ IDC + a + ̸ ICD + b<br />
= a + b + c + d = 180 ◦ ,<br />
implying that the quadrilateral PAIB is cyclic. By Ptolemy’s theorem, we<br />
have |AI|·|BP|+|BI|·|AP |=|AB|·|IP|, or<br />
|BP|· |AI| +|BI|·|AP | =|AB|. (††)<br />
|IP| |IP|<br />
Because PAIB is cyclic, it is not difficult to see that, as indicated in the<br />
figure, ̸ IPB = ̸ IAB = a, ̸ AP I = ̸ ABI = b, ̸ AIP = ̸ ABP = c,<br />
and ̸ PIB = ̸ PAB = d. Note that triangles AIP and ICB are similar,<br />
implying that<br />
|AI|<br />
|IP| = |IC| |AP |<br />
and<br />
|CB| |IP| = |IB|<br />
|CB| .<br />
Substituting the above equalities into the identity (††), we arrive at<br />
|BP|· |CI|<br />
|BC| + |BI|2<br />
|BC| =|AB|,