Michael Corral: Vector Calculus

122 CHAPTER 3. MULTIPLE INTEGRALS
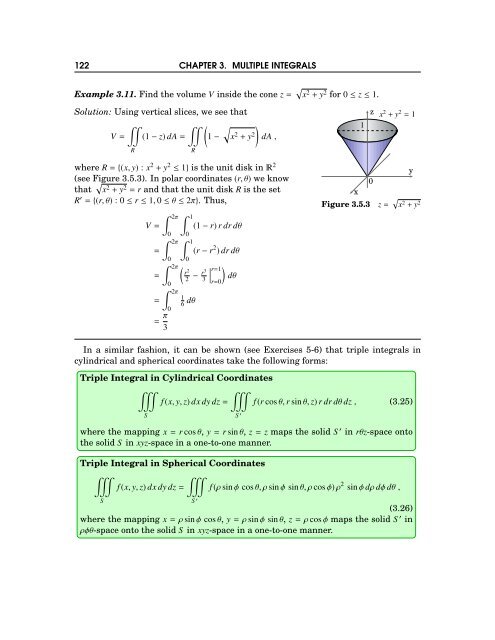
Example 3.11. Find the volume V inside the cone z= √ x 2 +y 2 for 0≤z≤1.
Solution: Using vertical slices, we see that
( )
V= (1−z)dA= 1−
√x 2 +y 2 dA,
1
z
x 2 +y 2 = 1
R
R
where R={(x,y) : x 2 +y 2 ≤ 1} is the unit disk in 2
(see Figure 3.5.3). In polar coordinates (r,θ) we know
that √ x 2 +y 2 = r and that the unit disk R is the set
R ′ ={(r,θ) : 0≤r≤1,0≤θ≤2π}. Thus,
V=
=
=
=
∫ 2π ∫ 1
0 0
∫ 2π ∫ 1
0
∫ 2π
0
∫ 2π
0
0
(1−r)rdrdθ
(r−r 2 )drdθ
(
r 2 2 − r3 3
1
6 dθ
∣
∣ r=1
r=0
)
dθ
y
0
x
Figure 3.5.3 z= √ x 2 +y 2
= π 3
In a similar fashion, it can be shown (see Exercises 5-6) that triple integrals in
cylindrical and spherical coordinates take the following forms:
Triple Integral in Cylindrical Coordinates
f(x,y,z)dxdydz= f(rcosθ,rsinθ,z)rdrdθdz, (3.25)
S
S ′
where the mapping x=rcosθ, y=rsinθ, z=zmaps the solid S ′ in rθz-space onto
the solid S in xyz-space in a one-to-one manner.
Triple Integral in Spherical Coordinates
f(x,y,z)dxdydz= f(ρsinφ cosθ,ρsinφ sinθ,ρcosφ)ρ 2 sinφdρdφdθ,
S
S ′ (3.26)
where the mapping x=ρsinφ cosθ, y=ρsinφ sinθ, z=ρcosφ maps the solid S ′ in
ρφθ-space onto the solid S in xyz-space in a one-to-one manner.