Michael Corral: Vector Calculus

4.1 Line Integrals 137
whichyoumayrecognizefromSection1.9asthelengthofthecurveC overtheinterval
[a,t], for all t in [a,b]. That is,
√
ds= s ′ (t)dt= x ′ (t) 2 +y ′ (t) 2 dt, (4.7)
by the Fundamental Theorem of Calculus.
For a general real-valued function f(x,y), what does the line integral ∫ C f(x,y)ds
represent? The preceding discussion of ds gives us a clue. You can think of differentials
as infinitesimal lengths. So if you think of f(x,y) as the height of a picket fence
alongC, then f(x,y)ds can be thought of as approximately the area of a section of that
fence ∫ over some infinitesimally small section of the curve, and thus the line integral
f(x,y)ds is the total area of that picket fence (see Figure 4.1.2).
C
y
f(x,y)
C ds
x
0
Figure 4.1.2 Area of shaded rectangle=height×width≈ f(x,y)ds
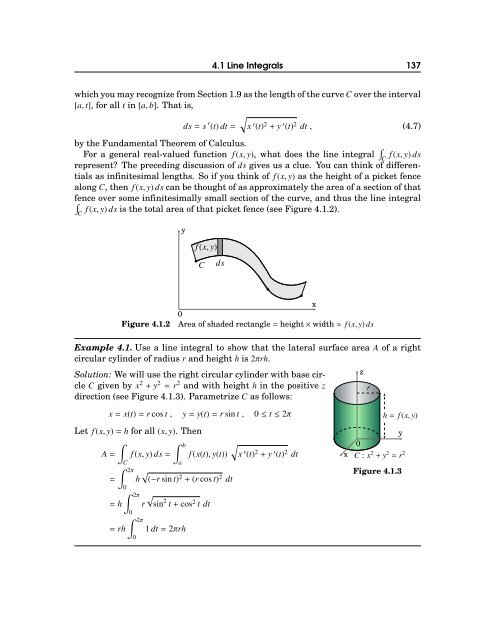
Example 4.1. Use a line integral to show that the lateral surface area A of a right
circular cylinder of radius r and height h is 2πrh.
Solution: We will use the right circular cylinder with base circle
C given by x 2 + y 2 = r 2 and with height h in the positive z
direction (see Figure 4.1.3). Parametrize C as follows:
z
r
x= x(t)=rcost, y=y(t)=rsint, 0≤t≤2π
Let f(x,y)=hfor all (x,y). Then
∫ ∫ b
A= f(x,y)ds= f(x(t),y(t))
=
= h
C
∫ 2π
= rh
0
∫ 2π
0
∫ 2π
0
a
h √ (−rsint) 2 +(rcost) 2 dt
√
r sin 2 t+cos 2 t dt
1dt=2πrh
√
x ′ (t) 2 +y ′ (t) 2 dt
x
h= f(x,y)
y
0
C : x 2 +y 2 = r 2
Figure 4.1.3