Michael Corral: Vector Calculus

140 CHAPTER 4. LINE AND SURFACE INTEGRALS
Theorem 4.1. For a vector field f(x,y)=P(x,y)i+Q(x,y)jand a curveC with a smooth
parametrization x= x(t), y=y(t), a≤t≤band position vector r(t)= x(t)i+y(t)j,
∫ ∫
f·dr= f·Tds, (4.15)
where T(t)= r′ (t)
‖r ′ (t)‖
is the unit tangent vector to C at (x(t),y(t)).
C
If the vector field f(x,y) represents the force moving an object along a curve C, then
the work W done by this force is
∫ ∫
W= f·Tds= f·dr. (4.16)
C
C
C
Example 4.2. Evaluate ∫ C (x2 +y 2 )dx+2xydy, where:
(a) C : x=t, y=2t, 0≤t≤1
(b) C : x=t, y=2t 2 , 0≤t≤1
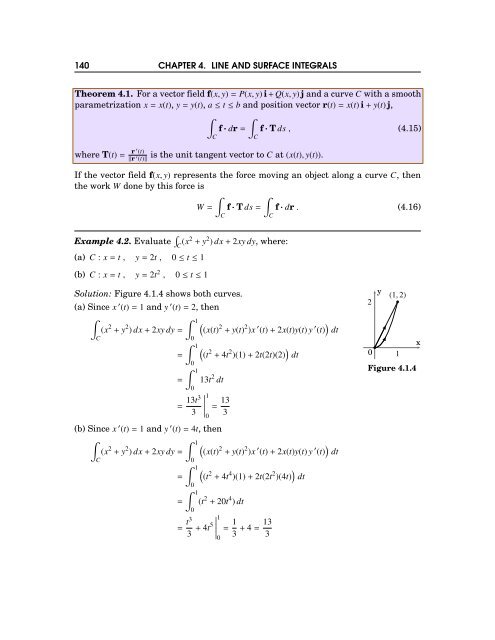
Solution: Figure 4.1.4 shows both curves.
(a) Since x ′ (t)=1and y ′ (t)=2, then
∫ ∫ 1 (
(x 2 +y 2 )dx+2xydy= (x(t) 2 +y(t) 2 )x ′ (t)+2x(t)y(t)y ′ (t) ) dt
C
=
=
0
∫ 1
0
∫ 1
0
= 13t3
3
(
(t 2 +4t 2 )(1)+2t(2t)(2) ) dt
13t 2 dt
∣
1
0
= 13 3
(b) Since x ′ (t)=1and y ′ (t)=4t, then
∫ ∫ 1 (
(x 2 +y 2 )dx+2xydy= (x(t) 2 +y(t) 2 )x ′ (t)+2x(t)y(t)y ′ (t) ) dt
C
=
=
0
∫ 1
0
∫ 1
0
(
(t 2 +4t 4 )(1)+2t(2t 2 )(4t) ) dt
(t 2 +20t 4 )dt
∣ ∣∣∣∣∣
= t3 1
3 +4t5 = 1 3 +4= 13 3
0
y
(1,2)
2
x
0 1
Figure 4.1.4