Michael Corral: Vector Calculus

172 CHAPTER 4. LINE AND SURFACE INTEGRALS
Note: The condition in Stokes’ Theorem that the surfaceΣhave a (continuously
varying) positive unit normal vector n and a boundary curveC traversed n-positively
can be expressed more precisely as follows: if r(t) is the position vector for C and
T(t)=r ′ (t)/‖r ′ (t)‖ is the unit tangent vector to C, then the vectors T, n, T×n form a
right-handed system.
Also, it should be noted that Stokes’ Theorem holds even when the boundary curve
C is piecewise smooth.
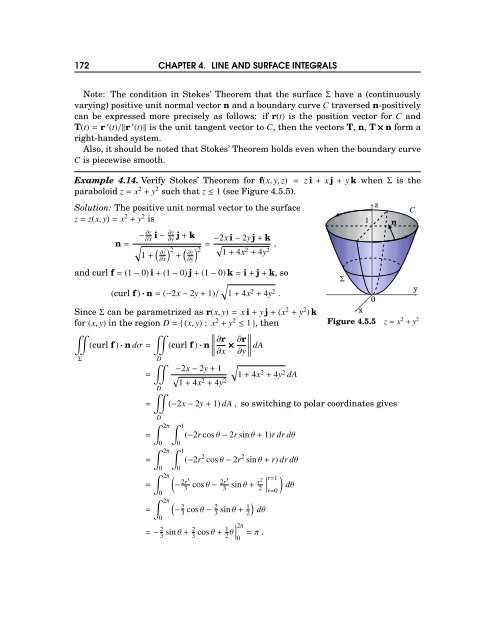
Example 4.14. Verify Stokes’ Theorem for f(x,y,z) = zi+ xj+yk whenΣis the
paraboloid z= x 2 +y 2 such that z≤1(see Figure 4.5.5).
Solution: The positive unit normal vector to the surface
z=z(x,y)= x 2 +y 2 is
n= −∂z ∂x i−∂z
√
1+ ( ∂z
∂x
∂y j+k
) 2+ ( ) = −2xi−2yj+k √
∂z
2
1+4x 2 +4y ,
2
∂y
and curl f=(1−0)i+(1−0)j+(1−0)k=i+j+k, so
√
(curl f)·n=(−2x−2y+1)/ 1+4x 2 +4y 2 .
SinceΣcan be parametrized as r(x,y)= xi+yj+(x 2 + y 2 )k
for (x,y) in the region D={(x,y) : x 2 +y 2 ≤ 1}, then
(curl f)·ndσ= (curl f)·n
∂r
∥∂x ×∂r
∂y∥ dA
Σ
D
−2x−2y+1
= √
√1+4x 2 +4y 2 dA
1+4x 2 +4y 2
D
D
∫ 2π ∫ 1
1
Σ
y
0
x
Figure 4.5.5 z= x 2 +y 2
= (−2x−2y+1)dA, so switching to polar coordinates gives
=
=
=
=
0 0
∫ 2π ∫ 1
0
∫ 2π
0
∫ 2π
0
0
(−2rcosθ−2rsinθ+1)rdrdθ
(−2r 2 cosθ−2r 2 sinθ+r)drdθ
(
− 2r3 2r3
3
cosθ−
3 sinθ+ r2 2
(
−
2
3 cosθ− 2 3 sinθ+ 1 2)
dθ
=− 2 3 sinθ+ 2 3 cosθ+ 1 2 θ ∣ ∣∣∣ 2π
0 =π.
∣
∣ r=1
r=0
)
dθ
z
n
C