Michael Corral: Vector Calculus

168 CHAPTER 4. LINE AND SURFACE INTEGRALS
∇F= f). So by Theorem 4.12 we know that
∫
f·dr=F(B)−F(A) , where A=(x(0),y(0),z(0)) and B=(x(8π),y(8π),z(8π)), so
C
= F(8πsin8π,8πcos8π,8π)−F(0sin0,0cos0,0)
= F(0,8π,8π)−F(0,0,0)
= 0+ (8π)2
2
+(8π) 2 −(0+0+0)=96π 2 .
WewillnowdiscussageneralizationofGreen’sTheoremin 2 toorientablesurfaces
in 3 , called Stokes’ Theorem. A surfaceΣin 3 is orientable if there is a continuous
vector field N in 3 such that N is nonzero and normal toΣ(i.e. perpendicular to the
tangent plane) at each point ofΣ. We say that such an N is a normal vector field.
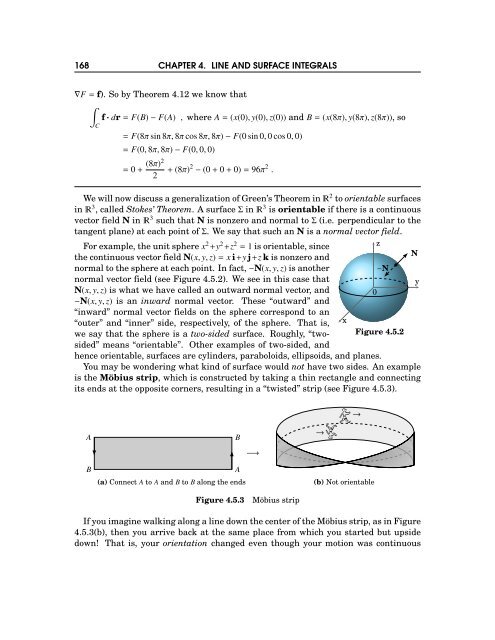
Forexample,theunitsphere x 2 +y 2 +z 2 = 1isorientable,since z
N
thecontinuousvectorfieldN(x,y,z)= xi+yj+zkisnonzeroand
normaltothesphereateachpoint. Infact,−N(x,y,z)isanother −N
normal vector field (see Figure 4.5.2). We see in this case that
y
N(x,y,z) is what we have called an outward normal vector, and 0
−N(x,y,z) is an inward normal vector. These “outward” and
“inward” normal vector fields on the sphere correspond to an
“outer” and “inner” side, respectively, of the sphere. That is, x
we say that the sphere is a two-sided surface. Roughly, “twosided”
means “orientable”. Other examples of two-sided, and
Figure 4.5.2
hence orientable, surfaces are cylinders, paraboloids, ellipsoids, and planes.
You may be wondering what kind of surface would not have two sides. An example
is the Möbius strip, which is constructed by taking a thin rectangle and connecting
its ends at the opposite corners, resulting in a “twisted” strip (see Figure 4.5.3).
A
B
(a) Connect A to A and B to B along the ends
B
A
−→
AA →
→
(b) Not orientable
Figure 4.5.3 Möbius strip
IfyouimaginewalkingalongalinedownthecenteroftheMöbiusstrip,asinFigure
4.5.3(b), then you arrive back at the same place from which you started but upside
down! That is, your orientation changed even though your motion was continuous