Lectures on String Theory

– 34 –
Orbits of the Virasoro algebra
+
Gauge condition for X
Constrained surface
_
L m =L m =0
Phase space (P,X)
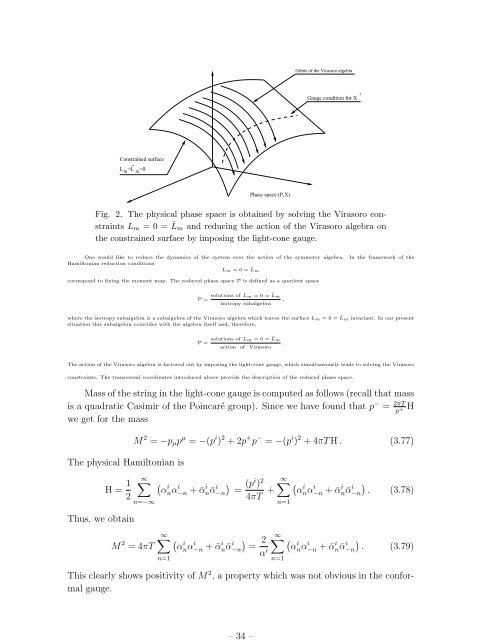
Fig. 2. The physical phase space is obtained by solving the Virasoro constraints
L m = 0 = ¯L m and reducing the action of the Virasoro algebra on
the constrained surface by imposing the light-cone gauge.
One would like to reduce the dynamics of the system over the action of the symmetry algebra.
Hamiltonian reduction conditions
L m = 0 = ¯L m
In the framework of the
correspond to fixing the moment map. The reduced phase space P is defined as a quotient space
P = solutions of L m = 0 = ¯L m
isotropy subalgebra
,
where the isotropy subalgebra is a subalgebra of the Virasoro algebra which leaves the surface L m = 0 = ¯L m invariant. In our present
situation this subalgebra coincides with the algebra itself and, therefore,
P = solutions of Lm = 0 = ¯L m
action of Virasoro
.
The action of the Virasoro algebra is factored out by imposing the light-cone gauge, which simultaneously leads to solving the Virasoro
constraints. The transversal coordinates introduced above provide the description of the reduced phase space.
Mass of the string in the light-cone gauge is computed as follows (recall that mass
is a quadratic Casimir of the Poincaré group). Since we have found that p − = 2πT H
p +
we get for the mass
The physical Hamiltonian is
H = 1 2
Thus, we obtain
M 2 = −p µ p µ = −(p i ) 2 + 2p + p − = −(p i ) 2 + 4πT H . (3.77)
∞∑
n=−∞
(
α
i
n α i −n + ᾱ i nᾱ i −n)
=
(p i ) 2
4πT + ∞
∑
n=1
( )
α
i
n α−n i + ᾱnᾱ i −n
i . (3.78)
M 2 = 4πT
∞∑
n=1
(
α
i
n α i −n + ᾱ i nᾱ i −n)
=
2
α ′
∞
∑
n=1
( )
α
i
n α−n i + ᾱnᾱ i −n
i . (3.79)
This clearly shows positivity of M 2 , a property which was not obvious in the conformal
gauge.