Lectures on String Theory

– 64 –
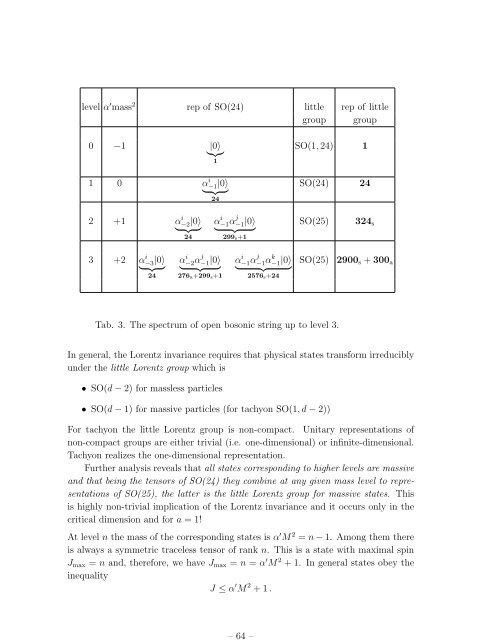
level α ′ mass 2 rep of SO(24) little rep of little
group group
0 −1 |0〉
}{{}
1
1 0 α−1|0〉
i
} {{ }
24
SO(1, 24) 1
SO(24) 24
2 +1 α−2|0〉
i
} {{ }
24
α i −1α j −1|0〉
} {{ }
299 s +1
SO(25)
324 s
3 +2 α−3|0〉
i
} {{ }
24
α i −2α j −1|0〉
} {{ }
276 a +299 s +1
α i −1α j −1α k −1|0〉
} {{ }
2576 s +24
SO(25)
2900 s + 300 a
Tab. 3. The spectrum of open bosonic string up to level 3.
In general, the Lorentz invariance requires that physical states transform irreducibly
under the little Lorentz group which is
• SO(d − 2) for massless particles
• SO(d − 1) for massive particles (for tachyon SO(1, d − 2))
For tachyon the little Lorentz group is non-compact. Unitary representations of
non-compact groups are either trivial (i.e. one-dimensional) or infinite-dimensional.
Tachyon realizes the one-dimensional representation.
Further analysis reveals that all states corresponding to higher levels are massive
and that being the tensors of SO(24) they combine at any given mass level to representations
of SO(25), the latter is the little Lorentz group for massive states. This
is highly non-trivial implication of the Lorentz invariance and it occurs only in the
critical dimension and for a = 1!
At level n the mass of the corresponding states is α ′ M 2 = n − 1. Among them there
is always a symmetric traceless tensor of rank n. This is a state with maximal spin
J max = n and, therefore, we have J max = n = α ′ M 2 + 1. In general states obey the
inequality
J ≤ α ′ M 2 + 1 .