Lectures on String Theory

More documents

Recommendations

Info

– 89 – i.e. the torus is characterized by one complex modulus τ. For g ≥ 2 there is theorem that states that the corresponding manifold always admits a metric with constant negative curvature. For n ≠ 0 this means that − 1 nR > 0 and, 2 therefore, dim ker∇ z (n) = 0. The Riemann surfaces with g ≥ 2 have no conformal isometries and by the Riemann-Roch theorem that means that the number of complex moduli n = 1 is 3g − 3. For n = 0 we have dim ker∇ (0) z = 1, because the corresponding kernel is spanned by constants. The Riemann-Roch theorem implies then that dim ker∇ z (1) − dim ker∇ (0) z = (2n + 1) (g − 1) = g − 1 , } {{ } } {{ } =1 n=0 i.e. dim ker∇ z (1) = g. The kernel of ∇z (1) is spanned by one forms ∇ z (1)ω z = h z¯z ¯∂ωz = 0 =⇒ ¯∂ω z = 0 . Thus we arrive at another important consequence of the Riemann-Roch theorem: on a Riemann surface of the genus g there exists precisely g linearly independent (globally defined) analytic one-forms. These analytic differential forms are called abelian differentials of the first kind. The information we obtained by using the Riemann-Roch theorem is summarized in the Table below. g dim ker∇ z (n) dim ker∇ z (n+1) 0 2n + 1 0 1 1 1 > 1 1 for n = 0 g 0 for n > 0 (2n + 1)(g − 1) Moduli space of tori Here we would like to look more closely at the moduli space which describes conformally non-equivalent tori – the Riemann surfaces of the genus g = 1. The torus can be obtained by performing the following identification on the complex plane z ≡ z + nλ 1 + mλ 2 , n, m ∈ Z , λ 1 , λ 2 ∈ C . The parameters λ 1,2 are subject to conformal transformations z → λz and, therefore, only their ratio τ = λ 2 λ 1 is scale-invariant. By using this freedom (the U(1)-rotation + real rescaling) one can always bring the parallelogram defining the torus upon
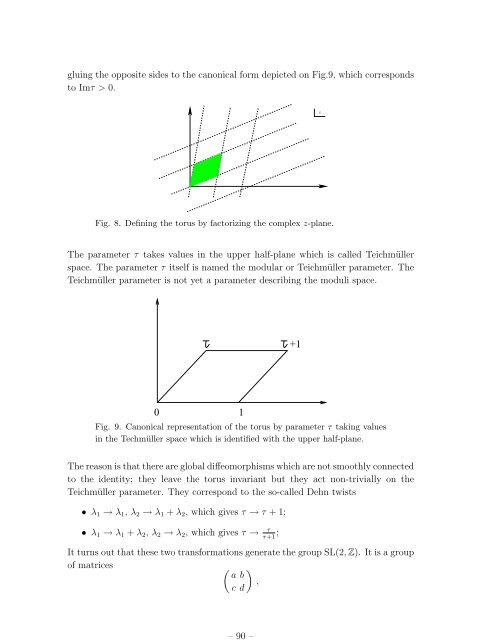
– 90 – gluing the opposite sides to the canonical form depicted on Fig.9, which corresponds to Imτ > 0. z ¡¡¡¡¡ ¢¡¢¡¢¡¢¡¢¡¢¡¢ ¡ ¡¡¡¡¡ ¢¡¢¡¢¡¢¡¢¡¢¡¢ ¡ ¡¡¡¡¡ ¢¡¢¡¢¡¢¡¢¡¢¡¢ ¡ ¡¡¡¡¡ ¢¡¢¡¢¡¢¡¢¡¢¡¢ ¡ ¡¡¡¡¡ ¢¡¢¡¢¡¢¡¢¡¢¡¢ ¡ ¡¡¡¡¡ ¢¡¢¡¢¡¢¡¢¡¢¡¢ ¡ ¡¡¡¡¡ ¢¡¢¡¢¡¢¡¢¡¢¡¢ ¡ ¡¡¡¡¡ ¢¡¢¡¢¡¢¡¢¡¢¡¢ ¡ ¡¡¡¡¡ ¢¡¢¡¢¡¢¡¢¡¢¡¢ ¡ ¡¡¡¡¡ ¢¡¢¡¢¡¢¡¢¡¢¡¢ ¡ ¡¡¡¡¡ ¢¡¢¡¢¡¢¡¢¡¢¡¢ ¡ ¡¡¡¡¡ ¢¡¢¡¢¡¢¡¢¡¢¡¢ ¡ ¡¡¡¡¡ ¢¡¢¡¢¡¢¡¢¡¢¡¢ ¡ ¡¡¡¡¡ ¢¡¢¡¢¡¢¡¢¡¢¡¢ ¡ Fig. 8. Defining the torus by factorizing the complex z-plane. The parameter τ takes values in the upper half-plane which is called Teichmüller space. The parameter τ itself is named the modular or Teichmüller parameter. The Teichmüller parameter is not yet a parameter describing the moduli space. +1 0 1 Fig. 9. Canonical representation of the torus by parameter τ taking values in the Techmüller space which is identified with the upper half-plane. The reason is that there are global diffeomorphisms which are not smoothly connected to the identity; they leave the torus invariant but they act non-trivially on the Teichmüller parameter. They correspond to the so-called Dehn twists • λ 1 → λ 1 , λ 2 → λ 1 + λ 2 , which gives τ → τ + 1; • λ 1 → λ 1 + λ 2 , λ 2 → λ 2 , which gives τ → τ ; τ+1 It turns out that these two transformations generate the group SL(2, Z). It is a group of matrices ( ) a b , c d
Page 1 and 2:
Preprint typeset in JHEP style - HY
Page 3 and 4:
- 2 - 6. Classical fermionic supers
Page 5 and 6:
- 4 - bosons and Ramond’s fermion
Page 7 and 8:
- 6 - ? Type I Type IIB E 8 x E 8 h
Page 9 and 10:
- 8 - 2. Relativistic particle Cons
Page 11 and 12:
- 10 - are called the first class c
Page 13 and 14:
- 12 - which is in complete agreeme
Page 15 and 16:
- 14 - The Nambu-Goto action ∫
Page 17 and 18:
- 16 - Exercise Show that the Hessi
Page 19 and 20:
- 18 - 3.2.3 Conformal gauge Consid
Page 21 and 22:
- 20 - which result into We see tha
Page 23 and 24:
- 22 - Analogously, {¯L m , X µ }
Page 25 and 26:
- 24 - 3.3.1 Solutions of the equat
Page 27 and 28:
- 26 - i.e. the total mass momentum
Page 29 and 30:
- 28 - It follows from the Schwarz
Page 31 and 32:
- 30 - 2. Varying w.r.t. γ τσ le
Page 33 and 34:
- 32 - The physical Hamiltonian and
Page 35 and 36:
- 34 - Orbits of the Virasoro algeb
Page 37 and 38:
- 36 - Let us outline the computati
Page 39 and 40: - 38 - Thus, we arrive at {l i− ,
Page 41 and 42: - 40 - One can correctly anticipate
Page 43 and 44: - 42 - Using α µ q α µ m+n−q
Page 45 and 46: - 44 - Here N is the number operato
Page 47 and 48: - 46 - string spectrum and makes it
Page 49 and 50: - 48 - Thus, for τ > τ ′ one ob
Page 51 and 52: where the expression on the r.h.s.
Page 53 and 54: - 52 - Plugging everything together
Page 55 and 56: - 54 - where we assume that τ 1 >
Page 57 and 58: - 56 - It also follows from the sca
Page 59 and 60: - 58 - 4.2 Quantization in the phys
Page 61 and 62: - 60 - Here by arrow we indicated t
Page 63 and 64: - 62 - Thus, our commutator takes t
Page 65 and 66: - 64 - level α ′ mass 2 rep of S
Page 67 and 68: - 66 - representations of the trans
Page 69 and 70: - 68 - Let us illustrate this proce
Page 71 and 72: - 70 - Here c α is called a ghost
Page 73 and 74: - 72 - The ghosts and anti-ghosts a
Page 75 and 76: - 74 - The expression in the bracke
Page 77 and 78: - 76 - One can check that these obj
Page 79 and 80: ¡ ¡ £¢ ¢ - 78 - from the cylin
Page 81 and 82: - 80 - coordinate chart ¡ ¢¡¢¡
Page 83 and 84: - 82 - The last formula can be unde
Page 85 and 86: - 84 - i.e the conformal factor φ
Page 87 and 88: - 86 - The last term here reflects
Page 89: - 88 - i.e. it is not normalizable.
Page 93 and 94: - 92 - Any point in the upper-half
Page 95 and 96: - 94 - d(d−1) 2 local Lorentz tra
Page 97 and 98: - 96 - The two-dimensional Dirac ma
Page 99 and 100: - 98 - The “flat” and “curved
Page 101 and 102: - 100 - Here D α ɛ = ∂ α ɛ
Page 103 and 104: - 102 - By using reparametrizations
Page 105 and 106: - 104 - Consider first the commutat
Page 107 and 108: - 106 - Now we note that in additio
Page 109 and 110: - 108 - One can also check that the
Page 111 and 112: - 110 - The oscillator ground state
Page 113 and 114: - 112 - and similar for ML 2 . For
Page 115 and 116: - 114 - real components. Thus, the
Page 117 and 118: - 116 - we get Since a general stat
Page 119 and 120: - 118 - that of Type IIB supergravi
Page 121 and 122: - 120 - In this form the Hamiltonia
Page 123 and 124: - 122 - group” was introduced by
Page 125 and 126: - 124 - where the operator Q k (x,
Page 127 and 128: - 126 - where we have substituted
Page 129 and 130: - 128 - D. Riemann normal coordinat
Page 131 and 132: - 130 - E. Exercises Exercise 1. Sh
Page 133 and 134: - 132 - Exercise 10. • Show that
Page 135 and 136: - 134 - Exercise 16. Prove that tha
Page 137 and 138: - 136 - where A(m) is a function of
Page 139 and 140: - 138 - Exercise 38. Compute the th
Page 141 and 142:
- 140 - as Here Exercise 50. Under
show all

Lectures on String Theory

You also want an ePaper? Increase the reach of your titles

Delete template?

Save as template?