Lectures on String Theory

– 77 –
5. Geometry and topology of string world-sheet
5.1 From Lorentzian to Euclidean world-sheets
String world-sheets and the target space both have Lorentzian signature. The connection
to the theory of Riemann surfaces can be made by performing the Wick
rotation of both world-sheet and the target space metrics. In particular, for the
world-sheet time τ this means τ → −iτ. Thus, on the string-world sheet this Euclidean
continuation corresponds to
σ ± = τ ± σ → −i(τ ± iσ) . (5.1)
A word of caution is needed here: One cannot really prove that the theory of the
Lorentzian world-sheets is equivalent to the theory of the Euclidean ones. However,
treating the world-sheets as Euclidean provides by itself a consistent theory of interacting
strings. The Euclidean version can be then used to learn as much as possible
about its Lorentzian counterpart.
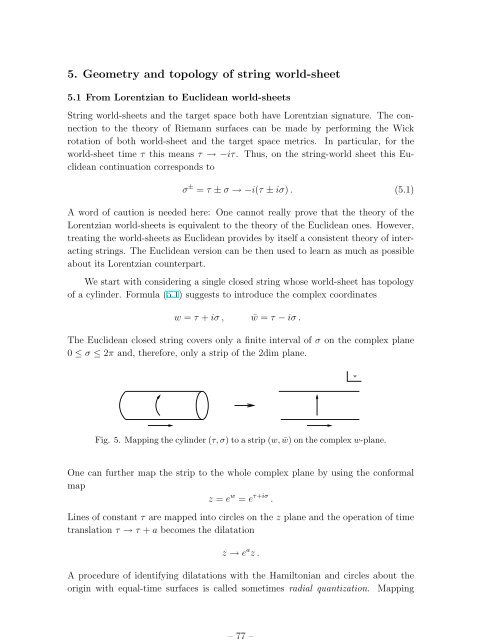
We start with considering a single closed string whose world-sheet has topology
of a cylinder. Formula (5.1) suggests to introduce the complex coordinates
w = τ + iσ , ¯w = τ − iσ .
The Euclidean closed string covers only a finite interval of σ on the complex plane
0 ≤ σ ≤ 2π and, therefore, only a strip of the 2dim plane.
w
Fig. 5. Mapping the cylinder (τ, σ) to a strip (w, ¯w) on the complex w-plane.
One can further map the strip to the whole complex plane by using the conformal
map
z = e w = e τ+iσ .
Lines of constant τ are mapped into circles on the z plane and the operation of time
translation τ → τ + a becomes the dilatation
z → e a z .
A procedure of identifying dilatations with the Hamiltonian and circles about the
origin with equal-time surfaces is called sometimes radial quantization. Mapping