- Page 2:
Astroparticle Physics
- Page 6:
Prof. Dr. Claus GrupenUniversity of
- Page 12:
VIPrefaceand M.Sc. Mehmet T. Kurt (
- Page 16:
VIIIPrefaceOn top of that, the basi
- Page 20:
XTable of Contents5 Acceleration Me
- Page 24:
XIITable of Contents11 The Cosmic M
- Page 30:
11 Historical Introduction“Look i
- Page 34:
1.1 Discoveries in the 20th Century
- Page 38:
1.1 Discoveries in the 20th Century
- Page 42:
1.1 Discoveries in the 20th Century
- Page 46:
1.2 Discoveries of New Elementary P
- Page 50:
1.3 Start of the Satellite Era 11ex
- Page 54:
1.3 Start of the Satellite Era 13CO
- Page 58:
1.3 Start of the Satellite Era 1519
- Page 62:
1.4 Open Questions 171989) and at e
- Page 66:
1.5 Problems 191.5 Problems1. Work
- Page 72:
22 2 The Standard Model of Elementa
- Page 84:
}28 2 The Standard Model of Element
- Page 88:
30 2 The Standard Model of Elementa
- Page 92:
32 2 The Standard Model of Elementa
- Page 98:
353 Kinematics and Cross Sections
- Page 102:
3.1 Threshold Energies 373.1 Thresh
- Page 106:
3.2 Four-Vectors 39Example 3: Consi
- Page 110:
3.2 Four-Vectors 41Example 6: Muon
- Page 114:
3.2 Four-Vectors 43Because of E γ2
- Page 118:
3.3 Lorentz Transformation 45energi
- Page 122:
3.5 Problems 47If j is the particle
- Page 126:
494 Physics of Particleand Radiatio
- Page 130:
4.2 Interaction Processes Used for
- Page 134:
4.2 Interaction Processes Used for
- Page 138:
4.3 Principles of the Atmospheric A
- Page 142:
4.4 Special Aspects of Photon Detec
- Page 146:
4.6 Propagation and Interactions of
- Page 150:
4.8 Problems 61board of a satellite
- Page 154:
635 Acceleration Mechanisms“Physi
- Page 158:
5.2 Acceleration by Sunspot Pairs 6
- Page 162:
5.3 Shock Acceleration 67The ejecte
- Page 166:
5.5 Pulsars 69Since this accelerati
- Page 170:
5.6 Binaries 71one obtains, using E
- Page 174:
5.7 Energy Spectra of Primary Parti
- Page 178:
5.7 Energy Spectra of Primary Parti
- Page 182:
776 Primary Cosmic Rays“It will b
- Page 186:
6.1 Charged Component of Primary Co
- Page 190:
6.1 Charged Component of Primary Co
- Page 194:
6.1 Charged Component of Primary Co
- Page 198:
6.1 Charged Component of Primary Co
- Page 202:
6.2 Neutrino Astronomy 87is only co
- Page 206:
6.2 Neutrino Astronomy 89ν e + e
- Page 210:
6.2 Neutrino Astronomy 91For an ass
- Page 214:
6.2 Neutrino Astronomy 93upward-goi
- Page 218:
6.2 Neutrino Astronomy 957 Be + p
- Page 222:
6.2 Neutrino Astronomy 97A reconstr
- Page 226:
6.2 Neutrino Astronomy 99nos (or in
- Page 230:
6.2 Neutrino Astronomy 101In the ho
- Page 234:
6.2 Neutrino Astronomy 103The exper
- Page 238:
6.2 Neutrino Astronomy 105muon spec
- Page 242:
6.2 Neutrino Astronomy 107The setup
- Page 246:
6.3 Gamma Astronomy 109All parts of
- Page 250:
6.3 Gamma Astronomy 111The energy s
- Page 254:
6.3 Gamma Astronomy 1136.3.3 Measur
- Page 258:
6.3 Gamma Astronomy 115v = c n = 29
- Page 262:
6.3 Gamma Astronomy 117The relation
- Page 266:
6.3 Gamma Astronomy 119Future space
- Page 270:
6.3 Gamma Astronomy 121γ -ray burs
- Page 274:
6.4 X-Ray Astronomy 123bursters all
- Page 278:
6.4 X-Ray Astronomy 125synchrotron
- Page 282:
6.4 X-Ray Astronomy 127In 1952 Wolt
- Page 286:
6.4 X-Ray Astronomy 129In 1959 the
- Page 290:
6.4 X-Ray Astronomy 131Measurements
- Page 294:
6.5 Gravitational-Wave Astronomy 13
- Page 298:
6.5 Gravitational-Wave Astronomy 13
- Page 302:
6.6 Problems 1373. Radiation exposu
- Page 306:
6.6 Problems 139the star and its te
- Page 310:
1417 Secondary Cosmic Rays“There
- Page 314:
7.1 Propagation in the Atmosphere 1
- Page 318:
7.1 Propagation in the Atmosphere 1
- Page 322:
7.2 Cosmic Rays at Sea Level 1477.2
- Page 326:
7.2 Cosmic Rays at Sea Level 149muo
- Page 330:
7.3 Cosmic Rays Underground 151N(ν
- Page 334:
7.3 Cosmic Rays Underground 153[ a
- Page 338:
7.3 Cosmic Rays Underground 155Fig.
- Page 342:
7.4 Extensive Air Showers 157hadron
- Page 346:
7.4 Extensive Air Showers 159precis
- Page 350:
7.4 Extensive Air Showers 161primar
- Page 354:
7.5 Nature and Origin of the Highes
- Page 358:
7.5 Nature and Origin of the Highes
- Page 362:
7.5 Nature and Origin of the Highes
- Page 366:
7.6 Problems 169‘Milky Way’ of
- Page 370:
1718 Cosmology“As far as the laws
- Page 374:
8.1 The Hubble Expansion 173isotrop
- Page 378:
8.2 The Isotropic and Homogeneous U
- Page 382:
8.3 The Friedmann Equation from New
- Page 386:
8.4 The Friedmann Equation from Gen
- Page 390:
8.4 The Friedmann Equation from Gen
- Page 394:
8.7 Nature of Solutions to the Frie
- Page 398:
8.7 Nature of Solutions to the Frie
- Page 402:
8.8 Experimental Evidence for the V
- Page 406:
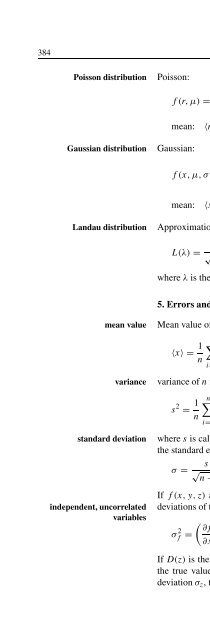
8.9 Problems 189where E max is the
- Page 410:
1919 The Early Universe“Who cares
- Page 414:
9.2 Thermodynamics of the Early Uni
- Page 418:
9.2 Thermodynamics of the Early Uni
- Page 422:
9.2 Thermodynamics of the Early Uni
- Page 426:
9.3 Solving the Friedmann Equation
- Page 430:
9.3 Solving the Friedmann Equation
- Page 434:
9.4 Thermal History of the First Te
- Page 438:
9.5 The Baryon Asymmetry of the Uni
- Page 442:
9.5 The Baryon Asymmetry of the Uni
- Page 446:
9.5 The Baryon Asymmetry of the Uni
- Page 450:
9.6 Problems 211investigation of CP
- Page 454:
21310 Big Bang Nucleosynthesis“In
- Page 458:
10.2 Start of the BBN Era 215these
- Page 462:
10.3 The Neutron-to-Proton Ratio 21
- Page 466:
10.4 Neutrino Decoupling, Positron
- Page 470:
10.5 Synthesis of Light Nuclei 221i
- Page 474:
10.6 Detailed BBN 223Fig. 10.4Evolu
- Page 478:
10.6 Detailed BBN 225prediction for
- Page 482:
10.7 Constraints on the Number of N
- Page 486:
22911 The Cosmic MicrowaveBackgroun
- Page 490:
11.2 Discovery and Basic Properties
- Page 494:
11.3 Formation of the CMB 23311.3 F
- Page 498:
11.4 CMB Anisotropies 235Once the p
- Page 502:
11.5 The Monopole and Dipole Terms
- Page 506:
11.6 Determination of Cosmological
- Page 510:
11.6 Determination of Cosmological
- Page 514:
11.6 Determination of Cosmological
- Page 518:
24512 Inflation“Inflation hasn’
- Page 522:
12.2 The Flatness Problem 247Althou
- Page 526:
12.3 The Monopole Problem 249place
- Page 530:
12.4 How Inflation Works 251the pre
- Page 534:
12.5 Mechanisms for Inflation 253On
- Page 538:
12.5 Mechanisms for Inflation 255th
- Page 542:
12.6 Solution to the Flatness Probl
- Page 546:
12.8 Solution to the Monopole Probl
- Page 550:
12.9 Inflation and the Growth of St
- Page 554:
12.10 Outlook on Inflation 263years
- Page 558:
26513 Dark Matter“There is a theo
- Page 562:
13.2 Motivation for Dark Matter 267
- Page 566:
13.2 Motivation for Dark Matter 269
- Page 570:
13.2 Motivation for Dark Matter 271
- Page 574:
13.2 Motivation for Dark Matter 273
- Page 578:
13.2 Motivation for Dark Matter 275
- Page 582:
13.2 Motivation for Dark Matter 277
- Page 586:
13.2 Motivation for Dark Matter 279
- Page 590:
13.2 Motivation for Dark Matter 281
- Page 594:
13.2 Motivation for Dark Matter 283
- Page 598:
13.3 Problems 285map of about 1 ◦
- Page 602:
28714 Astrobiology“In the beginni
- Page 606:
14 Astrobiology 289on the chances t
- Page 610:
14.1 Problems 2913. What determines
- Page 616:
294 15 OutlookTheory of Everythingo
- Page 620:
296 15 Outlookmatter dominanceprimo
- Page 626:
29916 Glossary“A good notation ha
- Page 630:
16 Glossary 301The antiparticle of
- Page 634:
16 Glossary 303The Big Rip is a cos
- Page 638:
16 Glossary 305Stars which have rap
- Page 642:
16 Glossary 307A quantity is conser
- Page 646:
16 Glossary 309X-ray binary consist
- Page 650:
16 Glossary 311The electron and its
- Page 654:
16 Glossary 313Particle detector fo
- Page 658:
16 Glossary 315The interaction of p
- Page 662:
16 Glossary 317High Resolution Imag
- Page 666:
16 Glossary 319Short for Large Elec
- Page 670:
16 Glossary 321Distant galaxies wit
- Page 674:
16 Glossary 323Transmutation of a n
- Page 678:
16 Glossary 325See fermion.The poin
- Page 682:
16 Glossary 327Quantum anomalies ca
- Page 686:
16 Glossary 329The metric describin
- Page 690:
16 Glossary 331This theory refers t
- Page 694:
16 Glossary 333Particles whose spin
- Page 698:
16 Glossary 335(c) The value of the
- Page 702:
33717 Solutions“The precise state
- Page 706:
17.1 Chapter 1 3394. By definition
- Page 710:
17.2 Chapter 2 3412. For any unstab
- Page 714:
17.4 Chapter 4 3434.p b❅■ ✒
- Page 718:
17.4 Chapter 4 3455. From the expre
- Page 722:
17.5 Chapter 5 347Consequences:The
- Page 726:
17.6 Chapter 6 3493. a) The average
- Page 730:
17.6 Chapter 6 351b) On both sides
- Page 734:
17.6 Chapter 6 353This gives the sc
- Page 738:
17.6 Chapter 6 3555. Measured flux
- Page 742:
17.7 Chapter 7 3574. N(> E,R) = A(a
- Page 746: 17.8 Chapter 8 359Since E = hν = h
- Page 750: 17.8 Chapter 8 3615. Photon mass m
- Page 754: 17.9 Chapter 9 3639. Schwarzschild
- Page 758: 17.9 Chapter 9 365which is the Schw
- Page 762: 17.10 Chapter 10 367whereσ√hadr
- Page 766: 17.11 Chapter 11 3694. Planck distr
- Page 770: 17.12 Chapter 12 371The related mas
- Page 774: 17.13 Chapter 13 373(p ′ W −m W
- Page 778: 17.14 Chapter 14 375The mass of a t
- Page 782: 17.14 Chapter 14 3770 = ddT[ 1 (T 3
- Page 786: 17.15 Chapter 15 3790 =−F eff (r)
- Page 792: 382 A Mathematical Appendixexamples
- Page 798: A.2 Mathematics for Angular Variati
- Page 802: A.2 Mathematics for Angular Variati
- Page 806: 389B Results from Statistical Physi
- Page 810: B.1 Statistical Mechanics Review 39
- Page 814: B.1 Statistical Mechanics Review 39
- Page 818: B.1 Statistical Mechanics Review 39
- Page 822: B.2 Number and Energy Densities 397
- Page 826: B.3 Equations of State 399which rel
- Page 830: 401C Definition of Equatorialand Ga
- Page 834: 403D Important Constants for Astrop
- Page 838: 405References“References are like
- Page 842: 407Further Reading“Education is t
- Page 846:
Further Reading 409Ulf Borgeest, Ka
- Page 850:
Further Reading 411M. A. Bucher, D.
- Page 856:
414 Photo Credits{20} ESA/XMM-Newto
- Page 860:
416 Indexanisotropy, 85, 86- cosmic
- Page 864:
418 Index- of hydrogen, 94, 222, 22
- Page 868:
420 Index- parameters, 243- - deter
- Page 872:
422 Indexeffective number of degree
- Page 876:
424 IndexFeynman diagram, 26, 98, 2
- Page 880:
426 IndexHarrison-Zel’dovich spec
- Page 884:
428 IndexKoshiba, M., 13kpc, 318, s
- Page 888:
430 Index- standard, see StandardMo
- Page 892:
432 Index- primary, energy spectrum
- Page 896:
434 Indexplanet, extrasolar, 18plan
- Page 900:
436 Indexrange- relation, energy-,
- Page 904:
438 Index- dark, 269- double, see b
- Page 908:
440 Indexuncertainty- principle, se


![arXiv:1001.0993v1 [hep-ph] 6 Jan 2010](https://img.yumpu.com/51282177/1/190x245/arxiv10010993v1-hep-ph-6-jan-2010.jpg?quality=85)

![arXiv:1008.3907v2 [astro-ph.CO] 1 Nov 2011](https://img.yumpu.com/48909562/1/190x245/arxiv10083907v2-astro-phco-1-nov-2011.jpg?quality=85)








![arXiv:1002.4928v1 [gr-qc] 26 Feb 2010](https://img.yumpu.com/41209516/1/190x245/arxiv10024928v1-gr-qc-26-feb-2010.jpg?quality=85)
![arXiv:1206.2653v1 [astro-ph.CO] 12 Jun 2012](https://img.yumpu.com/39510078/1/190x245/arxiv12062653v1-astro-phco-12-jun-2012.jpg?quality=85)
