principles and applications of microearthquake networks

124 5. Inversion and Optimization
information about derivatives. In general, the optimization problem can
be solved more effectively if more information about derivatives is provided.
However, this must be traded off against the extra computing
needed to compute the derivatives. Search methods usually are not effective
when the function to be optimized has more than one independent
variable. Methods in the third class are modifications of the classical
Newton-Raphson (or Newton) method. Methods in the second and third
classes may be referred to as derivative methods, and are discussed next.
5.4.2. Derivative Methods
These methods for optimization are based on the Taylor expansion of
the objective function. For a function of one variable, f(x>, which is differentiable
at least n times in an interval containing points (x) and (x + ax),
we may expand f (x + ax) in terms of f(x) as given by Courant and John
( 1965, p. 446) as
(5.67) f(x + ax) = f(x) + dm
dX
6s + . . . +-----
1 d"f(x)
n ! dx'l
For a function of n variables, F(x), where x is given by Eq. (5.65), we may
generalize this procedure (Courant and John, 1974, pp. 68-70) and write
(5.68) F(x + 6x) = F(x) + g T 6x + @XTH 6x + . . .
where gT is the transpose of the gradient vector g and is given by
(5.69) gT = VF(x) = (dF/ds,, dF/dx2,
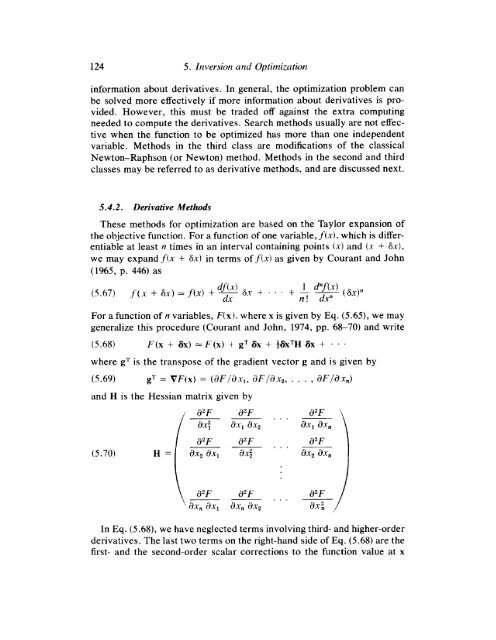
and H is the Hessian matrix given by
i
a2F d2F
ax; ax, ax2
d2F a'F
(5.70) H = ax2 as, ax;
...
..
\
I
ax, ax,
d2F
ax, ax,
a'F i)*F
\ ax, ax, ax, ax, .
In Eq. (5.68), we have neglected terms involving third- and higher-order
derivatives. The last two terms on the right-hand side of Eq. (5.68) are the
first- and the second-order scalar corrections to the function value at x