Sullivan Microsite DigiSample

Sullivan AP˙Sullivan˙Chapter01 October 8, 2016 17:4
Section 1.1 • Limits of Functions Using Section Numerical 1.1 • Assess and Graphical Your Understanding Techniques 89 83
(b) Investigate lim cos π by using a table and Solution evaluating Thethe
function f is a(c) piecewise-defined Graph the function function. C. Its graph is shown in Figure 12.
x→0 x2 y
function f (x) = cos π Observe that as x approaches
x 2 at
(d)
2
Use
from
the graph
the left,
to investigate
the value
lim
of f
C(w)
is close
and
to
lim
7,
C(w).
and as
Do
x
w→1− w→1 +
10
approaches 2 from the right, the value of f is close to 7. In fact, we can make the value
(2, 10)
x =− 2 3 , − 2 5 , − 2 7 , − 2 9 ,..., 2 9 , 2 7 , 2 5 , 2 of f as close as we please to 7 these by choosing suggest that x sufficiently lim C(w) exists?
3 .
w→1
close to 2 but not equal to 2.
This suggests lim f (x) = 7. ■
(e) Use the graph to investigate lim C(w) and lim x→2
w→12− w→12
(c) Compare the results from (a) and (b). What do you conclude
7
If we use a table to investigate lim f (x), the result is the same. See Table 3.
Do these
about the limit? Why do you think this happens? What is your
x→2 suggest that lim C(w) exists?
w→12
view about using a table to draw a conclusion about limits? (f) Use the graph to investigate lim
TABLE 3
C(w).
w→0 +
(d) Use technology to graph f . Begin with the x-window
(g) Use the graph to investigate lim w→13
[−2π, 2π] and the y-window [−1, 1]. If you were x approaches finding 2 from the left
x approaches − 2 from the right
−−−−−−−−−−−−−−−−−−−−−→
←−−−−−−−−−−−−−−−−−−−−−−
lim f (x) using a graph, what would you conclude? Zoom in 61. Correlating Student Success to Study Time Professor Smith
x→0 x 1.99 1.999 1.9999 1.99999 → 2 ← 2.00001 2.0001 2.001 2.01
on the graph. Describe what you see. (Hint: Be sure your
claims that a student’s final exam score is a function of the time t
f (x) 6.97 6.997 6.9997 6.99997
calculator is set to the radian mode.)
(in hours) that f (x) the approaches student studies. 7 7.00003 He claims 7.0003 that the7.003 closer 7.03 to
PAGE
x − 8
seven hours one studies, the closer to 100% the student scores
85 57. (a) Use a table 1 to investigate 2 3 limx
.
x→2 2
on the final. He claims that studying significantly less than seven
(b) How close must { x be to 2, so that f (x) is within
Figure
0.1 of
12
the
shows that f hours (2) = may 10cause but that one to this bevalue underprepared has no impact for the test, on the while limit as
3x + 1 if x = 2
Figure 12 limit? f (x) =
x approaches 2. In fact, f (2) studying can equal significantly any number, more than and seven it would hours have mayno cause effect on the
10 if x = 2
(c) How close must x be to 2, so that f (x) islimit within as0.01 x approaches of the 2. “burnout.”
limit?
58. (a) Use a table to investigate lim(5 − 2x).
x→2
We make the following(a) observations: Write Professor Smith’s claim symbolically as a limit.
• The limit L of a function (b) y Write = f (x) Professor as x approaches Smith’s claima number using thec ε-δ does definition not depend
(b) How close must x be to 2, so that f (x) is within on the 0.1 of value the of f at c. of limit.
limit?
• The limit L of a function Source: y = Submitted f (x) as xbyapproaches the students aofnumber Millikin University. c is unique; that is,
(c) How close must x be to 2, so that f (x) is within a function 0.01 of the cannot have more than one limit. (A proof of this property is given in
62. The definition of the slope of the tangent line to the graph of
limit?
Appendix B.)
f (x) − f (c)
59. First-Class Mail As of April
• If there is no single number y = f that (x) at the the value point of (c, f f approaches (c)) is m tan = aslim
.
x→c
x gets
2016, the U.S. Postal Service
xclose − c to c, we
say that f has no limit as x approaches c, or more simply, that the limit of f does
charged $0.47 postage for
Another way to express this slope is to define a new variable
not exist at c.
first-class letters weighing up to
h = x − c. Rewrite the slope of the tangent line m tan using h and c.
and including 1 ounce, plus a flat
63. If f (2) = 6, can you conclude anything about lim f (x)? Explain
fee of $0.21 for each additional
x→2
your reasoning. NOW WORK AP® Practice Problem 4.
or partial ounce up to and
64. If lim f (x) = 6, can you conclude anything about f (2)? Explain
including 3.5 ounces. First-class
x→2
letter rates do not apply to letters Examples 6 and 7 that follow your illustrate reasoning. situations in which a limit does not exist.
weighing more than 3.5 ounces.
65. The graph of f (x) = x − 3
CALC
is a straight line with a point punched
Source: U.S. Postal Service Notice 123 EXAMPLE 6 Investigating 3 − x
out. a Limit Using a Graph
CLIP
{
(a) Find a function C that models the first-class postage charged, (a) What straight line and x
what if
point? x < 0
Use a graph to investigate lim f (x) if f (x) =
in dollars, for a letter weighing w ounces. Assume w>0.
x→0 1 if x > 0 .
(b) Use the graph of f to investigate the one-sided limits of f as
(b) What is the y domain of C?
Solution Figure 13 shows the graph x approaches of f . We 3. first investigate the one-sided limits. The
(c) Graph the2
function C.
graph suggests that, as x approaches (c) Does the 0 from graphthe suggest left, that lim f (x) exists? If so, what is it?
(d) Use the graph to investigate lim C(w) and lim C(w). Do
x→3
1
w→2− w→2 + lim
66. (a) Use a table to investigate x→0 lim(1 + x)
these suggest that lim C(w) exists?
.
x→0
w→2
(e) Use the graph to investigate lim and as x approaches 0 from(b) theUse right, graphing technology to graph g(x) = (1 + x) 1/x .
2 1 1 2 3 x
w→0 + (c) What lim do (a) and (b) suggest about lim(1 + x) 1/x ?
1
(f) Use the graph to investigate lim x→0 + x→0
w→3.5 − CAS (d) Find lim(1 + x) 1/x .
2
Since there is no single number that x→0
the values of f approach when x is close to 0, we
60. First-Class Mail As of April 2016, the U.S. conclude Postal Service that lim f (x) does not exist. ■
charged $0.94 postage { for first-class large envelope weighing x→0
x if x < 0
up to
Figure and13 including f (x) = 1 ounce, plus a flat fee of $0.21 for each Tableadditional
4 uses a numerical approach to support the conclusion that lim f (x) does not
1 if x > 0
or partial ounce up to and including 13 ounces. First-class rates do Challenge Problems
x→0
exist.
not apply to large envelopes weighing more than 13 ounces. For Problems 67–70, investigate each of the following limits.
Source: U.S. Postal Service Notice 123
{
TABLE 4
1 if x is an integer
f (x) =
(a) Find a function C that models the first-class postage x approaches charged, 0 from the left
0 if xis approaches not an integer 0 from the right
in dollars, for a large envelope weighing w ounces. −−−−−−−−−−−−−−−−−−−−→
Assume
←−−−−−−−−−−−−−−−−−−−−−
w>0.
x −0.01 −0.00167. −0.0001 lim f (x) x→2
→68. 0 lim f ←(x) x→1/2
69. 0.0001 lim f (x) 0.001 70.
x→3
lim 0.01
(b) What is the domain of C?
f (x) −0.01 −0.001 −0.0001 no single number 1 1 1
Teaching Tip
In Section 1.3, students will learn the formal
definition of continuity. Problems about continuity
on the exams often involve piecewise functions.
When investigating the limits of piecewise functions
graphically, consider checking the limits using
direct substitution into the appropriate portions
of the piecewise function. Doing so will lay the
groundwork for Section 1.3. Here you want to focus
heavily on getting students to write the one-sided
limits correctly. A graphic organizer can be useful
here, and a teacher modeling this correctly when
students first see it will save many mistakes later.
This is the template.
lim ( what function ?) = ( what yvalue ?)
x → 1
+
( x approaches what value ?)
The student can then set up the organizer with
blanks for the parenthetical expressions.
Kathryn Sidenstricker /Dreamstime.com
Examples 5 and 6 lead to the following result.
AP® CaLC skill builder
for example 6
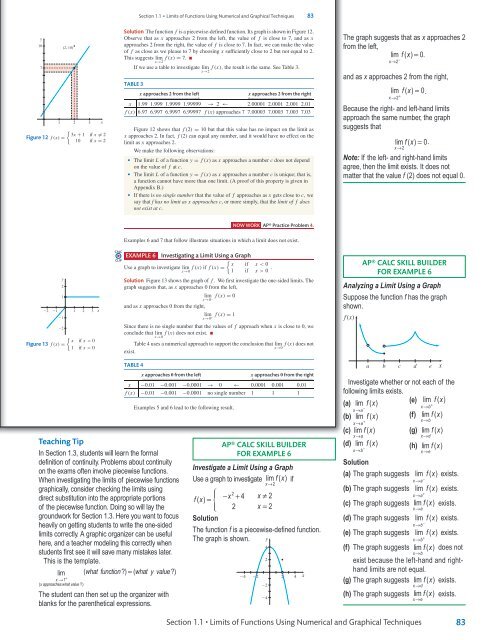
Investigate a Limit Using a Graph
Use a graph to investigate lim fx ( ) if
x→2
⎧⎪
2
fx ( ) =
− x + 4 x ≠ 2
⎨
⎩⎪ 2 x = 2
Solution
The function f is a piecewise-defined function.
The graph is shown. y
24
22
4
2
22
24
2
4
x
The graph suggests that as x approaches 2
from the left,
lim fx ( ) = 0.
x→2
−
and as x approaches 2 from the right,
lim fx ( ) = 0.
x→ 2
+
Because the right- and left-hand limits
approach the same number, the graph
suggests that
lim fx ( ) = 0.
x→2
Note: If the left- and right-hand limits
agree, then the limit exists. It does not
matter that the value f (2) does not equal 0.
AP® CaLC skill builder
for example 6
Analyzing a Limit Using a Graph
Suppose the function f has the graph
shown.
f (x)
a b c d e
Investigate whether or not each of the
following limits exists.
(a) lim fx ( )
(e) lim fx ( )
x→a
− x→ b
+
(b) lim fx ( ) (f) lim fx ( )
x→ a
+ x→b
(c) lim fx ( ) (g) lim fx ( )
x→a
x→d
(d) lim fx ( ) (h) lim fx ( )
x→b
− x→e
Solution
(a) The graph suggests lim fx ( ) exists.
x→a
−
(b) The graph suggests lim fx ( ) exists.
x→ a
+
(c) The graph suggests lim fx ( ) exists.
x→a
(d) The graph suggests lim fx ( ) exists.
x→b
−
(e) The graph suggests lim fx ( ) exists.
x→ b
+
(f) The graph suggests lim fx ( ) does not
x→b
exist because the left-hand and righthand
limits are not equal.
(g) The graph suggests lim fx ( ) exists.
(h) The graph suggests
x→d
x→e
x
lim fx ( ) exists.
x→0 f (x) 83
Section 1.1 • Limits of Functions Using Numerical and Graphical Techniques
TE_Sullivan_Chapter01_PART 0.indd 12
11/01/17 9:52 am