Sullivan Microsite DigiSample

Sullivan AP˙Sullivan˙Chapter01 October 8, 2016 17:4
Sullivan
108 Chapter 1 • Limits and Continuity
Teaching Tip
Consider teaching the overall principles
without relying on rote learning of
the theorems presented, such as the
continuity of a sum, difference, product,
and quotient presented on this page.
Students may balk at the number of
theorems presented, when in reality, they
only have to understand the overarching
principle of how to determine if a function
is continuous at a point and how to identify
points of discontinuity algebraically.
Proof If P is a polynomial function, its domain is the set of real numbers. For a
polynomial function,
lim P(x) = P(c)
x→c
for any number c. That is, a polynomial function is continuous at every real number.
If R(x) = p(x) is a rational function, then p(x) and q(x) are polynomials and the
q(x)
domain of R is {x|q(x) = 0}. The Limit of a Rational Function (p. 96) states that for
all c in the domain of a rational function,
lim R(x) = R(c)
x→c
So a rational function is continuous at every number in its domain. ■
To summarize:
• If a function is continuous on an interval, its graph has no holes or gaps on that
interval.
• If a function is continuous on its domain, it will be continuous at every number
in its domain; its graph may have holes or gaps at numbers that are not in the
domain.
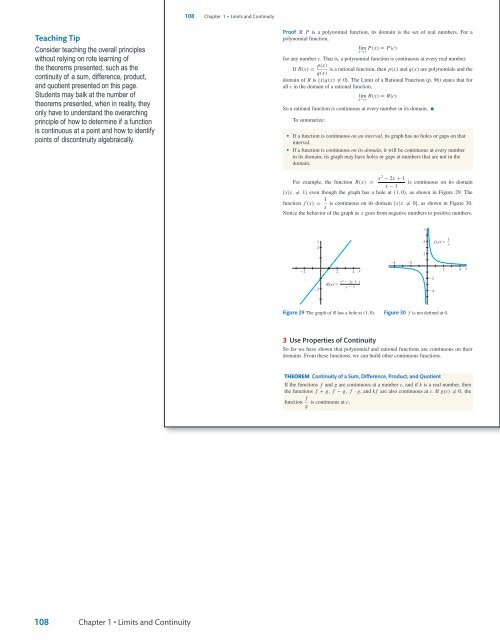
For example, the function R(x) = x 2 − 2x + 1
is continuous on its domain
x − 1
{x|x = 1} even though the graph has a hole at (1, 0), as shown in Figure 29. The
function f (x) = 1 is continuous on its domain {x|x = 0}, as shown in Figure 30.
x
Notice the behavior of the graph as x goes from negative numbers to positive numbers.
y
y
2
4
2
f (x) 1 x
2
2
4
x
4
2
2
4
x
2
R(x) x2 2x 1
x 1
2
4
Figure 29 The graph of R has a hole at (1, 0).
Figure 30 f is not defined at 0.
3 Use Properties of Continuity
So far we have shown that polynomial and rational functions are continuous on their
domains. From these functions, we can build other continuous functions.
THEOREM Continuity of a Sum, Difference, Product, and Quotient
If the functions f and g are continuous at a number c, and if k is a real number, then
the functions f + g, f − g, f · g, and kf are also continuous at c. If g(c) = 0, the
function f is continuous at c.
g
108
Chapter 1 • Limits and Continuity
TE_Sullivan_Chapter01_PART I.indd 5
11/01/17 9:58 am