Sullivan Microsite DigiSample

Sullivan AP˙Sullivan˙Chapter01 October 8, 2016 17:4
Sullivan
112 Chapter 1 • Limits and Continuity
Must-Do Problems for
Exam Readiness
AB: 13–18, 19–35 odd, 59–63 odd, AP ®
Practice Problems
BC: 13–18, 24, 25, 29, 51–56, 79, and all
AP ® Practice Problems
TRM Full Solutions to Section
1.3 Problems and AP® Practice
Problems
Answers to Section 1.3
Problems
1. True.
2. False.
3. fc () is defined, lim fx ( ) exists,
x→c
lim fx ( ) = fc ()
x→c
4. True.
5. False.
6. False.
7. False.
8. True.
9. Discontinuous.
10. Continuous.
11. True.
12. False.
13. (a) Discontinuous at c =− 3.
(b) lim ( ) ≠ f ( −3)
fx
x→−3
(c) Removable.
(d) f ( − 3) =−2
14. (a) Continuous at c = 0.
15. (a) Discontinuous at c = 2.
(b) lim fx ( ) does not exist.
x→2
(c) Not removable.
16. (a) Discontinuous at c = 3.
(b) lim fx ( ) does not exist.
x→3
(c) Not removable.
17. (a) Continuous at c = 4.
18. (a) Discontinuous at c = 5.
(b) f not defined at c = 5, and
lim fx ( ) ≠ lim fx ( ) so lim fx ( ) does
− +
x→5 x→5
x→5
not exist.
(c) Not removable.
19. Continuous at c =− 1.
20. Continuous at c = 5.
21. Continuous at c =− 2.
22. Discontinuous at c = 2.
23. Continuous at c = 2.
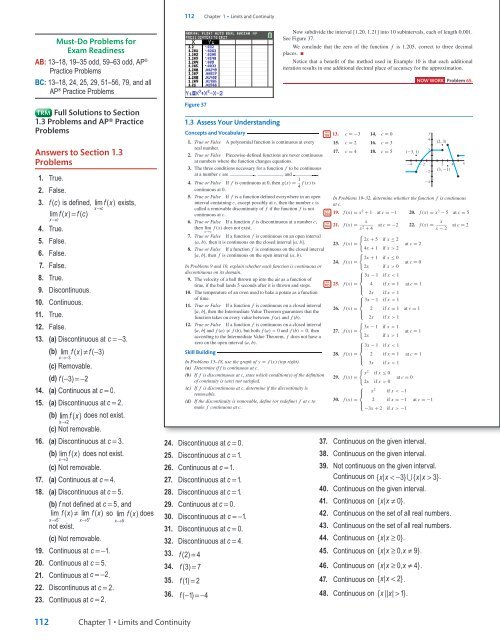
Figure 37
1.3 Assess Your Understanding
Now subdivide the interval [1.20, 1.21] into 10 subintervals, each of length 0.001.
See Figure 37.
We conclude that the zero of the function f is 1.205, correct to three decimal
places. ■
Notice that a benefit of the method used in Example 10 is that each additional
iteration results in one additional decimal place of accuracy for the approximation.
Concepts and Vocabulary
PAGE
105 13. c =−3 14. c = 0
1. True or False A polynomial function is continuous at every 15. c = 2 16. c = 3
real number.
2. True or False Piecewise-defined functions are never continuous
at numbers where the function changes equations.
3. The three conditions necessary for a function f to be continuous
17. c = 4 18. c = 5
at a number c are , , and .
4. True or False If f is continuous at 0, then g(x) = 1 f (x) is
4
continuous at 0.
5. True or False If f is a function defined everywhere in an open
interval containing c, except possibly at c, then the number c is
called a removable discontinuity of f if the function f is not
continuous at c.
6. True or False If a function f is discontinuous at a number c,
then lim f (x) does not exist.
x→c
7. True or False If a function f is continuous on an open interval
(a, b), then it is continuous on the closed interval [a, b].
8. True or False If a function f is continuous on the closed interval
[a, b], then f is continuous on the open interval (a, b).
In Problems 9 and 10, explain whether each function is continuous or
discontinuous on its domain.
9. The velocity of a ball thrown up into the air as a function of
time, if the ball lands 5 seconds after it is thrown and stops.
10. The temperature of an oven used to bake a potato as a function
of time.
11. True or False If a function f is continuous on a closed interval
[a, b], then the Intermediate Value Theorem guarantees that the
function takes on every value between f (a) and f (b).
12. True or False If a function f is continuous on a closed interval
[a, b] and f (a) = f (b), but both f (a) >0 and f (b) >0, then
according to the Intermediate Value Theorem, f does not have a
zero on the open interval (a, b).
Skill Building
In Problems 13–18, use the graph of y = f (x) (top right).
(a) Determine if f is continuous at c.
(b) If f is discontinuous at c, state which condition(s) of the definition
of continuity is (are) not satisfied.
(c) If f is discontinuous at c, determine if the discontinuity is
removable.
(d) If the discontinuity is removable, define (or redefine) f at c to
make f continuous at c.
24. Discontinuous at c = 0.
25. Discontinuous at c = 1.
26. Continuous at c = 1.
27. Discontinuous at c = 1.
28. Discontinuous at c = 1.
29. Continuous at c = 0.
30. Discontinuous at c =− 1.
31. Discontinuous at c = 0.
32. Discontinuous at c = 4.
33. f (2) = 4
34. f (3) = 7
35. f (1) = 2
36. f ( − 1) =−4
(3, 1)
NOW WORK Problem 65.
y
4
2
4 2 2 4
2 (3, 1)
4
(2, 3)
In Problems 19–32, determine whether the function f is continuous
at c.
PAGE
104 19. f (x) = x 2 + 1 at c =−1 20. f (x) = x 3 − 5 at c = 5
PAGE
104 21. f (x) = x
at c =−2 22. f (x) = x
x − 2
x 2 + 4
2x + 5 if x ≤ 2
23. f (x) =
at c = 2
4x + 1 if x > 2
2x + 1 if x ≤ 0
24. f (x) =
at c = 0
2x if x > 0
⎧
3x − 1 if x < 1
⎪⎨
PAGE
105 25. f (x) = 4 if x = 1 at c = 1
⎪⎩
2x if x > 1
⎧
3x − 1 if x < 1
⎪⎨
26. f (x) = 2 if x = 1 at c = 1
⎪⎩
2x if x > 1
3x − 1 if x < 1
27. f (x) =
at c = 1
2x if x > 1
⎧
3x − 1 ⎪⎨
if x < 1
28. f (x) = 2 if x = 1 at c = 1
⎪⎩
3x if x > 1
x 2 if x ≤ 0
29. f (x) =
at c = 0
2x if x > 0
⎧
⎪⎨
x 2 if x < −1
30. f (x) = 2 if x =−1
⎪⎩
−3x + 2 if x > −1
at c =−1
37. Continuous on the given interval.
38. Continuous on the given interval.
39. Not continuous on the given interval.
Continuous on { xx | 3}.
40. Continuous on the given interval.
41. Continuous on { xx | ≠ 0} .
42. Continuous on the set of all real numbers.
43. Continuous on the set of all real numbers.
44. Continuous on { xx | ≥ 0} .
45. Continuous on { xx | ≥0, x ≠ 9} .
46. Continuous on { xx | ≥0, x ≠ 4} .
47. Continuous on { xx | < 2} .
48. Continuous on { x|| x| > 1} .
x
at c = 2
112
Chapter 1 • Limits and Continuity
TE_Sullivan_Chapter01_PART I.indd 9
11/01/17 9:58 am