Sullivan Microsite DigiSample

More documents

Recommendations

Info

Sullivan AP˙Sullivan˙Chapter01 October 8, 2016 17:4 Sullivan 110 Chapter 1 • Limits and Continuity AP® Exam Tip y 4 y f 1 (x) y x The Intermediate Value Theorem sometimes appears on the multiplechoice portion of the exam. The Intermediate Value Theorem also appears sometimes as a portion of a free-response question. Even if it only appears sporadically on the exams, it is important that students learn it. For example, see the 2007 AP ® Calculus AB Exam, Question 3. 2 y f (x) 4 2 2 4 Figure 31 THEOREM Continuity of an Inverse Function If f is a one-to-one function that is continuous on its domain, then its inverse function f −1 is also continuous on its domain. 2 4 x AP® Exam Tip The three theorems that students must cite and use on the exam as a part of an answer or justification for an answer are the IVT (Intermediate Value Theorem), the EVT (Extreme Value Theorem, which will be learned in Section 4.2), and the MVT (Mean Value Theorem, which will be learned in Section 4.3). It is a good idea to have students practice them throughout the year. 5000 m 3765.6 m 2000 m 4 Use the Intermediate Value Theorem Functions that are continuous on a closed interval have many important properties. One of them is stated in the Intermediate Value Theorem. The proof of the Intermediate Value Theorem may be found in most books on advanced calculus. THEOREM The Intermediate Value Theorem Let f be a function that is continuous on a closed interval [a, b] and f (a) = f (b). If N is any number between f (a) and f (b), then there is at least one number c in the open interval (a, b) for which f (c) = N. To get a better idea of this result, suppose you climb a mountain, starting at an elevation of 2000 meters and ending at an elevation of 5000 meters. No matter how many ups and downs you take as you climb, at some time your altitude must be 3765.6 meters, or any other number between 2000 and 5000. In other words, a function f that is continuous on a closed interval [a, b] must take on all values between f (a) and f (b). Figure 32 illustrates this. Figure 33 shows why the continuity of the function is crucial. Notice in Figure 33 that there is a hole in the graph of f at the point (c, N). Because of the discontinuity at c, there is no number c in the open interval (a, b) for which f (c) = N. y y Teaching Tip f(b) f(b) A picture is worth 1,000 words! The Intermediate Value Theorem is very much a commonsense theorem that can easily be conveyed using Figures 32 and 33. N f(a) a f (c) N y f (x) c b x N f(a) a y f (x) c b x ∑ Mathematical Practices Tip MPAC 1: Reasoning with Definitions and Theorems Justifying answers will now include confirming that the hypotheses of theorems are met before stating the conclusions. The Web site AP ® Central, under the heading Teaching and Assessing AP ® Calculus, offers resources and short videos by AP ® Calculus teachers demonstrating how they prepare students. The module on continuity and differentiability is particularly good and can be accessed through a calculus teacher’s AP ® Audit account. Figure 32 f takes on every value between f (a) and f (b). Figure 33 A discontinuity at c results in no number c in (a, b) for which f (c) = N. The Intermediate Value Theorem is an existence theorem. It states that there is at least one number c for which f (c) = N, but it does not tell us how to find c. However, we can use the Intermediate Value Theorem to locate an open interval (a, b) that contains c. An immediate application of the Intermediate Value Theorem involves locating the zeros of a function. Suppose a function f is continuous on the closed interval [a, b] and f (a) and f (b) have opposite signs. Then by the Intermediate Value Theorem, there is TRM Section 1.3: Worksheet 3 This worksheet contains an explanation of the Intermediate Value Theorem and one problem based upon a portion of a former free-response exam question. 110 Chapter 1 • Limits and Continuity TE_Sullivan_Chapter01_PART I.indd 7 11/01/17 9:58 am
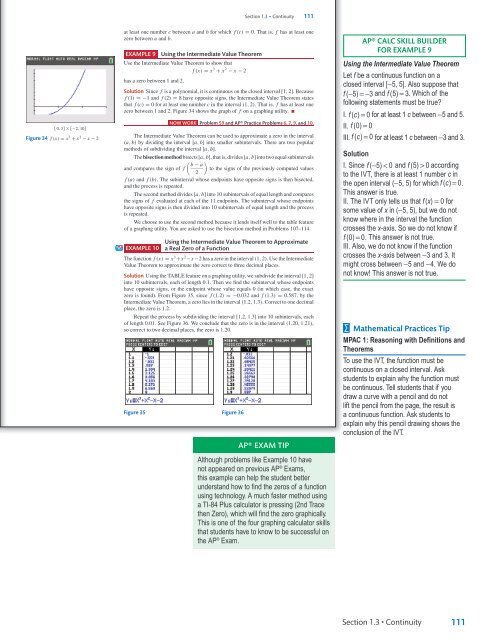
Sullivan AP˙Sullivan˙Chapter01 October 8, 2016 17:4 Section 1.3 • Continuity 111 f 0, 3g 3 f22, 10g Figure 34 f (x) = x 3 + x 2 − x − 2 at least one number c between a and b for which f (c) = 0. That is, f has at least one zero between a and b. EXAMPLE 9 Using the Intermediate Value Theorem Use the Intermediate Value Theorem to show that f (x) = x 3 + x 2 − x − 2 has a zero between 1 and 2. Solution Since f is a polynomial, it is continuous on the closed interval [1, 2]. Because f (1) =−1 and f (2) = 8 have opposite signs, the Intermediate Value Theorem states that f (c) = 0 for at least one number c in the interval (1, 2). That is, f has at least one zero between 1 and 2. Figure 34 shows the graph of f on a graphing utility. ■ NOW WORK Problem 59 and AP® Practice Problems 6, 7, 9, and 10. The Intermediate Value Theorem can be used to approximate a zero in the interval (a, b) by dividing the interval [a, b] into smaller subintervals. There are two popular methods of subdividing the interval [a, b]. The bisection method bisects [a, b], that is, divides [a, b] into two equal subintervals ( ) b − a and compares the sign of f to the signs of the previously computed values 2 f (a) and f (b). The subinterval whose endpoints have opposite signs is then bisected, and the process is repeated. The second method divides [a, b] into 10 subintervals of equal length and compares the signs of f evaluated at each of the 11 endpoints. The subinterval whose endpoints have opposite signs is then divided into 10 subintervals of equal length and the process is repeated. We choose to use the second method because it lends itself well to the table feature of a graphing utility. You are asked to use the bisection method in Problems 107–114. EXAMPLE 10 Using the Intermediate Value Theorem to Approximate a Real Zero of a Function The function f (x) = x 3 +x 2 −x −2 has a zero in the interval (1, 2). Use the Intermediate Value Theorem to approximate the zero correct to three decimal places. Solution Using the TABLE feature on a graphing utility, we subdivide the interval [1, 2] into 10 subintervals, each of length 0.1. Then we find the subinterval whose endpoints have opposite signs, or the endpoint whose value equals 0 (in which case, the exact zero is found). From Figure 35, since f (1.2) =−0.032 and f (1.3) = 0.587, by the Intermediate Value Theorem, a zero lies in the interval (1.2, 1.3). Correct to one decimal place, the zero is 1.2. Repeat the process by subdividing the interval [1.2, 1.3] into 10 subintervals, each of length 0.01. See Figure 36. We conclude that the zero is in the interval (1.20, 1.21), so correct to two decimal places, the zero is 1.20. Figure 35 Figure 36 AP® Exam Tip Although problems like Example 10 have not appeared on previous AP ® Exams, this example can help the student better understand how to find the zeros of a function using technology. A much faster method using a TI-84 Plus calculator is pressing (2nd Trace then Zero), which will find the zero graphically. This is one of the four graphing calculator skills that students have to know to be successful on the AP ® Exam. AP® Calc Skill Builder for Example 9 Using the Intermediate Value Theorem Let f be a continuous function on a closed interval [−5, 5]. Also suppose that f ( − 5) =− 3and f (5) = 3. Which of the following statements must be true? I. f() c= 0 for at least 1 c between −5 and 5. II. f (0) = 0 III. f() c= 0 for at least 1 c between −3 and 3. Solution I. Since f ( − 5) < 0 and f (5) > 0according to the IVT, there is at least 1 number c in the open interval (−5, 5) for which fc () = 0. This answer is true. II. The IVT only tells us that f (x) = 0 for some value of x in (−5, 5), but we do not know where in the interval the function crosses the x-axis. So we do not know if f (0) = 0. This answer is not true. III. Also, we do not know if the function crosses the x-axis between −3 and 3. It might cross between −5 and −4. We do not know! This answer is not true. ∑ Mathematical Practices Tip MPAC 1: Reasoning with Definitions and Theorems To use the IVT, the function must be continuous on a closed interval. Ask students to explain why the function must be continuous. Tell students that if you draw a curve with a pencil and do not lift the pencil from the page, the result is a continuous function. Ask students to explain why this pencil drawing shows the conclusion of the IVT. Section 1.3 • Continuity 111 TE_Sullivan_Chapter01_PART I.indd 8 11/01/17 9:58 am
Page 2 and 3: Chapter 1 Limits and Continuity Ove
Page 4 and 5: Chapter 1: Resources TRM Teacher’
Page 6 and 7: Sullivan AP˙Sullivan˙Chapter01 Oc
Page 52 and 53: Teaching Tip Alternate Example Find

Sullivan Microsite DigiSample

Create successful ePaper yourself

Delete template?

Save as template?