Sullivan Microsite DigiSample
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
<strong>Sullivan</strong> AP˙<strong>Sullivan</strong>˙Chapter01 October 8, 2016 17:4<br />
<strong>Sullivan</strong><br />
132 Chapter 1 • Limits and Continuity<br />
Teaching Tip<br />
When studying the limit of functions as x<br />
approaches ∞ or −∞, it helps students to<br />
keep the following generalizations in mind:<br />
Infinity in the denominator:<br />
2<br />
y<br />
4<br />
2<br />
4 2 4<br />
2<br />
x<br />
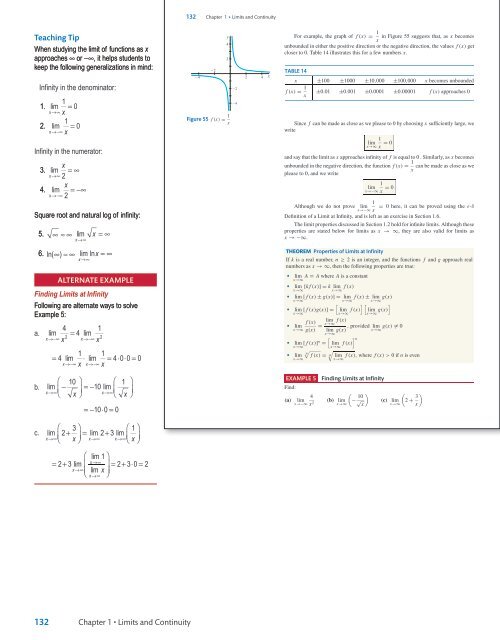
For example, the graph of f (x) = 1 in Figure 55 suggests that, as x becomes<br />
x<br />
unbounded in either the positive direction or the negative direction, the values f (x) get<br />
closer to 0. Table 14 illustrates this for a few numbers x.<br />
TABLE 14<br />
x ±100 ±1000 ±10,000 ±100,000 x becomes unbounded<br />
f (x) = 1 x<br />
±0.01 ±0.001 ±0.0001 ±0.00001 f (x) approaches 0<br />
1. lim 1 = 0<br />
x→∞ x<br />
4<br />
2. lim 1 = 0<br />
x→−∞ x<br />
Infinity in the numerator:<br />
x<br />
3. =∞<br />
xlim<br />
→∞ 2<br />
x<br />
4. =−∞<br />
xlim<br />
→−∞ 2<br />
Square root and natural log of infinity:<br />
5. ∞≈∞ lim x =∞<br />
x→∞<br />
Figure 55 f (x) = 1 x<br />
Since f can be made as close as we please to 0 by choosing x sufficiently large, we<br />
write<br />
1<br />
lim<br />
x→∞ x = 0<br />
and say that the limit as x approaches infinity of f is equal to 0 . Similarly, as x becomes<br />
unbounded in the negative direction, the function f (x) = 1 can be made as close as we<br />
x<br />
please to 0, and we write<br />
lim<br />
x→−∞<br />
1<br />
x = 0<br />
1<br />
Although we do not prove lim = 0 here, it can be proved using the ε-δ<br />
x→−∞ x<br />
Definition of a Limit at Infinity, and is left as an exercise in Section 1.6.<br />
The limit properties discussed in Section 1.2 hold for infinite limits. Although these<br />
properties are stated below for limits as x → ∞, they are also valid for limits as<br />
x → −∞.<br />
6. ln( ∞) ≈∞ lim lnx =∞<br />
x→∞<br />
THEOREM Properties of Limits at Infinity<br />
If k is a real number, n ≥ 2 is an integer, and the functions f and g approach real<br />
numbers as x →∞, then the following properties are true:<br />
Alternate Example<br />
Finding Limits at Infinity<br />
Following are alternate ways to solve<br />
Example 5:<br />
a. lim 4 = 4 lim 1<br />
x→−∞<br />
2<br />
x x→−∞<br />
2<br />
x<br />
= 4 lim 1 ⋅ lim 1 = 400 ⋅ ⋅ = 0<br />
x→−∞<br />
x x→−∞<br />
x<br />
• lim A = A where A is a constant<br />
x→∞<br />
• lim [kf(x)] = k lim f (x)<br />
x→∞ x→∞<br />
• lim [ f (x) ± g(x)] = lim f (x) ± lim g(x)<br />
x→∞ x→∞ x→∞<br />
[ ][ ]<br />
• lim [ f (x)g(x)] = lim f (x) lim g(x)<br />
x→∞ x→∞ x→∞<br />
f (x) lim<br />
• f (x)<br />
lim<br />
x→∞ g(x) = x→∞<br />
, provided lim g(x) = 0<br />
lim g(x) x→∞<br />
x→∞<br />
[ ] n<br />
• lim [ f<br />
x→∞ (x)]n = lim f (x)<br />
x→∞<br />
√ √<br />
n<br />
• lim f (x) = n lim f (x), where f (x) >0 if n is even<br />
x→∞<br />
x→∞<br />
b. ⎛ 10 ⎞<br />
⎜ − ⎟<br />
⎝ ⎠<br />
=− ⎛<br />
lim<br />
10 lim<br />
x→∞<br />
x→∞⎜<br />
x ⎝<br />
=−10⋅ 0=<br />
0<br />
1 ⎞<br />
⎟<br />
x ⎠<br />
EXAMPLE 5<br />
Find:<br />
(a)<br />
lim<br />
x→−∞<br />
Finding Limits at Infinity<br />
(<br />
4<br />
(b) lim − 10 )<br />
√<br />
x 2 x→∞ x<br />
(c)<br />
(<br />
lim 2 + 3 )<br />
x→∞ x<br />
⎛ 3 ⎞<br />
c. +<br />
⎝<br />
⎜<br />
⎠<br />
⎟ = + ⎛ ⎞<br />
lim 2 lim 2 3 lim 1<br />
x→∞ x x→∞ x→∞⎝<br />
⎜ x⎠<br />
⎟<br />
⎛ ⎞<br />
→∞<br />
= 2+<br />
3 lim lim 1<br />
x<br />
⎝<br />
⎜<br />
⎠<br />
⎟ = 2 + 3 ⋅ 0 = 2<br />
x→∞<br />
lim x<br />
x→∞<br />
132<br />
Chapter 1 • Limits and Continuity<br />
TE_<strong>Sullivan</strong>_Chapter01_PART II.indd 15<br />
11/01/17 9:55 am