Sullivan Microsite DigiSample

Sullivan AP˙Sullivan˙Chapter01 October 8, 2016 17:4
Section 1.3 • Continuity 103
y
c
y f (x)
(c, f (c))
x
y
1.3 Continuity
c
(c, f (c))
OBJECTIVES When you finish this section, you should be able to:
1 Determine whether a function is continuous at a number (p. 103)
2 Determine intervals on which a function is continuous (p. 106)
3 Use properties of continuity (p. 108)
4 Use the Intermediate Value Theorem (p. 110)
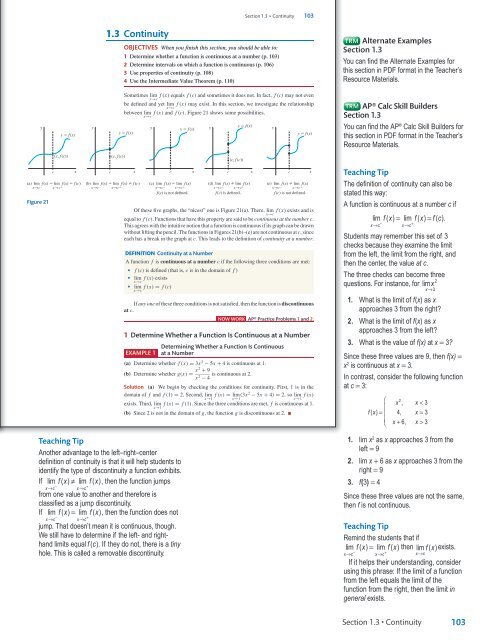
Sometimes lim f (x) equals f (c) and sometimes it does not. In fact, f (c) may not even
x→c
be defined and yet lim f (x) may exist. In this section, we investigate the relationship
x→c
between lim f (x) and f (c). Figure 21 shows some possibilities.
x→c
y f (x)
x
y
c
y f (x)
(b) lim f(x) lim f(x) f (c) (c) lim f (x) lim f(x)
(a) lim f(x) lim f(x) f (c)
x→c x→c x→c x→c x→c x→c
f(c) is not defined.
Figure 21
x
y
c
(c, f (c))
y f (x)
(d) lim f (x) lim f (x)
x→c x→c
f (c) is defined.
x
y
c
y f (x)
(e) lim f (x) lim f (x)
x→c x→c
f (c) is not defined.
Of these five graphs, the “nicest” one is Figure 21(a). There, lim
x→c
f (x) exists and is
equal to f (c). Functions that have this property are said to be continuous at the number c.
This agrees with the intuitive notion that a function is continuous if its graph can be drawn
without lifting the pencil. The functions in Figures 21(b)–(e) are not continuous at c, since
each has a break in the graph at c. This leads to the definition of continuity at a number.
DEFINITION Continuity at a Number
A function f is continuous at a number c if the following three conditions are met:
• f (c) is defined (that is, c is in the domain of f )
• lim f (x) exists
x→c
• lim f (x) = f (c)
x→c
If any one of these three conditions is not satisfied, then the function is discontinuous
at c.
NOW WORK AP® Practice Problems 1 and 2.
1 Determine Whether a Function Is Continuous at a Number
EXAMPLE 1
Teaching Tip
Another advantage to the left–right–center
definition of continuity is that it will help students to
identify the type of discontinuity a function exhibits.
If lim fx ( ) ≠ lim fx ( ), then the function jumps
− +
x→c x→c
from one value to another and therefore is
classified as a jump discontinuity.
If lim fx ( ) = lim fx ( ), then the function does not
− +
x→c x→c
jump. That doesn’t mean it is continuous, though.
We still have to determine if the left- and righthand
limits equal fc (). If they do not, there is a tiny
hole. This is called a removable discontinuity.
Determining Whether a Function Is Continuous
at a Number
(a) Determine whether f (x) = 3x 2 − 5x + 4 is continuous at 1.
(b) Determine whether g(x) = x 2 + 9
is continuous at 2.
x 2 − 4
Solution (a) We begin by checking the conditions for continuity. First, 1 is in the
domain of f and f (1) = 2. Second, lim f (x) = lim(3x 2 − 5x + 4) = 2, so lim f (x)
x→1 x→1 x→1
exists. Third, lim f (x) = f (1). Since the three conditions are met, f is continuous at 1.
x→1
(b) Since 2 is not in the domain of g, the function g is discontinuous at 2. ■
x
TRM Alternate Examples
Section 1.3
You can find the Alternate Examples for
this section in PDF format in the Teacher’s
Resource Materials.
TRM AP® Calc Skill Builders
Section 1.3
You can find the AP ® Calc Skill Builders for
this section in PDF format in the Teacher’s
Resource Materials.
Teaching Tip
The definition of continuity can also be
stated this way:
A function is continuous at a number c if
lim fx ( ) = lim fx ( ) = fc ().
− +
x→c x→c
Students may remember this set of 3
checks because they examine the limit
from the left, the limit from the right, and
then the center, the value at c.
The three checks can become three
2
questions. For instance, for lim x
x→3
1. What is the limit of f (x) as x
approaches 3 from the right?
2. What is the limit of f (x) as x
approaches 3 from the left?
3. What is the value of f(x) at x = 3?
Since these three values are 9, then f(x) =
x 2 is continuous at x = 3.
In contrast, consider the following function
at c = 3:
⎛
⎜
fx ( ) = ⎜
⎜
⎝
2
x , x < 3
4, x = 3
x+ 6, x > 3
1. lim x 2 as x approaches 3 from the
left = 9
2. lim x + 6 as x approaches 3 from the
right = 9
3. f (3) = 4
Since these three values are not the same,
then f is not continuous.
Teaching Tip
Remind the students that if
lim fx ( ) = lim fx ( ) then lim fx ( ) exists.
− +
x→c x→c
x→c
If it helps their understanding, consider
using this phrase: If the limit of a function
from the left equals the limit of the
function from the right, then the limit in
general exists.
Section 1.3 • Continuity
103
TE_Sullivan_Chapter01_PART 0.indd 32
11/01/17 9:53 am