Sullivan Microsite DigiSample

More documents

Recommendations

Info

Sullivan AP˙Sullivan˙Chapter01 October 8, 2016 17:4 Sullivan 136 Chapter 1 • Limits and Continuity Teaching Tip Students may not find it intuitive that the x lim e = 0. x→−∞ Consider showing them the following steps: lim e x x→−∞ = lim 1 Rewritethe expression. x→−∞ e − x = 0 As x approaches negative infinity,the limit approaches zero. y 4 2 (0, 1) 2 Figure 59 f (x) = e x 2 x Other Infinite Limits at Infinity We have seen that all polynomial functions have infinite limits at infinity, and some rational functions have infinite limits at infinity. Other functions also have an infinite limit at infinity. For example, consider the function f (x) = e x . The graph of the exponential function, shown in Figure 59, suggests that lim x→−∞ ex = 0 lim x→∞ ex =∞ These limits are supported by the information in Table 15. TABLE 15 x −1 −5 −10 −20 x approaches −∞ f (x) = e x 0.36788 0.00674 0.00005 −2 × 10 −9 f (x) approaches 0 x 1 5 10 20 x approaches ∞ f (x) = e x e ≈ 2.71828 148.41 22,026 4.85 × 10 8 f (x) becomes unbounded y 2 EXAMPLE 9 Find lim ln x. x→∞ Finding the Limit at Infinity of g(x) =ln x Solution Table 16 and the graph of g(x) = ln x in Figure 60 suggest that g(x) = ln x has an infinite limit at infinity. That is, 2 4 x lim ln x =∞ x→∞ 2 4 Figure 60 g(x) = ln x y 4 TABLE 16 x e 10 e 100 e 1000 e 10,000 e 100,000 → x becomes unbounded g(x) = ln x 10 100 1000 10,000 100,000 → g(x) becomes unbounded Now let’s compare the graph of f (x) = e x in Figure 59, the graph of g(x) = ln x in Figure 60, and the graph of h(x) = x 2 in Figure 61. As x becomes unbounded in the positive direction, all three of the functions increase without bound. But in Table 17, f (x) = e x approaches infinity more rapidly than h(x) = x 2 , which approaches infinity more rapidly than g(x) = ln x. ■ 24 22 2 21 2 4 x TABLE 17 x 10 50 100 1000 10,000 f (x) = e x 22,026 5.185 × 10 21 2.688 × 10 43 e 1000 e 10,000 h(x) = x 2 100 2500 = 2.5 × 10 3 1.0 × 10 4 1.0 × 10 6 1.0 × 10 8 g(x) = ln x 2.303 3.912 4.605 6.908 9.210 Figure 61 h(x) = x 2 EXAMPLE 10 Application: Decomposition of Salt in Water Salt (NaCl) dissolves in water into sodium (Na + ) ions and chloride (Cl − ) ions according to the law of uninhibited decay A(t) = A 0 e kt where A = A(t) is the amount (in kilograms) of undissolved salt present at time t (in hours), A 0 is the original amount of undissolved salt, and k is a negative number that represents the rate of dissolution. 136 Chapter 1 • Limits and Continuity TE_Sullivan_Chapter01_PART II.indd 19 11/01/17 9:56 am
Sullivan AP˙Sullivan˙Chapter01 October 8, 2016 17:4 Section 1.5 • Infinite Limits; Limits at Infinity; Asymptotes 137 NEED TO REVIEW? Solving exponential equations is discussed in Section P.5, pp. 48--49. (a) If initially there are 25 kilograms (kg) of undissolved salt and after 10 hours (h) there are 15 kg of undissolved salt remaining, how much undissolved salt is left after one day? (b) How long will it take until 1 kg of undissolved salt remains? 2 (c) Find lim A(t). t→∞ (d) Interpret the answer found in (c). Solution (a) Initially, there are 25 kg of undissolved salt, so A(0) = A 0 = 25. To find the number k in A(t) = A 0 e kt , we use the fact that at t = 10, then A(10) = 15. That is, A(10) = 15 = 25e 10k A(t) = A 0e kt , A 0 = 25; A(10) = 15 e 10k = 3 5 10k = ln 3 5 k = 1 ln 0.6 10 So, A(t) = 25e ( 1 10 ln 0.6)t . The amount of undissolved salt that remains after one day (24 h) is A(24) = 25e ( 1 10 ln 0.6)24 ≈ 7.337 kilograms (b) We want to find t so that A(t) = 25e ( 1 10 ln 0.6)t = 1 kg. Then 2 1 2 = 25e( 1 e ( 1 10 ln 0.6)t = 1 50 ( ) 1 10 ln 0.6 t = ln 1 50 t ≈ 76.582 10 ln 0.6)t common error Sometimes students think that a function cannot cross a horizontal asymptote. A function cannot cross a vertical asymptote; however, horizontal asymptotes describe the function’s behavior as x approaches positive or negative infinity. Horizontal asymptotes give no information about the function’s behavior anywhere else. A function may cross a horizontal asymptote for values of x that are not near infinity; however, as x becomes infinitely large (or small), the function will approach, but will not cross, a limiting value. That limiting value defines the horizontal asymptote. 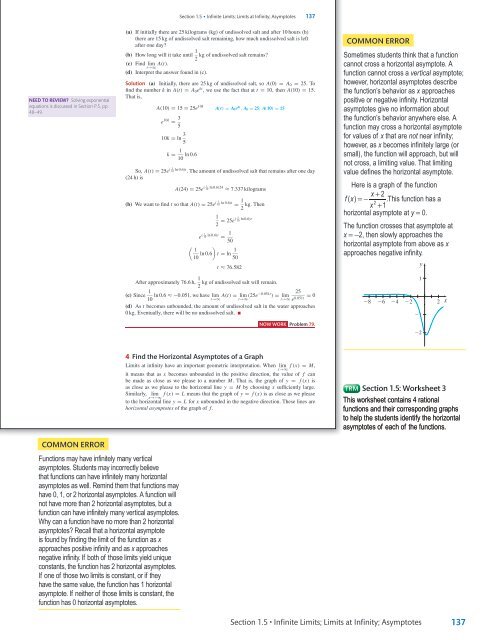Here is a graph of the function x fx=− + 2 ( ) x + 1 . This function has a 2 horizontal asymptote at y = 0. The function crosses that asymptote at x = −2, then slowly approaches the horizontal asymptote from above as x approaches negative infinity. y After approximately 76.6 h, 1 kg of undissolved salt will remain. 2 (c) Since 1 25 ln 0.6 ≈−0.051, we have lim A(t) = lim 10 t→∞ t→∞ (25e−0.051t ) = lim = 0 t→∞ e 0.051t (d) As t becomes unbounded, the amount of undissolved salt in the water approaches 0 kg. Eventually, there will be no undissolved salt. ■ 1 28 26 24 22 21 2 x NOW WORK Problem 79. 22 4 Find the Horizontal Asymptotes of a Graph Limits at infinity have an important geometric interpretation. When lim f (x) = M, x→∞ it means that as x becomes unbounded in the positive direction, the value of f can be made as close as we please to a number M. That is, the graph of y = f (x) is as close as we please to the horizontal line y = M by choosing x sufficiently large. Similarly, lim f (x) = L means that the graph of y = f (x) is as close as we please x→−∞ to the horizontal line y = L for x unbounded in the negative direction. These lines are horizontal asymptotes of the graph of f. TRM Section 1.5: Worksheet 3 This worksheet contains 4 rational functions and their corresponding graphs to help the students identify the horizontal asymptotes of each of the functions. common error Functions may have infinitely many vertical asymptotes. Students may incorrectly believe that functions can have infinitely many horizontal asymptotes as well. Remind them that functions may have 0, 1, or 2 horizontal asymptotes. A function will not have more than 2 horizontal asymptotes, but a function can have infinitely many vertical asymptotes. Why can a function have no more than 2 horizontal asymptotes? Recall that a horizontal asymptote is found by finding the limit of the function as x approaches positive infinity and as x approaches negative infinity. If both of those limits yield unique constants, the function has 2 horizontal asymptotes. If one of those two limits is constant, or if they have the same value, the function has 1 horizontal asymptote. If neither of those limits is constant, the function has 0 horizontal asymptotes. Section 1.5 • Infinite Limits; Limits at Infinity; Asymptotes 137 TE_Sullivan_Chapter01_PART II.indd 20 11/01/17 9:56 am
Page 2 and 3:
Chapter 1 Limits and Continuity Ove
Page 4 and 5:
Chapter 1: Resources TRM Teacher’
Page 6 and 7:
Sullivan AP˙Sullivan˙Chapter01 Oc
Page 8 and 9:
Page 10 and 11:
Page 12 and 13:
Page 14 and 15:
Page 16 and 17: Sullivan AP˙Sullivan˙Chapter01 Oc
Page 52 and 53: Teaching Tip Alternate Example Find
show all

Sullivan Microsite DigiSample

Create successful ePaper yourself

Delete template?

Save as template?