Sullivan Microsite DigiSample
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
<strong>Sullivan</strong> AP˙<strong>Sullivan</strong>˙Chapter01 October 8, 2016 17:4<br />
Section 1.3 • Assess Your Understanding 113<br />
⎧<br />
4 − 3x<br />
⎪⎨<br />
2 if x < 0<br />
4 if x = 0 at c = 0<br />
31. f (x) = <br />
⎪⎩ 16 − x 2<br />
if 0 < x < 4<br />
4 − x<br />
⎧√ ⎨ 4 + x if −4 ≤ x ≤ 4<br />
<br />
32. f (x) =<br />
⎩ x 2 − 3x − 4<br />
at c = 4<br />
if x > 4<br />
x − 4<br />
In Problems 33–36, each function f has a removable<br />
discontinuity at c. Define f (c) so that f is continuous at c.<br />
33. f (x) = x2 − 4<br />
x − 2 , c = 2<br />
34. f (x) = x2 + x − 12<br />
, c = 3<br />
x − 3<br />
⎧<br />
⎨ 1 + x if x < 1<br />
PAGE<br />
105 35. f (x) = 4 if x = 1 c = 1<br />
⎩<br />
2x if x > 1<br />
⎧<br />
⎨ x 2 + 5x if x < −1<br />
36. f (x) = 0 if x =−1 c =−1<br />
⎩<br />
x − 3 if x > −1<br />
In Problems 37–40, determine if each function f is<br />
continuous on the given interval. If the answer is no,<br />
state the interval, if any, on which f is continuous.<br />
PAGE<br />
107 37. f (x) = x2 − 9<br />
on the interval [−3, 3)<br />
x − 3<br />
38. f (x) = 1 + 1 on the interval [−1, 0)<br />
x<br />
39.<br />
1<br />
f (x) = on the interval [−3, 3]<br />
x 2 − 9<br />
<br />
40. f (x) = 9 − x 2 on the interval [−3, 3]<br />
In Problems 41–50, determine where each function f is continuous.<br />
First determine the domain of the function. Then support your decision<br />
using properties of continuity.<br />
41. f (x) = 2x 2 + 5x − 1 42. f (x) = x + 1 + 2x<br />
x<br />
x 2 + 5<br />
43. f (x) = (x − 1)(x 2 + x + 1) 44. f (x) = √ x(x 3 − 5)<br />
PAGE<br />
109 45. f (x) = √ x − 9<br />
46. f (x) = √ x − 4<br />
x − 3 x − 2<br />
<br />
PAGE<br />
109 47. f (x) =<br />
x 2 + 1<br />
2 − x<br />
48. f (x) =<br />
<br />
4<br />
x 2 − 1<br />
49. f (x) = (2x 2 + 5x − 3) 2/3 50. f (x) = (x + 2) 1/2<br />
In Problems 51–56, use the function<br />
⎧ √<br />
15 − 3x if x < 2<br />
⎪⎨ √<br />
5 if x = 2<br />
f (x) =<br />
9 − x ⎪⎩<br />
2 if 2 < x < 3<br />
x − 2 if 3 ≤ x<br />
51. Is f continuous at 0? Why or why not?<br />
52. Is f continuous at 4? Why or why not?<br />
PAGE<br />
105 53. Is f continuous at 3? Why or why not?<br />
49. Continuous on the set of all real numbers.<br />
50. Continuous on { xx | ≥− 2} .<br />
51. f is continuous at 0 because lim fx ( ) = f (0).<br />
x→0<br />
52. f is discontinuous at 4 because lim fx ( )<br />
x→4<br />
does not exist.<br />
53. f is discontinuous at 3 because lim fx ( ) does<br />
x → 3<br />
not exist.<br />
54. f is discontinuous at 2 because lim fx ( ) does<br />
x→2<br />
not exist.<br />
55. f is continuous at 1 because lim fx ( ) = f (1).<br />
x→1<br />
56. f is continuous at 2.5 because<br />
lim fx ( ) = f (2.5).<br />
x→2.5<br />
54. Is f continuous at 2? Why or why not?<br />
55. Is f continuous at 1? Why or why not?<br />
56. Is f continuous at 2.5? Why or why not?<br />
In Problems 57 and 58:<br />
(a) Use technology to graph f using a suitable scale on each axis.<br />
(b) Based on the graph from (a), determine where f is continuous.<br />
(c) Use the definition of continuity to determine where f is continuous.<br />
(d) What advice would you give a fellow student about using<br />
technology to determine where a function is continuous?<br />
57. f (x) = x3 − 8<br />
x − 2<br />
58. f (x) = x2 − 3x + 2<br />
3x − 6<br />
In Problems 59–64, use the Intermediate Value Theorem to determine<br />
which of the functions must have zeros in the given interval. Indicate<br />
those for which the theorem gives no information. Do not attempt to<br />
locate the zeros.<br />
PAGE<br />
111 59. f (x) = x 3 − 3x on [−2, 2]<br />
60. f (x) = x 4 − 1on[−2, 2]<br />
61.<br />
x<br />
f (x) = − 1 on [10, 20]<br />
(x + 1) 2<br />
62. f (x) = x 3 − 2x 2 − x + 2 on [3, 4]<br />
63. f (x) = x3 − 1<br />
on [0, 2]<br />
x − 1<br />
64. f (x) = x2 + 3x + 2<br />
x 2 on [−3, 0]<br />
− 1<br />
In Problems 65–72, verify that each function has a zero in the indicated<br />
interval. Then use the Intermediate Value Theorem to approximate the<br />
zero correct to three decimal places by repeatedly subdividing the<br />
interval containing the zero into 10 subintervals.<br />
PAGE<br />
112 65. f (x) = x 3 + 3x − 5; interval: [1, 2]<br />
66. f (x) = x 3 − 4x + 2; interval: [1, 2]<br />
67. f (x) = 2x 3 + 3x 2 + 4x − 1; interval: [0, 1]<br />
68. f (x) = x 3 − x 2 − 2x + 1; interval: [0, 1]<br />
69. f (x) = x 3 − 6x − 12; interval: [3, 4]<br />
70. f (x) = 3x 3 + 5x − 40; interval: [2, 3]<br />
71. f (x) = x 4 − 2x 3 + 21x − 23; interval: [1, 2]<br />
72. f (x) = x 4 − x 3 + x − 2; interval: [1, 2]<br />
In Problems 73 and 74,<br />
(a) Use the Intermediate Value Theorem to show that f has a zero in<br />
the given interval.<br />
(b) Use technology to find the zero rounded to three decimal places.<br />
<br />
73. f (x) = x 2 + 4x − 2 in [0, 1]<br />
74. f (x) = x 3 − x + 2in[−2, 0]<br />
Applications and Extensions<br />
Heaviside Functions In Problems 75 and 76, determine whether the<br />
given Heaviside function is continuous at c.<br />
0 if t < 1<br />
75. u 1(t) =<br />
1 if t ≥ 1<br />
c = 1<br />
0 if t < 3<br />
76. u 3(t) =<br />
1 if t ≥ 3<br />
c = 3<br />
57. (a) <br />
y<br />
18<br />
14<br />
10<br />
6<br />
2<br />
3 2 1 1 2 3 x<br />
(b) Based on the graph, it appears that the<br />
function is continuous for all real numbers.<br />
(c) f is actually continuous at all real numbers<br />
except x = 2.<br />
(d) Answers will vary. Sample answer:<br />
Conclusions drawn from graphing technology<br />
should always be confirmed using basic<br />
analysis.<br />
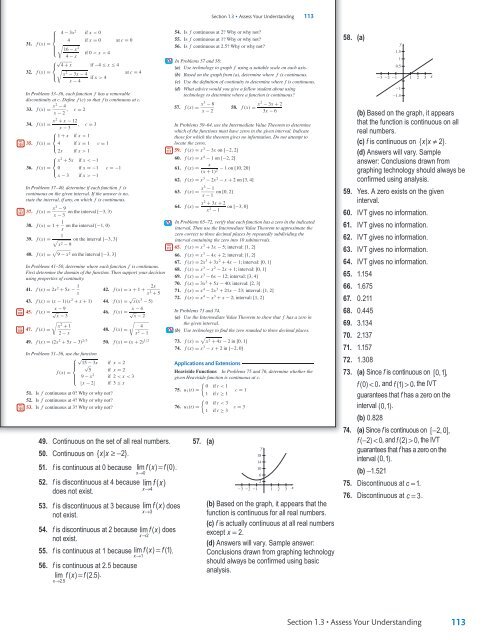
58. (a)<br />
23 22 21<br />
y<br />
1.5<br />
1<br />
0.5<br />
21<br />
21.5<br />
1 2 3<br />
(b) Based on the graph, it appears<br />
that the function is continuous on all<br />
real numbers.<br />
(c) f is continuous on { xx | ≠ 2}.<br />
(d) Answers will vary. Sample<br />
answer: Conclusions drawn from<br />
graphing technology should always be<br />
confirmed using analysis.<br />
59. Yes. A zero exists on the given<br />
interval.<br />
60. IVT gives no information.<br />
61. IVT gives no information.<br />
62. IVT gives no information.<br />
63. IVT gives no information.<br />
64. IVT gives no information.<br />
65. 1.154<br />
66. 1.675<br />
67. 0.211<br />
68. 0.445<br />
69. 3.134<br />
70. 2.137<br />
71. 1.157<br />
72. 1.308<br />
73. (a) Since f is continuous on [0,1],<br />
f (0) < 0, and f (1) > 0, the IVT<br />
guarantees that f has a zero on the<br />
interval (0,1).<br />
(b) 0.828<br />
74. (a) Since f is continuous on [ −2,0],<br />
f ( − 2) < 0, and f (2) > 0, the IVT<br />
guarantees that f has a zero on the<br />
interval (0,1).<br />
(b) −1.521<br />
75. Discontinuous at c = 1.<br />
76. Discontinuous at c = 3.<br />
x<br />
Section 1.3 • Assess Your Understanding<br />
113<br />
TE_<strong>Sullivan</strong>_Chapter01_PART I.indd 10<br />
11/01/17 9:58 am