Sullivan Microsite DigiSample

Sullivan AP˙Sullivan˙Chapter01 October 8, 2016 17:4
Section 1.3 • Continuity 107
22 21
y
2
1
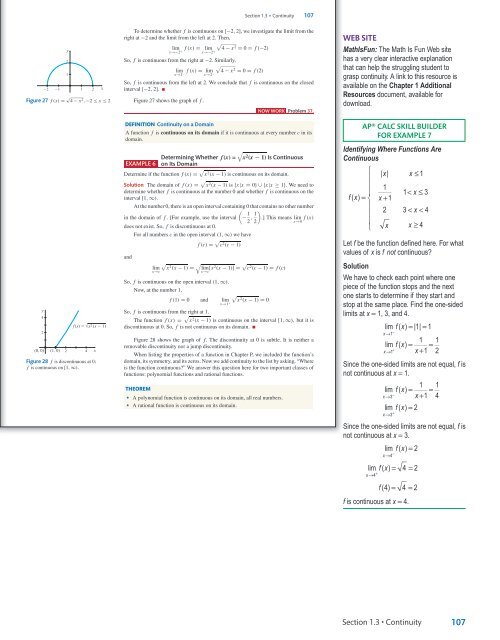
Figure 27 f (x) = √ 4 − x 2 , −2 ≤ x ≤ 2
y
4
2
(0, 0)
(1, 0)
1
2 4
2
x
x
f (x) x 2 (x 1)
Figure 28 f is discontinuous at 0;
f is continuous on [1, ∞).
To determine whether f is continuous on [−2, 2], we investigate the limit from the
right at −2 and the limit from the left at 2. Then,
√
lim f (x) = lim
x→−2 +
4 − x
2
= 0 = f (−2)
x→−2 +
So, f is continuous from the right at −2. Similarly,
lim
x→2 −
√
f (x) = lim 4 − x 2
= 0 = f (2)
x→2 −
So, f is continuous from the left at 2. We conclude that f is continuous on the closed
interval [−2, 2]. ■
Figure 27 shows the graph of f .
DEFINITION Continuity on a Domain
NOW WORK Problem 37.
A function f is continuous on its domain if it is continuous at every number c in its
domain.
EXAMPLE 6
√
Determining Whether f(x) = x 2 (x − 1) Is Continuous
on Its Domain
Determine if the function f (x) = √ x 2 (x − 1) is continuous on its domain.
Solution The domain of f (x) = √ x 2 (x − 1) is {x|x = 0} ∪{x|x ≥ 1}. We need to
determine whether f is continuous at the number 0 and whether f is continuous on the
interval [1, ∞).
At the number 0, there is an open interval containing 0 that contains no other number
( )
in the domain of f . [For example, use the interval − 1 2 , 1 2
.] This means lim f (x)
x→0
does not exist. So, f is discontinuous at 0.
and
For all numbers c in the open interval (1, ∞) we have
f (c) = √ c 2 (c − 1)
√
lim x 2
(x − 1) = √ lim[x 2 (x − 1)] = √ c 2 (c − 1) = f (c)
x→c
x→c
So, f is continuous on the open interval (1, ∞).
Now, at the number 1,
So, f is continuous from the right at 1.
f (1) = 0 and lim
x→1 + √
x
2
(x − 1) = 0
The function f (x) = √ x 2 (x − 1) is continuous on the interval [1, ∞), but it is
discontinuous at 0. So, f is not continuous on its domain. ■
Figure 28 shows the graph of f. The discontinuity at 0 is subtle. It is neither a
removable discontinuity nor a jump discontinuity.
When listing the properties of a function in Chapter P, we included the function’s
domain, its symmetry, and its zeros. Now we add continuity to the list by asking, “Where
is the function continuous?” We answer this question here for two important classes of
functions: polynomial functions and rational functions.
THEOREM
• A polynomial function is continuous on its domain, all real numbers.
• A rational function is continuous on its domain.
WEB SITE
MathIsFun: The Math Is Fun Web site
has a very clear interactive explanation
that can help the struggling student to
grasp continuity. A link to this resource is
available on the Chapter 1 Additional
Resources document, available for
download.
AP® Calc Skill Builder
for Example 7
Identifying Where Functions Are
Continuous
⎧
⎪
⎪
⎪
fx ( ) = ⎨
⎪
⎪
⎪
⎩
| x| x ≤ 1
1
1< x ≤3
x + 1
2 3< x < 4
x
x ≥ 4
Let f be the function defined here. For what
values of x is f not continuous?
Solution
We have to check each point where one
piece of the function stops and the next
one starts to determine if they start and
stop at the same place. Find the one-sided
limits at x = 1, 3, and 4.
lim fx ( ) = |1| = 1
x→1
− 1 1
lim fx ( ) = =
x→ 1
+ x + 1 2
Since the one-sided limits are not equal, f is
not continuous at x = 1.
1 1
lim fx ( ) = =
x→3
− x + 1 4
lim fx ( ) = 2
x→ 3
+
Since the one-sided limits are not equal, f is
not continuous at x = 3.
lim fx ( ) = 2
x→4
−
lim fx ( ) = 4 = 2
→ + f (4) = 4 = 2
x 4
f is continuous at x = 4.
Section 1.3 • Continuity 107
TE_Sullivan_Chapter01_PART I.indd 4
11/01/17 9:58 am